If $A$ is a thermodynamic variable (ex:Pressure, volume, entropy). then If $\oint{A}=0$, then does it imply that $A$ has to be a state function? I'm trying to prove that Entropy is a state function. In a reversible process, $\oint{\frac{dQ}{T}}=0$ (taking it for granted). From this can I deduce that entropy has to be a state function?
$\begingroup$
$\endgroup$
asked Apr 2, 2020 at 15:50
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
0
If $\oint \mathrm dA = 0$, then $A$ needs to be a state function which implies it is path independent.
Why?
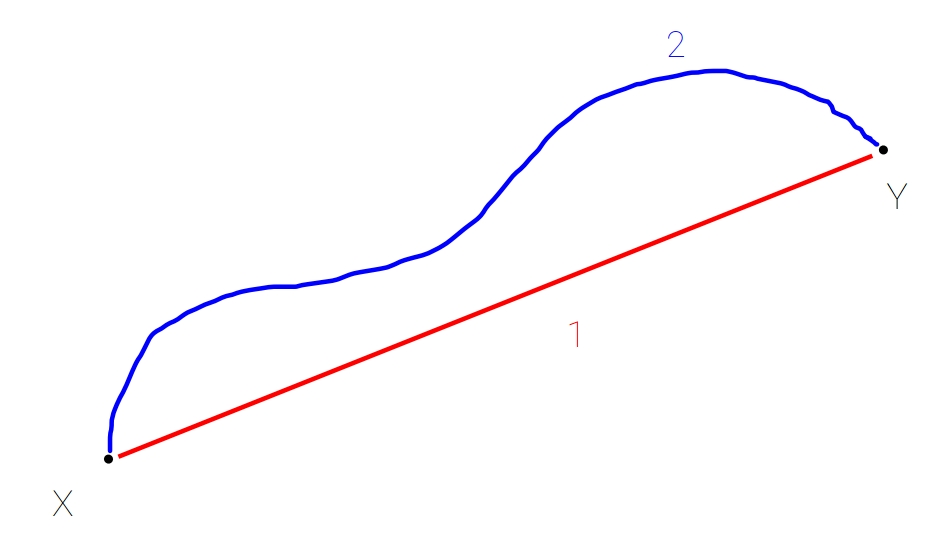
In the above image, if we go from $X$ to $Y$ by path 1 and return from $Y$ to $X$ by path 2, then $\oint \mathrm dA = 0$ since we started from $X$ and returned back to $X$. Therefore,
$$\oint \mathrm dA =\left( \int _X ^Y \mathrm dA \right)_{\text{path 1}} + \left( \int _Y ^X \mathrm dA \right)_{\text{path 2}}=0$$
So,
\begin{align} \left( \int _X ^Y \mathrm dA \right)_{\text{path 1}}&=-\left( \int _Y ^X \mathrm dA \right)_{\text{path 2}}\\ \left( \int _X ^Y \mathrm dA \right)_{\text{path 1}}&=\left( \int _X ^Y \mathrm dA \right)_{\text{path 2}} \end{align}
Thus no matter what path you take, $\int \mathrm d A $ will always be the same. The above procedure can be repeated with any two arbitrary paths and the value of $\int \mathrm d A$ will always come out to be the same.
