Point with greatest acceleration - constantly accelerated rolling wheel

Question

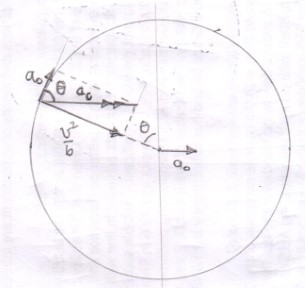
This is a problem from Analytical Mechanics, Fowles & Cassiday. I am not sure if the solution in the solution manual is correct, and I am not sure if my solution is correct.

A wheel of radius $b$ rolls along the ground with constant forward acceleration $a_0$. Show that, at any given instant, the magnitude of the acceleration of any point on the wheel is $$a_c=\sqrt{a^2_0+\frac{v^4}{b^2}}$$ relative to the center of the wheel and is also $$a_g=a_0\sqrt{2+2\cos(\theta)+\frac{v^4}{a^2_0b^2}-\frac{2v^2\sin(\theta)}{a_0b}}$$ relative to the ground. Let's call what's under the square root $A$ (in the expression for $a_g$). Here $v$ is the instantaneous forward speed, and $\theta$ defines the location of the point on the wheel, measured forward from the highest point. Which point has the greatest acceleration relative to the ground?

Showing that those magnitudes are correct is fine. For the last question, the solution manual simply states that the acceleration has its maximum at the top of the wheel. It then jots down the following calculation: $$-2\sin(\theta)-\frac{2v^2}{a_0b}\cos(\theta)=0, \\ \theta=\arctan(-\frac{v^2}{a_0b}).$$ It does not offer any further explanation. It's already problematic to me that this $\theta$ is zero (since they say it's supposed to be a maximum at the top of the wheel) if and only if $v=0$ at the top of the wheel, which I don't think is the case since the velocity vector for $\theta=0$ is $v$ in the $x$-direction (assuming that the initial velocity is zero). The last calculation of course arises as setting the derivative of $A$ with respect to $\theta$ to zero, where it is assumed that $v$ does not depend on $\theta$. Implicitly, it seems that they use the chain rule $$\frac{da_g}{dt}=\frac{da_g}{d\theta}\frac{d\theta}{dt}$$ to use the derivative with respect to $\theta$ instead of time. As $\frac{d\theta}{dt}=\frac{v}{b}$, the derivative with respect to $t$ is zero if and only if the derivative with respect to $\theta$ is zero. This is of course fine, but taking the derivative of $A$ with respect to time yields $-6\sin(\theta)+\frac{2v^2}{a_0b}(2-\cos(\theta))=0$, which does not give the same result for $\theta$ as what they say (in fact, I cannot find a closed-form expression for $\theta$). Obviously, you get the same result when you take the derivative of $A$ with respect to $\theta$ and when you do not assume that $\frac{dv}{d\theta}\neq0$. In fact, using $a_0=\frac{dv}{dt}=\frac{dv}{d\theta}\frac{d\theta}{dt}$ yields $\frac{dv}{d\theta}=\frac{ba_0}{v}$.

Anyhow, I am unsure of what's right (and why), so some help would be appreciated. Like I said, I personally find that the angle where the acceleration is at its maximum is defined by $$\frac{\sin(\theta)}{2-\cos(\theta)}=\frac{v^2}{3a_0b}.$$

Answer 1

I think you are correct in pointing out that the acceleration relative to the ground is not a maximum at the top.

Using your notation $\vec{a_{\rm g}} = \vec{a_{\rm c}} + \vec{a_{\rm 0}}$ and you are asked to maximise the magnitude of $\vec{a_{\rm g}}$.

The point to note is that the magnitude of $\vec{a_{\rm c}}$ is constant and all that happens as the position on the wheel changes is that the direction of $\vec{a_{\rm c}}$ changes.

The maximum of $\vec{a_{\rm g}}$ will occur when both $\vec{a_{\rm c}}$ and $\vec{a_{\rm 0}}$, point in the same direction as shown in the diagram below.

From the diagram, with angle $\theta$ negative, you can see where the relationship $\theta=\arctan\left (-\dfrac{v^2}{a_0b}\right )$ comes from, without differentiation.

Answer 2

$a_g$ is the acceleration at a given time, so it makes no sense to differentiate against time, since you want the maximum depending on theta you differentiate just for theta. No chain rule involved, at a different time you will have a different $v$, so the max will be at a different point.