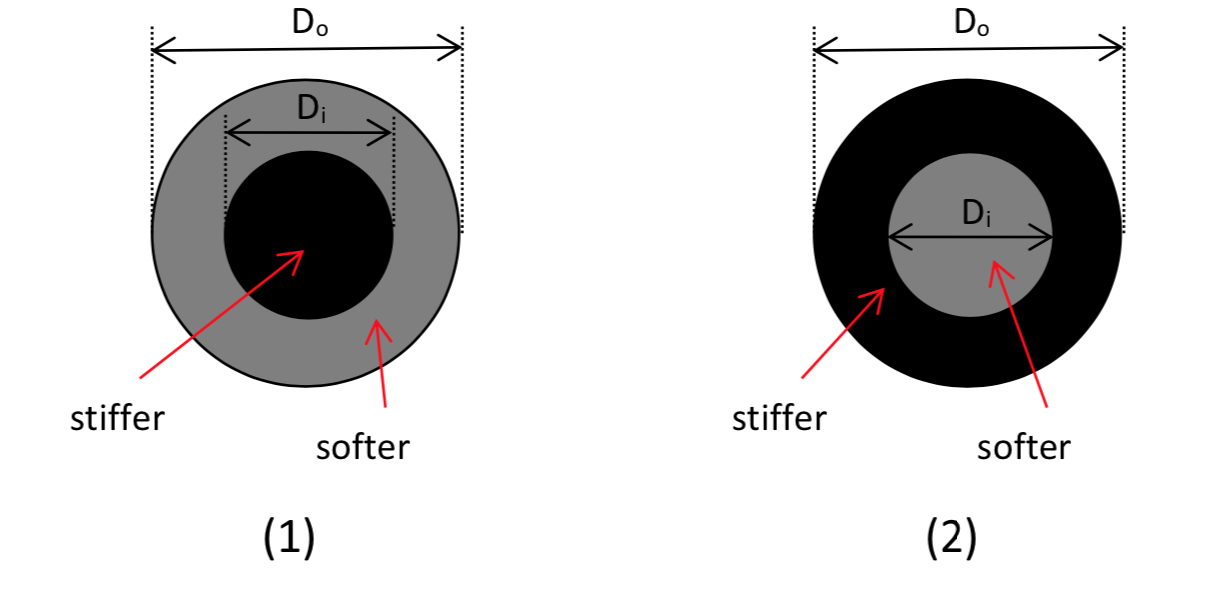
Which one deforms more when subjected to the same torque? I am having trouble thinking about this mathematically and with equations. Don't know what to do...
-
$\begingroup$ What are the boundary conditions and loads for this problem? $\endgroup$– nicoguaroCommented May 14, 2020 at 16:42
-
$\begingroup$ Maybe are you referring to a rod that is long compared with $D_0$ with a torque applied in the ends? $\endgroup$– nicoguaroCommented May 14, 2020 at 16:53
1 Answer
I will assume that you are talking about the twist of a slender bar.
In that case, you can consider that the torsional stiffness is written as
$$ \kappa_0 = J_0 G_0 + J_1 G_1 = \frac{\pi G_0 (r_1^4 -r_0^4)}{2} + \frac{\pi r_0^4\, G_1}{2}\, , $$
for one case, and
$$ \kappa_1 = J_0 G_1 + J_1 G_0 = \frac{\pi G_1 (r_1^4 -r_0^4)}{2} + \frac{\pi r_0^4\, G_0}{2}\, , $$
in the other.
Now, let us compare both of these
$$\kappa_r = \frac{\kappa_1}{\kappa_0} = \frac{G_1 r_1^4 - r_0^4 G_1 + G_0 r_0^4} {G_0 r_1^4 + r_0^4 G_1 -G_0 r_0^4}\, ,$$
and if we take the following limits,
\begin{align} \lim_{G_1 \rightarrow \infty} = \frac{r_1^4 - r_0^4}{r_0^4}\, ,\\ \lim_{G_1 \rightarrow 0} = \frac{r_0^4}{r_1^4 - r_0^4}\, , \end{align}
we see that the stiffness is higher when the external part is stiffer.