Would it be harder to lift a dumb bell in free falling lift, where the dumbbell is falling with us or would it be easier?
$\begingroup$
$\endgroup$
Add a comment
|
3 Answers
$\begingroup$
$\endgroup$
0
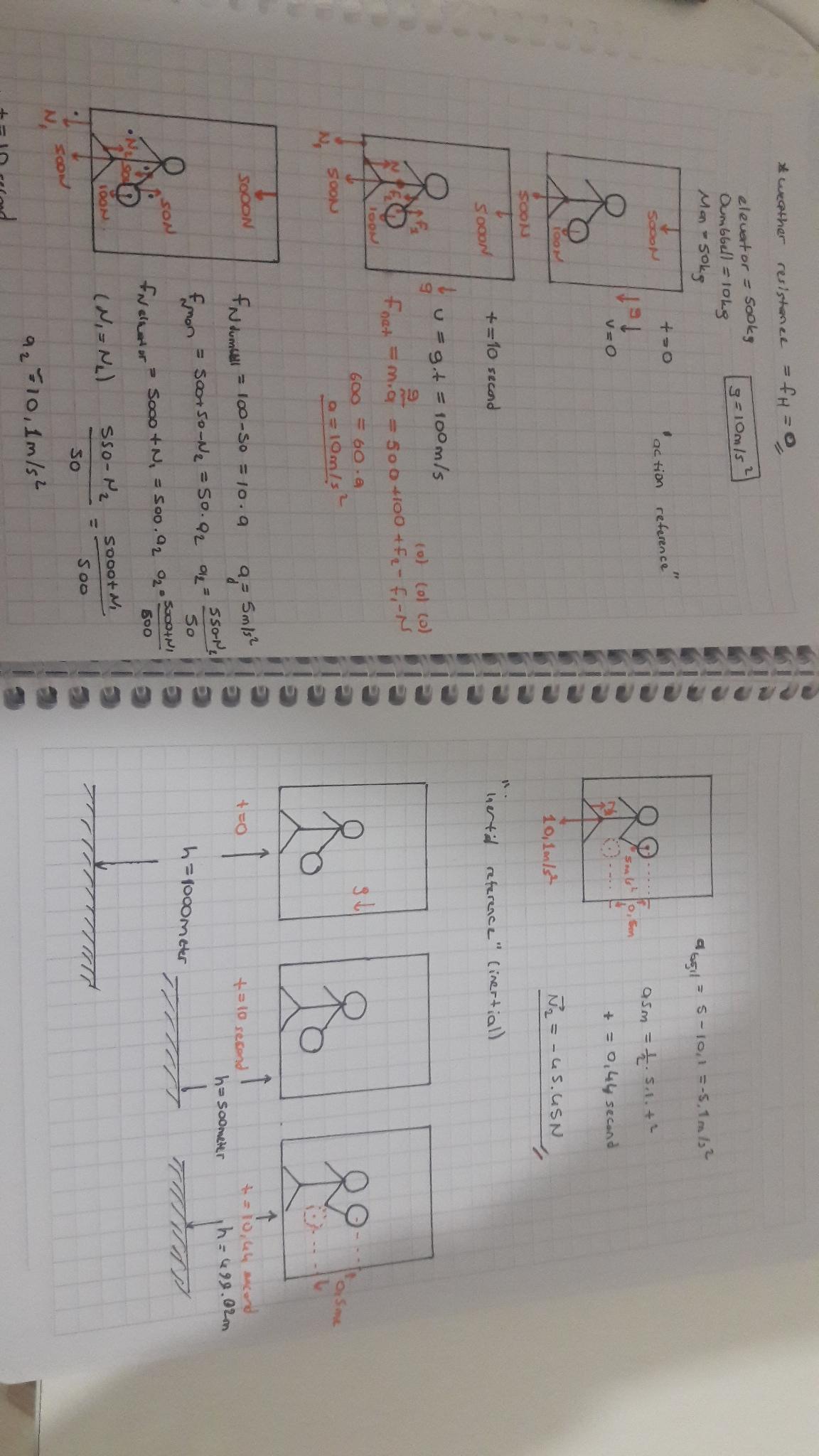
In free fall both you and the dumbbell would be weightless. If you were to release it it would “float” in the air along side you. It would be as if you were in outer space far from any gravitational field. Since the dumbbell is weightless “lifting” has no meaning.
Bottom line: it would require no force to move the dumbbell at constant velocity. It would require a force to accelerate it per Newton’s second law $F=ma$.
Hope this helps
$\begingroup$
$\endgroup$
10
It would be easier to cause a dumbbell to accelerate w.r.t. the person if the dumbbell was in free fall along with the person, such as in a free falling lift containing the person and the dumbbell. If $a$, $g$, $m$ and $F$ denote the acceleration of the dumbbell w.r.t. the person, the constant acceleration due to gravity, the mass of the dumbbell and the force applied by the person on the dumbbell in the direction opposite to that of gravity, respectively, then the equation of motion in the vertically upward direction will be $ma = F + m \cdot -g - m \cdot -g$ where we recognize the last term as the pseudo or non-inertial force due to the use of the non-inertial reference frame attached to the person (who is assumed to be accelerating in the direction of gravity with acceleration $g$). This implies that $ma = F$ which is in contrast to a person who is not free falling, attempting to accelerate the dumbbell by applying the force $\tilde{F}$ while stationary w.r.t. the ground with the equation of motion $ma = \tilde{F} - mg$. The answer then follows on observing that $F < \tilde{F}$. This is similar to the situation of apparent weightlessness in a steadily orbiting space station.
answered Mar 4, 2020 at 5:58
-
$\begingroup$ I am sorry, but this description is making me confused. So in the end, does it need more or less visible effort to lift a dumb bell in free falling. $\endgroup$ Commented Mar 4, 2020 at 6:16
-
1$\begingroup$ It requires less apparent effort to 'lift' the dumbbell if it is free falling along with the person lifting it. $\endgroup$ Commented Mar 4, 2020 at 6:17
-
$\begingroup$ How much less? Like half or only point difference? $\endgroup$ Commented Mar 4, 2020 at 6:23
-
$\begingroup$ The difference in the efforts can be measured in terms of the magnitude of the force required to cause the same relative acceleration of the dumbbell. As calculated in the answer, the difference is $\tilde{F} - F = mg$. So, the direct answer is, less by the weight of the dumbbell on the ground. $\endgroup$ Commented Mar 4, 2020 at 6:25
-
1$\begingroup$ I think you would benefit from the answer and discussion to this question. $\endgroup$ Commented Mar 4, 2020 at 7:05
$\begingroup$
$\endgroup$
Free fall is falling with the acceleration of gravity. Therefore, the person inside the elevator does not feel his weight while holding the dumbbell (unless a force is applied). However, if it exerts a force, the dumbbell acceleration decreases. So he feels as if he is up in the elevator. However, according to the external viewer, the elevator and dumbbell move downward. I threw you these detailed force analyses. I examined it in the action and inactive reference frames.
