Hubble tension and density parameter

Question

Hi I was reading about the Hubble tension. I know that the Planck Collaboration gives an actual value for the Hubble parameter as $H_{0}=64.3 kms^{-1}Mpc^{-1}$ and the group that measures $H_{0}$ with Type Ia Supernovae gives $H_{0}=74.03 kms^{-1}Mpc^{-1}$.

Since there is a tension for the measured values of $H_{0}$ and since in the standard cosmological model $H_{0}$ is given by

$$H_{0}=\int_{0}^{Z_{last}}\frac{dz}{\sqrt{\Omega_{m}(1+z)^3+\Omega_{r}(1+z)^4+\Omega_{\Lambda}}}$$ where $Z_{last}\approx 1100$ is the redshift where radiation decouples from matter.

Planck Colaboration gives $\Omega_{m}=0.315\pm 0.007$ $\Omega_{\Lambda}=0.6847\pm 0.0073$, $\Omega_{r}= 9.28656\times 10^{-5}$.

And I can't find the values measured with supernovae, but my question is there is a tension between the values of $\Omega$ with CMB and the values with supernovae?

Or someone knows where are the values of $\Omega$ measured with supernovae?

Answer 1

Being model-independent, other than assuming $\Omega_k=0$ (zero curvature of the universe), supernovae measurement of $H_0$ does not need the input of $\Omega_m$, $\Omega_r$, or $\Omega_{\Lambda}$.

Supernovae methods directly measure $H_0$ $$ H_0 = \frac{\dot{a}(t)}{a(t)}|_{t=t_0} $$ via (see here)

• Red shift to infer the speed of the receding supernovae-hosting galaxies
• Cosmic ladders like Cepheids to infer distance

On the other hand, $H_0$ from Planck is model dependent, since it needs the input of $\Omega_m$, $\Omega_r$, and $\Omega_{\Lambda}$ at red shift $z\sim 1100$ to derive the Hubble parameter at red shift $z=0$ by integrating the Friedmann equation all the way from $z\sim 1100$ to $z=0$ as shown above in the OP.

Generally speaking, direct measurements are more trustworthy. Supernovae measurements are actually corroborated by other direct measurement methods (see here, here, here, and here). Barring systemic bias, the culprit and the root cause of "Hubble tension" is most likely the standard cosmology model $\Lambda$CDM (see Is standard cosmology $\Lambda$CDM currently in deep trouble?). Either

• $\Omega_m$, $\Omega_r$, or $\Omega_{\Lambda}$ is possibly off, they are not what we think they are. To quote Donald (another Donald) "there are known knowns...there are known unknowns...there are also unknown unknowns". One might consider adding more "dark" elements into the mixture like the Kafkaesque scenario of "dark interaction between dark matter and dark energy" (I am not making this up. See here).
• or there is something wrong with Friedmann equation and for that matter Einstein's gravity equations.

The Jury is still out. And it's an opportune time to be a cosmologist now, since historically "cloud over physics" is a harbinger of phenomenal science breakthroughs.

__

Added note:

There is an interesting Youtube video on the "The crisis in cosmology" (see here).

Answer 2

Supernovae observations at low redshifts can yield a value for $H_0$ that is insensitive to other cosmological parameters and independent of other methods. That isn't the case for the other main cosmological parameters, where joint constraints from multiple methods (including the CMB, baryon acoustic oscillations) are needed to get them with any precision.

Some very recent results can be found in the summary provided on behalf of the Dark Energy Survey (DES) by Abbott et al. (2019), which also contains many references to other sources of data.

"Supernova cosmology" works by comparing the apparent magnitude of Type Ia supernovae, which depends on their luminosity distance, with their redshift. The former depends on the present-day value of the Hubble parameter, but also on its history with redshift, and hence has some sensitivity to the cosmological parameters.

The important equations are that the luminosity distance is given by $$d_L = c(1+z)\int_0^{z} \frac{dz'}{H(z')},$$ where the Hubble parameter depends on redshift as $$ H(z) = H_0 \left[ \Omega_M (1+z)^3 + \Omega_\Lambda (1+z)^{-3(1+w)}\right]^{-1/3}\ .$$

In the latter equation, $w$ is the equation of state parameter for dark energy. If we are dealing with a cosmological constant then $w=-1$. The above equations assume a flat universe and the results are sensitive to both $\Omega_M$ and $\Omega_\Lambda$.

More general formulations are also possible where flatness is not assumed and what is found is a degeneracy between $\Omega_M$ and $\Omega_\Lambda$. i.e. Supernovae observations alone do not yield both parameters simultaneously, they are most sensitive to the combination $\Omega_\Lambda - \Omega_M$. A typical plot of results is shown below (this one from the Supernova Cosmology Project, but all other surveys have produced plots with similar morphology).

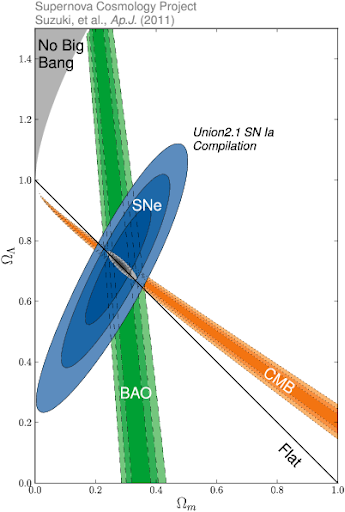
These plots, sometimes called "concordance plots" show that the cosmological parameters are actually given by simultaneous constraints by multiple cosmological observations. So whilst the CMB results alone strongly favour a flat universe, the supernova observations suggest that in order to be flat there must be some form of dark energy.

To get to your question. Observations of supernovae do not independently yield any cosmological parameters (with any precision) apart from $H_0$. The constraint on $H_0$ is obtained from the supernovae that are observed at low redshift, that sample the expansion that is in the local universe, but which are far enough away to be in the "Hubble flow". At low redshifts ($z<0.1$) then $H(z) \simeq H_0$, with only small corrections that depend on the cosmological parameters.

Determining the other cosmological parameters depends on combining the supernova results with other constraints, so it isn't possible to talk about tensions between results from supernovae Vs results with other methods.