Does solar frame dragging induce a higher centrifugal force to Mercury so its orbit is precessing as well as being more outwards than it should be if there wasn't solar frame dragging?
$\begingroup$
$\endgroup$
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
5
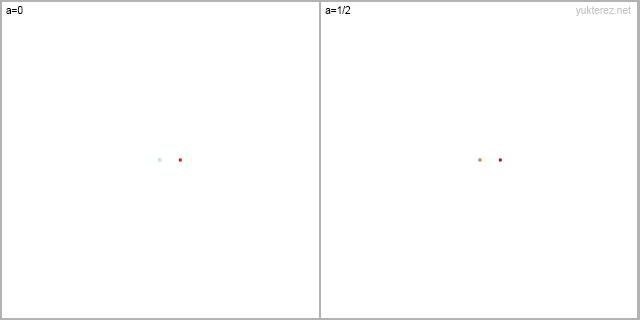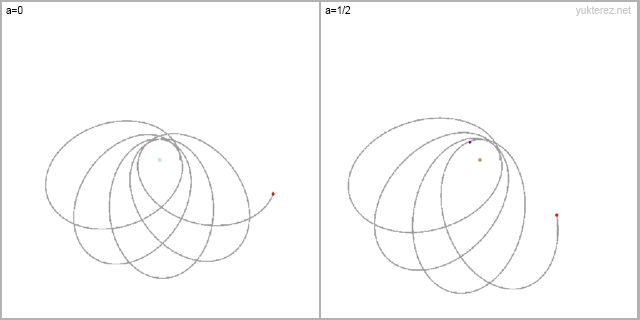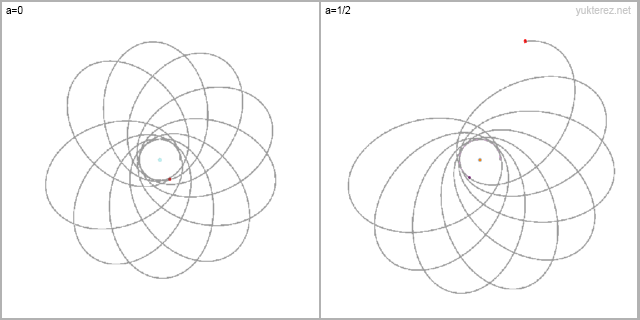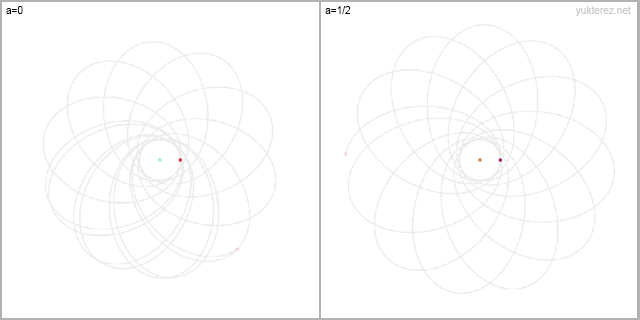
Frame dragging is not responsible for Mercury's precession, that can be explained with a combination of Newton's planetary tug and the Schwarzschild metric. Frame dragging has the effect that the a prograde circular orbit at a given radius need smaller velocity than without frame dragging, and a larger velocity for a retrograde orbit. The perihelion is also shifted a bit, but that is not significant for Mercury. In the strong field around a black hole the effect is more visible: on the left the orbit is around a Schwarzschild black hole and therefore without frame dragging, and on the right around a Kerr black hole. Both start with the same initial conditions at x=20 with v=5/12/√2:
Since they both start with the same initial velocity and at the same distance, but the one on the right has frame dragging for support, its farthest radius is farther than the one without frame dragging.
-
$\begingroup$ So precession is same no matter of orbit being prograde or retrograde?Thanks for answering this question. $\endgroup$– jbradvi9Commented Jan 18, 2020 at 14:41
-
$\begingroup$ For Mercury the frame dragging is neglible, so practically yes. In the black hole example above the perihelion shift is even less with frame dragging, since when we start at the same periapsis, the apoapsis of the test particle is farther away from the center of mass. Therefore the Schwarzschild particle's perihelion on the left has shifted by ≈180° at the 6th revolution, but the Kerr particle's on the right visibly less. $\endgroup$– YukterezCommented Jan 18, 2020 at 15:10
-
$\begingroup$ It also depends on how the same initial conditions are defined in curved spacetime, since the metric changes. Here I used the cartesian embedding of the Boyer Lindquist coordinates for the position and the local velocity relative to a ZAMO (purple dot in circular motion on the right), if you use the same r-coordinate instead of the same x the periapsis would be a little farther for the Kerr particle, and if you define its initial velocity relative to a fixed observer (not possible below the ergosphere) the initial velocity would be lower for a propgrade particle, and the apoapsis closer $\endgroup$– YukterezCommented Jan 18, 2020 at 16:27
-
1$\begingroup$ Am I right.....When a body starts falling into a black hole radially it will after a while gain angular velocity so the question is: does space-time along its curvature have also curl? $\endgroup$– jbradvi9Commented Jan 18, 2020 at 22:52
-
1$\begingroup$ Angular momentum is conserved, so if you start with no angular momentum you keep zero angular momentum all the way down. In the frame of a far away observer you will gain angular velocity though, but since your angular velocity relative to the local corotating obervers (the ZAMOs) stays zero, so does your angular momentum. The curl is discussed on page 10 of arxiv.org/pdf/gr-qc/0411060.pdf#page=10 $\endgroup$– YukterezCommented Jan 18, 2020 at 23:01