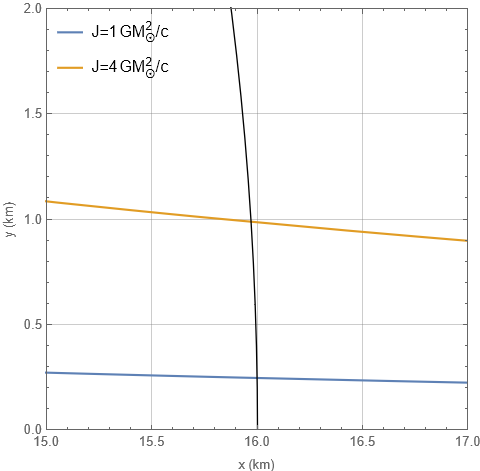
PSR J1748−2446ad is the fastest known pulsar rotating at 716 times per second. This neutron star is estimated to have a mass of less than two solar masses and a radius of less than 16km. The linear velocity at the equator is approximately 24% the speed of light or over 70,000 km/second. As such, this object would exhibit an extreme degree of frame dragging.
How would the trajectory of an incoming electrically neutral particle (say a neutron) initially approaching on the equatorial plane and perpendicular to the rotational axis be modified due to the frame dragging effect? Would the frame dragging effect be so great that the particle would spiral down revolving around the neutron star multiple times before impacting the surface, or would its impact point be shifted off a pathway perpendicular to the rotational axis by a far smaller amount?
Please assume a neutron star mass of two solar masses, a radius of 16km, an initial approach velocity of the particle toward the neutron of zero m/s (such that its relative motion would be initiated due to gravitational attraction), and an initial distance between the neutron and the surface of the neutron star of any arbitrary amount you deem reasonable (perhaps 100km or 1000km?) to demonstrate the magnitude of the frame dragging effect. Also, given these initial conditions, would the frame dragging affect the neutron path taking it off the equatorial plane or would it be constrained to that plane? It would be great if an equation of the actual path could be provided, or at least an equation giving the vector force on the neutron as a function of time, such that this (presumably) differential equation could subsequently be solved either exactly, or by numerical methods, by myself or others to be subsequently posted.
I understand frame dragging qualitatively but not quantitatively, and the math is beyond me. If a frame dragging expert could address this specific extreme though real example, I think it could help the community gain a better understanding of this phenomenon. Thanks in advance for responses and insights!