Relation between linear and angular velocity

Question

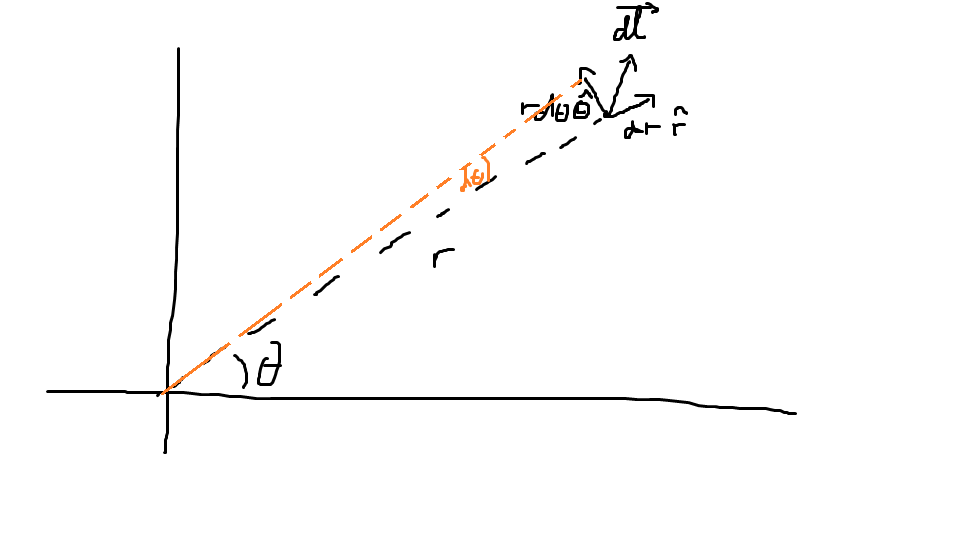
I'm getting really confused about when can I use

$$ \vec{v} = \dot{r}\hat{r} + \omega \times r \hat{\theta} $$

and the following identity, which I'm not sure if it's vectorial (did I write it correctly?)

$$ \vec{v} = \omega r \hat{\theta} $$

I'd be really happy to see a formal proof of how those are developed, and to understand in which case do I use each of them. unfortunately I wasn't able find anything (probably I wasn't searching right, but I'm not sure what else to write other than "velocity of rotating object proof")

Answer 1

Imagine the scenario where you have an object (let’s say a coin) on a revolving platform.

The first term is simply the velocity of the coin as it moves across the platform, irrespective of the platform rotating. In the case where the radius is fixed, this term is zero as $\dot{r}=0$ for a constant radius.

There’s certainly more rigorous ways of deriving the second term, but in the case where omega and r are perpendicular (as is often the case in many simple problems), the cross product $v =(\omega \times r) = \omega r \hat{\theta}= v$

A convenient way of remembering this is to simply remember the equation for the length of a curve traced out by a given angle of a circle, $S = r\theta$, which becomes $v = r\omega$ after taking a time derivative (once again assuming that the radius is constant so that our $\dot{r}=0$)

Hopefully this makes sense, let me know if I can expand a bit more, I’m waiting at the dmv with little else to do!

Answer 2

For a formal proof, remember that in 2D $$\hat{r} = \cos \theta \cdot \hat{x} + \sin \theta\cdot \hat{y}$$ Because the object you're pointing at is moving, its direction vector has a derivative: $$ \frac{d}{dt} \hat{r} = (-\sin \theta)\cdot \dot\theta \cdot \hat x + (\cos \theta)\cdot \dot\theta \cdot \hat y = \dot\theta \cdot (-\sin \theta \cdot \hat{x} + \cos \theta \cdot \hat{y}) $$ But the last term in brackets is exactly the definition of $\hat{\theta}$ so $\frac{d}{dt} \hat{r} = \dot\theta \hat\theta$.

Now, for obtaining the velocity, just remember that $$\vec{v}=\frac{d}{dt} (\vec{r}) = \frac{d}{dt} (r\cdot \hat{r}) = \dot r \hat{r} + r\dot {\hat{r}} = \dot r \hat r + r\dot \theta \hat \theta$$ The last equality coming from what we proved earlier.

For 3d, the proof is very similar.

If you are interested in some intuition and not just calculus, look at the drawing I added. You can see that when you move a bit, you can look at the increments of length you did in every direction as the sides of a right-side triangle, meaning you moved $$ \vec{dl} = dr\cdot \hat{r} + r\cdot d \theta \cdot \hat{\theta}$$ so dividing by time will give $$ \frac{\vec{dl}}{dt} = \frac{dr}{dt} \hat{r} + r\cdot \frac{d\theta}{dt} \hat{\theta}$$