What is the current through the lamp?

Question

We have the following circuit:

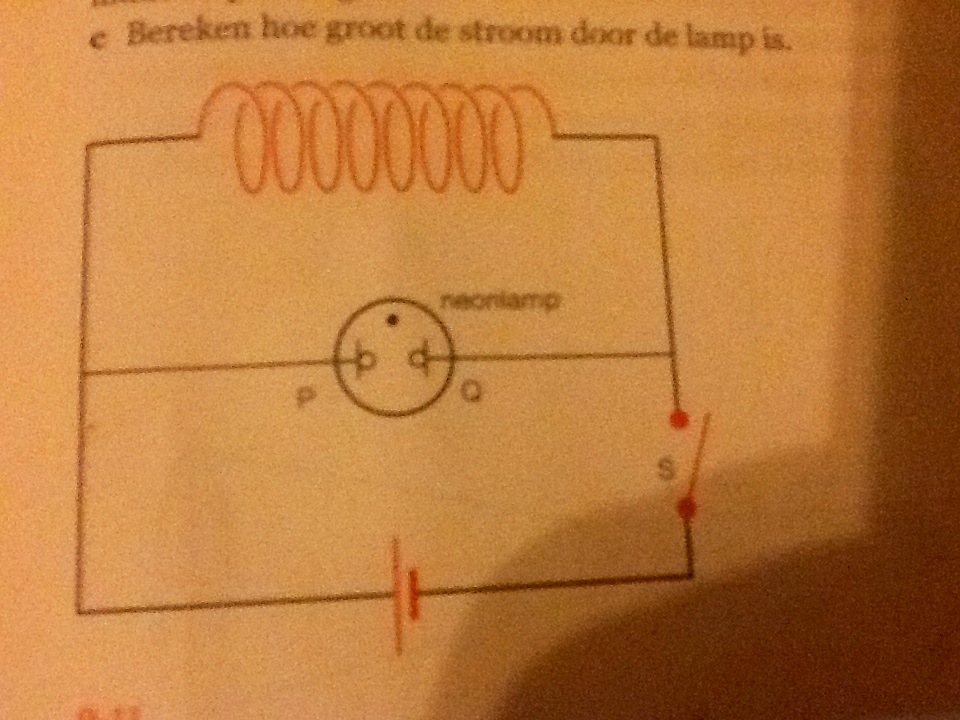
A neon lamp and a inductor are connected in parallel to a battery of 1.5 $V$. The inductor has a 1000 loops, a length of $5.0 cm$, an area of $12cm^2$ and a resistance of $3.2 \Omega$. The lamp shines when the voltage is $\geq 80V$.

• When the switch is closed, $B$ in the inductor is $1.2\times 10^{-2} T$.

• The flux then is $1.4 \times 10^{-5} Wb$

(calculated myself, both approximations).

You open the switch. During $1.0 \times 10^{-4} s$ there is induction. Calculate how big the current through the lamp is.

My textbook provides me with the following answer:

$U_{ind} = 1000 . 1.4 \times 10^{-5} / 1.0 \times 10^{-4} = 1.4 \times 10^{2} V$.

$ I = U/R_{tot} = 1.4 \times 10^{2} / (3.2+1.2) = 32A$

My concerns:

• How do we know that $1.4 \times 10^{-5}$ is $|\Delta \phi|$? This is the flux in the inductor while the switch is closed, but when you open it doesn't induction increase/decrease the flux? Or will the flux just become 0 and hence give us $1.4 \times 10^{-5}$ ?

• Why do we have to take the $R_{tot}$? What does the resistance of the inductor have to do with the lamp?

p.s. - This question can't be asked on electronics SE, since their site doesn't allow for such a question.

Answer 1

When you close the switch the inductor "charges", gaining magnetic energy and hence an associated flux. When you open the switch, there is a potential energy associated with the inductor, and hence it will "discharge", generating a current in the circuit. So under the assumption that all the flux discharges, then $\Delta \phi$ will be $1.4 \times 10^{-5}$ $Wb$.

Now that there is a current flowing in the circuit, the current will see all the resistances in the circuit, not just the ones in front of it (since the circuit is closed and the sums of the sources and potential drops around the whole circuit must be zero.)

One can consider only the resistance of the lamp if the resistance of the inductor was zero. But since it has a finite resistance (you could think of it like the internal resistance of a battery) you will have to consider the internal resistance of the inductor in series with the resistance of the lamp.

Answer 2

Yeah ok so this problem, is like I said a little silly. It seems like you have to assume the current drops to zero in the given time and therefore so does the flux. This gives you the first part, the induced voltage across the inductor. For the second part, it seems we simply have to apply Kirchoff's first loop rule and Ohm's law to find the current in the loop. This all seems very odd to me, because we are assuming the current is changing in order to induce a voltage, but also a single value for the current. Really, the current should be time dependent and the induction occurs for all time, not simply a finite amount. For the sake of completing this homework problem, we are done, but in reality we have to solve a differential equation and end up with exponential behavior.

Answer 3

I think the other answers cover your question about the change in flux. As to why $U/R_{tot}$ is used instead of $U/R_{lamp}$... it's not quite clear whether you're expected to find the current at a particular point on the circuit, or what point that might be. If I had to pick one current to characterize the circuit, it would be the current through a bit of wire that didn't have any parallel counterparts (or equivalently the sum of the currents through a set of parallel wires). The voltage drop has to be equal across both the lamp and the inductor, so the current is just $I_{tot}=V/R_{tot}$. If after this you're interested in the current through the lamp, you can find it easily by calculating the current through the inductor individually ($I=V/R_{ind}$), then $I_{lamp}=I_{tot}-I_{ind}$.

In your original question you don't say anything about needing to find the current through the lamp, but in one of your comments you imply that that's the quantity you're after - is that actually what the original problem statement asks for?