Why does foam in a rotating liquid accumulate near the centre?

Question

I first noticed this while having a coffee. When the coffee was rotating in the cup, most of its foam accumulated near the centre. I recreated the effect with some soap and water. The accumulated foam formed a beautiful dome. You can see the dome formation in detail in this video.

Side view

Top view

---

I wonder what causes this pattern formation. I would expect the foam to move away from the centre due to centrifugal forces, but that's not what I see. I believe there's something else to it. So here's the question in short:

Why does foam accumulate near the centre of rotating fluids?

Also, why there is a characteristic dome shape for the accumulated foam?

Answer 1

The water experiences a greater centrifugal force than the bubbles

Both the bubbles and the water experience a centrifugal force. However, since the centrifugal force is given by:

$$ F_c = m R \omega ^2 $$

You can see that a more massive object (the water) will experience a greater centrifugal force. From the perspective of the rotating frame, those forces would look like the pink arrows below:

Thus the water at the same $R$ as the bubble will flow around the bubble, shoving it closer to the center of rotation. Of course, the bubbles still rise to the surface, so they rise in a pile and cause the bubble bulge in your picture:

This is the same principle by which a centrifuge operates, but instead of throwing the heavier material to the outside of the rotating water, it throws the lighter objects towards the center of the rotating water.

Answer 2

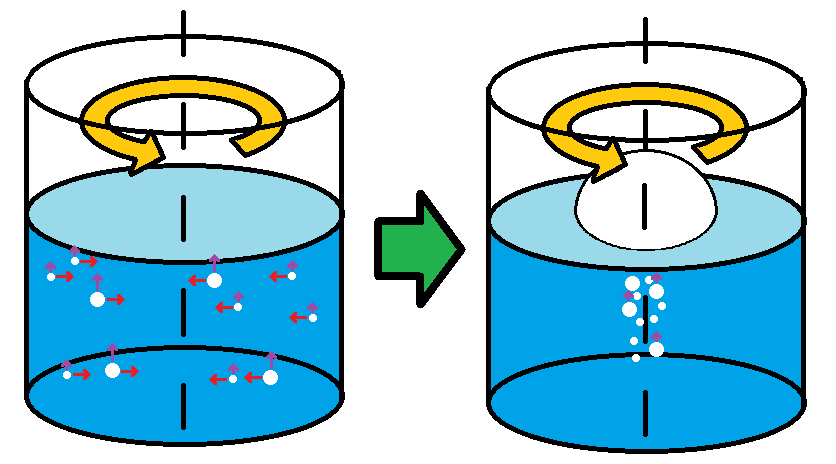
Well it is simple, first of all let us look at a sub problem. Let's ask what would be the shape of the surface of the fluid in case of a uniform angular rotation. I am, for simplicity, assuming that the fluid has no turbulence and it is uniformly dense.

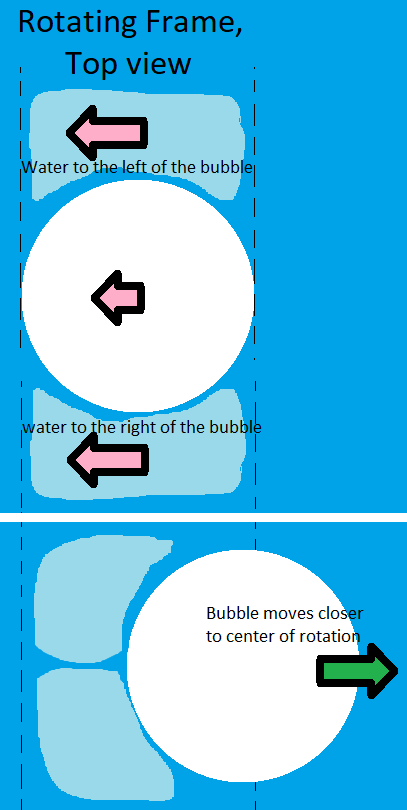
Every classical physics problem can be analysed using free body diagrams, and if you draw one for these, you will find that there are two forces acting on a mass element, one the centrifugal force on it (I have done the analysis from the frame of the axis) and other gravity. Now we use the following property of liquids: they can't withstand shear force, i.e. they will distribute in such a way that the net force on the fluid is perpendicular to the fluid surface.

Now we are ready to deal with the problem. If you analyse the free body diagram, you will find that $$\frac{dy}{dr}=\omega^2r$$

If you solve this differential equation you will get the following result $$y=\frac{\omega^2r^2}{2}$$ Which basically means that the fluid surface is like a parabola (technically it's a paraboloid)

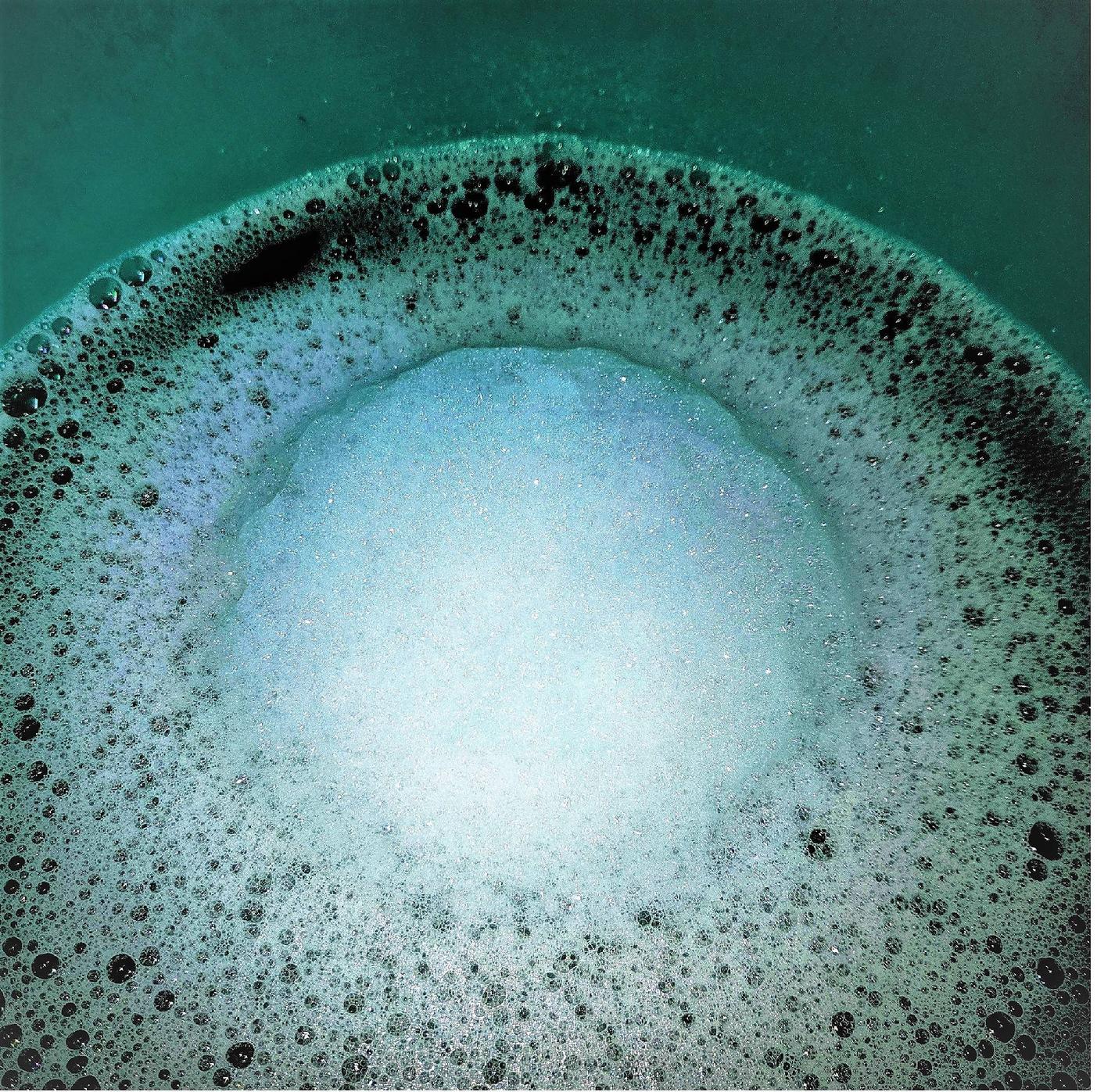
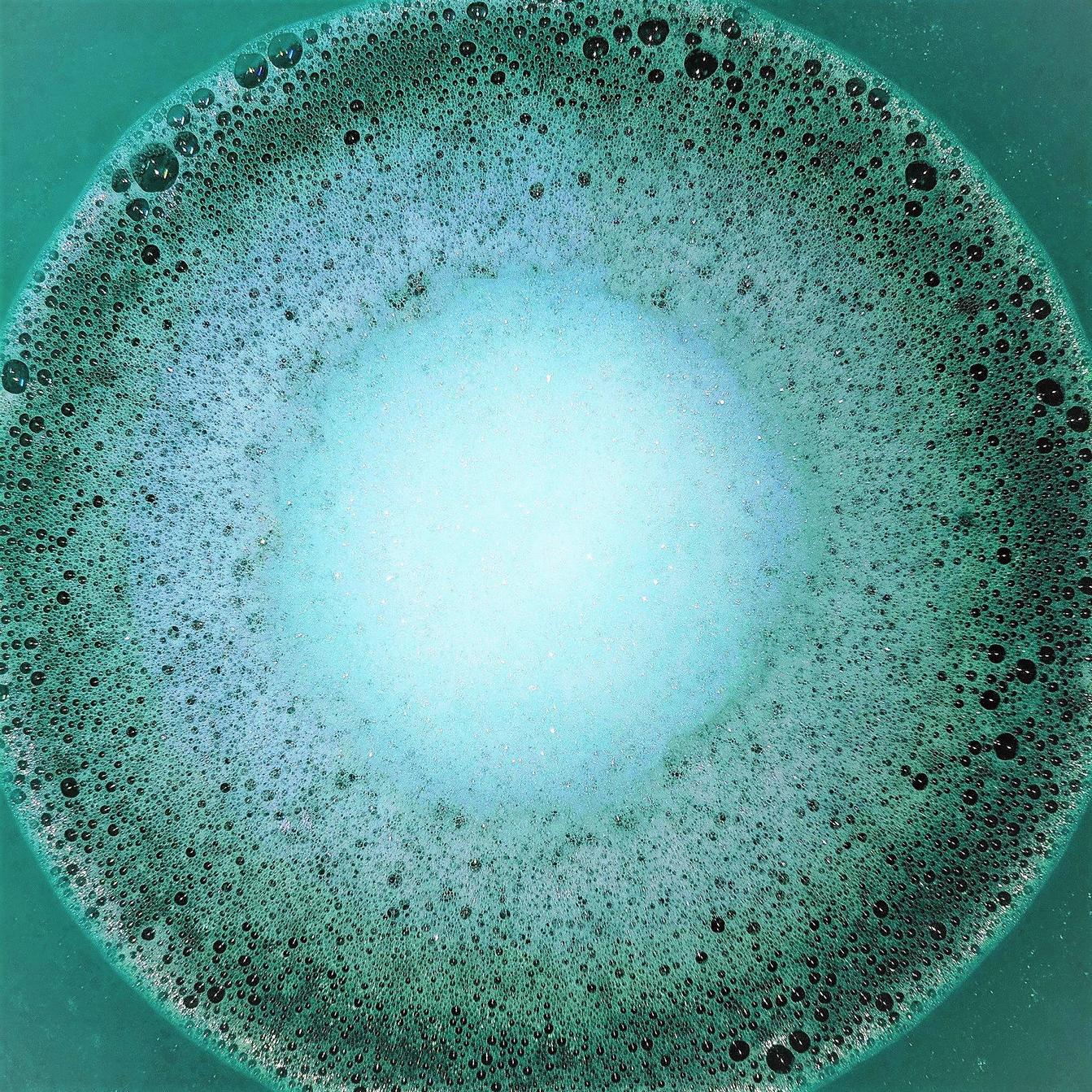
Now I am going to assume one more thing: that the density of the foam is much less compared to that of the fluid, which means that it isn't going to affect the distribution of the fluid.

Now from here it must be clear that the liquid is forming a kind of gravitational well (it is definitely not the right term, but is quite useful) which causes the foam to accumulate on the center.