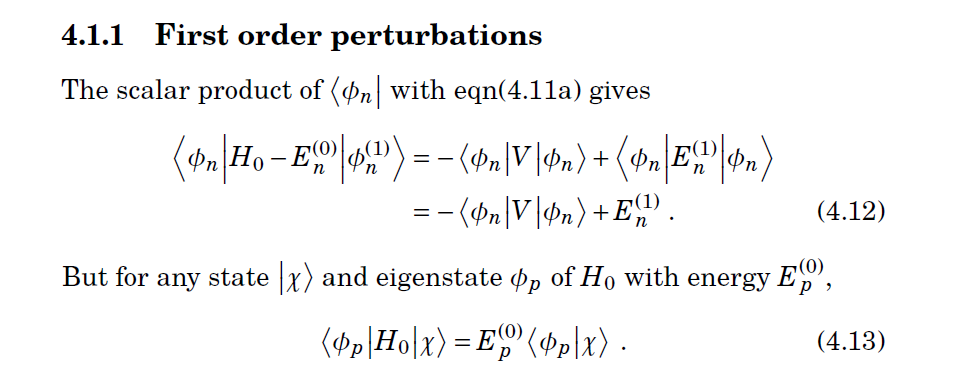
This comes from notes on perturbation theory I am learning in class.
I have seen this a few times but never really understood it: how is it that we can apply an operator to the conjugate state on the left when an operator is meant to operate on whatever is in front of it (or the right state in the notation)?
Since the Operator $H_0$ is Hermitian, $\langle\phi_p|H_0|\chi\rangle$ is equal to its adjoint $\langle\chi|H_0|\phi_p\rangle^*$. Now, we act to the right, and since $E^{(0)}_p$ is real, we obtain $E_p^{(0)}\langle\chi|\phi_p\rangle^*$ which is equal to $E_p^{(0)}\langle \phi_p|\chi\rangle$ since the unit operator is also hermitian.
