There is a lower limit for speed in Maxwell's speed distribution curve I.e. 0 but it doesn't has upper bound.It seems that the molecules of an ideal gas can move with infinite speed.Is it really possible that the molecules can move with infinite speed or is it understood that the upper limit is equal to speed of light in vaccum?
$\begingroup$
$\endgroup$
3
asked Oct 22, 2019 at 17:57
-
$\begingroup$ Note that as $v\to c$, the (classical) Maxwell distribution is no longer valid & you need the relativistic version (usually called Maxwell-Juettner distribution). $\endgroup$– Kyle KanosCommented Oct 22, 2019 at 18:03
-
$\begingroup$ @Kyle Kanos was there any fault in Maxwell's effort? $\endgroup$– Shreyansh PathakCommented Oct 22, 2019 at 18:05
-
2$\begingroup$ No, it was obviously derived about 50 years before relativity was discovered... $\endgroup$– Kyle KanosCommented Oct 22, 2019 at 18:05
Add a comment
|
3 Answers
$\begingroup$
$\endgroup$
3
Sure, the distribution goes off to $v\to\infty$, but the tail of the distribution drops off almost exponentially. Therefore, the probability of observing a molecule with higher and higher speeds becomes extremely unlikely.
I think it is still (classically) reasonable to not have a cut-off speed. Perhaps the molecules will collide in such a way so that one keeps getting hit in the same direction over and over. Relativistically, sure, I guess you could work out what the distribution would be.
I don't think it would make a huge difference though. The low probabilities should make stuff at that end pretty irrelevant. And with the relativistic correction you would need the distribution to drop faster than the classical case. Therefore, I would expect even smaller probabilities for large $v$.
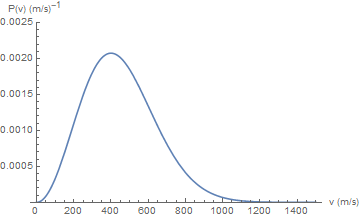
For a more numerical approach, here is a graph of the distribution for a nitrogen molecule at $T=293\,\mathrm K$
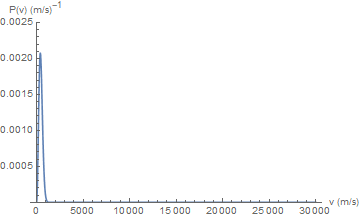
and here is the same plot but out to $v=.0001c$
As you can see, the probability of observing speeds even remotely close to the speed of light is practically $0$.
More precisely, the probability of observing a speed greater than $1500 \,\mathrm{m/s}$, which is $.0005\%$ of the speed of light, is $$\int_{1500}^\infty P(v)\,\text dv\approx3.4\times10^{-6}$$
Therefore, I would say there is no issue here, as the Maxwell distribution predicts that you will not have to worry about this relativistic limit.
answered Oct 22, 2019 at 18:05
-
$\begingroup$ So,is it sure that the upper bound is $c$? $\endgroup$ Commented Oct 22, 2019 at 18:08
-
$\begingroup$ @Unique For the Maxwell distribution function, no, there is no upper bound. It was not derived in a relativistic setting. $\endgroup$ Commented Oct 22, 2019 at 18:08
-
$\begingroup$ @unique The upper limit is infinity as long as this is much lower the $c$, since the distribution loses its validity at speeds of the order $c$. $\endgroup$– my2ctsCommented Oct 22, 2019 at 18:57
$\begingroup$
$\endgroup$
2
The Maxwell speed distribution curve is based on non-relativistic mechanics where the kinetic energy of a particle of mass $m$ with speed $v$ is $\frac{1}{2} m v^2$. In non-relativistic mechanics there is no limit on how fast a particle can move.
-
$\begingroup$ Thanks for the effort but how that answers my question? $\endgroup$ Commented Oct 22, 2019 at 18:06
-
$\begingroup$ added the phrase " In non-relativistic mechanics there is no limit on how fast a particle can move". $\endgroup$– jimCommented Oct 22, 2019 at 18:09
$\begingroup$
$\endgroup$
5
It's because Maxwell developed it before the Einstein had worked out special relativity, when there was no maximum speed known. If you want to rework it for special relativity you need to rework it in terms of special relativistic kinetic energy.
Specifically, the special relativistic version is: $$ f(p,x) = \frac{4\pi p^2}{V h^3}\exp\left(-\frac{1}{kT} \left[\sqrt{p^2c^2 + m^2 c^4} - mc^2\right]\right), $$ where $p$ is the special relativistic momentum, and it has no upper limit. $V$ is the volume the gas is in, and $h$ is Planck's constant. You'll need to figure out the normalization for this integral yourself, if you're curious. Figuring out the version of this that works for velocity, instead of momentum, is a little involved; it's an exercise in change of variables in calculus using the formula $$p = \frac{m v}{\sqrt{1 - \frac{v^2}{c^2}}}.$$
answered Oct 22, 2019 at 18:04
-
$\begingroup$ That does not look like the relativistic Maxwell equation I am familiar with. Do you have a link to the derivation of this form? $\endgroup$ Commented Oct 22, 2019 at 18:56
-
$\begingroup$ @KyleKanos I did it in my head just now, but I'm sure you can find a derivation somewhere - it's bog standard thermodynamics using the canonical formulation of mechanics (i.e. phase space). The number in a phase space volume is given by: $$f(x,p) = V^{-1} e^{-E/kT} \frac{\mathrm{d}^3 p \,\mathrm{d}^3x}{h^3}.$$ Put $p$ in spherically symmetric polar coordinates, and add an, honestly irrelevant, offset to $E$ to make it kinetic energy, and you get what I wrote. $\endgroup$ Commented Oct 22, 2019 at 19:47
-
$\begingroup$ Searching for "relativistic partion function" gives these results: en.wikipedia.org/wiki/… , phys.uri.edu/gerhard/PHY525/wtex91.pdf , etc. $\endgroup$ Commented Oct 22, 2019 at 19:51
-
$\begingroup$ I expected to obtain this form; might be different starting & ending points , will have to work on yours more. $\endgroup$ Commented Oct 22, 2019 at 20:17
-
$\begingroup$ @KyleKanos They're nearly the same. That one is just properly normalized, and the constants have been rearranged. $\endgroup$ Commented Oct 22, 2019 at 20:23