Renormalization group and summation of diagrams

Question

Currently I'm studying renormalization group, and I'm having trouble understanding the following statement which I see almost everywhere in books on QFT: renormalization group sums a series of divergent diagrams.

In particular examples, like 1-loop corrections to photon propagator, it is clear: we consider a following series 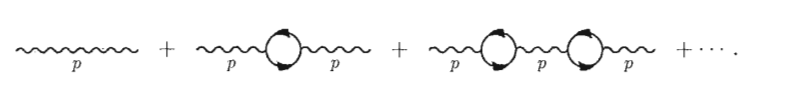It sums as a geometric progression and gives the desirable answer, same as RG equation provides if we consider a contribution to beta-function from the first diagram. But is there any way to construct and look at concrete series of diagrams, which we have resummed via the use of RG equation in a given order, in an arbitrary case, in order to find what contributions we missed?

Say, we have a $\phi^4$ theory. The beta-function in one-loop is $\beta(\lambda) = \frac{3\lambda^2}{16\pi^2}$ is given by a first divergent contribution to 4-point function - diagram with one "bubble"; the factor 3 comes from crossing symmetry. By solving the RG equations, we get for a running coupling constant on scale $p$

$\lambda(p) = \frac{\lambda(\mu)}{1 - \frac{3\lambda}{16\pi^2} \log p/\mu}$

where $\mu$ is the reference scale. If we expand the denominator, we see a series which looks like it's given by some set of perturbative expansion terms; the first one is just the "one-bubble-diagrams". But I wasn't able to find what diagrams correspond to different terms even in the next order, especially to reproduce the strange factor of 9.

Answer 1

There isn't a sense in which the extra terms obtained by using the renormalization group correspond to any specific subset of Feynman diagrams. As you have already pointed out, for $\phi^4$ theory, it is not true that you just sum the "bubble" diagrams; you need to calculate all of the corrections, and then these corrections will contain the correct $\log^2(p/\mu)$ dependence predicted by expanding your effective coupling, but will also contain other terms.

The argument that you can predict the form of these higher-order terms can go as follows, using dimensional regularization. At first-order in $\phi^4$ theory, you obtain $$ \Gamma^{(4)}(p) = \mu^{\epsilon} u_0 \left\{ 1 - \frac{3 u_0}{16 \pi^2 \epsilon} \left[ 1 + \epsilon \log(p/\mu) \right] + \cdots \right\}. $$ Here, I am taking $\Gamma^{(4)}(k_i)$ to be the four-point function, defined with total momentum $p$ flowing through it. The omitted terms in the ellipsis are momentum-independent and finite for $\epsilon \rightarrow 0$.

At this point, one introduces a renormalized coupling to subtract the divergent term, $$ u_0 = u \left( 1 + \frac{3 u}{16 \pi^2 \epsilon} \right), $$ and this is sufficient to renormalize the correlation function to $O(u^{2})$.

How do we use this result to obtain information about higher-order contributions? Well we can already read off a particular $O(u^3)$ contribution just from noticing that we will have a term $$ \Gamma^{(4)} \supset \frac{18 u^3}{(16 \pi^2) \epsilon} \log(p/\mu) $$ coming from the counter-term for $u_0$ defined above. Such a term is initially very worrying, because it is a momentum-dependent divergence - we cannot subtract this using counter-terms! Therefore, for the theory to make sense, it must be that a corresponding divergence with identical momentum-dependence will arise at two-loop to cancel this off. Of course, within dimensional regularization, the $log(p/\mu)$ dependence always occurs due to expanding a function like $(p/\mu)^{\epsilon}$. In particular, the above divergence would need to come from a term like $$ -\frac{18 u^3}{(16 \pi^2) \epsilon^2} (p/\mu)^{\epsilon} = -\frac{18 u^3}{(16 \pi^2) \epsilon^2} - \frac{18 u^3}{(16 \pi^2) \epsilon} \log(p/\mu) - \frac{9 u^3}{(16 \pi^2)} \log^2(p/\mu) $$ Therefore, if this term shows up at two-loop (and it needs to in order for this renormalization scheme to make sense), it follows that one also needs the $- \frac{9 u^3}{(16 \pi^2)} \log^2(p/\mu)$ term. But in this particular case, the term is generated by several (all?) two-loop diagrams, which in turn also contribute other terms which one-loop RG doesn't know about.