Are there two ways of representing a vector i.e., parrallelogram and resolution?

Question

the question was:

The component of a vector is

(a) Always less than its magnitude

(b) Always greater than its magnitude

(c) Always equal to its magnitude

(d) None of these

according to me ans will be none of these because

The magnitude of the component of a vector (projection) may be less than or equal to the magnitude(never more than it.) of the vector itself which will depend on what you are taking the components along. The magnitude of the component may be equal to the magnitude of the vector if and only of the projection is taken along itself, otherwise, it will always be less. For instance, consider a vector 4i where i is a unit vector along the x-axis. Now the magnitude of the component of this vector along the x-axis is 4, same as that of the vector. Now, consider a vector 3i+4j, where i and j are unit vectors along x and y-axis respectively, the magnitude of this vector is 5 but the magnitude of components of this vector are 3 and 4 along the x and y-axis respectively.

but my teacher told me:

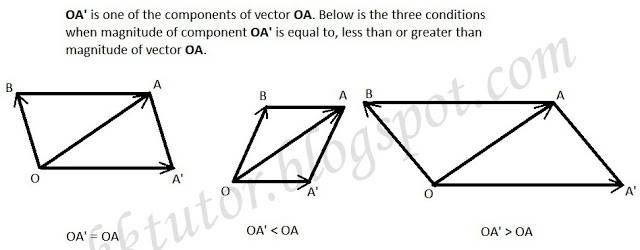
The component of a vector may be less than, greater than or equal to its magnitude.

i didnot understood how the component can be greater than the vector itself

on asking he showed me this :

my question is : can a vector have components in any set of two directions you choose that may not be mutually perpendicular. ?

Answer 1

. . . . can a vector have components in any set of two directions you choose that may not be mutually perpendicular. ?

Yes.
If $\hat a$ and $\hat b$ are two unit vectors (axis directions) which are not colinear to one another then any vector $\vec c$ can be resolved into two components $a$ and $b$ such that $\vec c = a\,\hat a + b\,\hat b$.
Notice that the only condition for the unit vector (axis directions) is that they must not be colinear to one another, ie they can be orientated at any angle relative to one another other than $0^\circ$ or $180^\circ$.

Perhaps an example will help?

Suppose there is a displacement from an origin of $6$ in the direction North-East and the two chosen axes are $\hat y$ which is the direction North and $\hat x$ which is in the direction East.
Then this displacement of $6$ in the North-Easterly direction is the same as a displacement $3\sqrt 2$ East then $3\sqrt 2$ North.
This displacement can be written as $3\sqrt 2 \, \hat x + 3 \sqrt 2 \,\hat y$ and these two components are shown as red vectors in the diagram below.

Now consider a displacement equal to $6$ North_West, $\hat {y'}$, followed by a displacement of $6\sqrt 2$ East, $\hat x$.
The total displacement can be written as $6 \, \hat {y'}+ 6 \sqrt 2 \,\hat x$ and these two components are shown as blue vectors in the diagram below.

Answer 2

Of course vector can be decomposed into any set of two directions chosen arbitrary. That's the basis of vector algebra. Mutually perpendicular components exists only in Euclidean space which is sub-set of more general vector representation rule.

Another way to think, is that rectangle is a special case of parallelogram where all inner angles are $\frac{\pi}{2}$.
Parallelogram is a special case of Trapezoid. And so on, until the most general case of polygon.