On proving the $E$-field is null inside the layer of a conductor having a cavity

Question

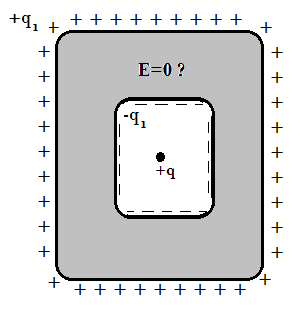
Let us consider a conductor having a cavity (both can have an arbitrary shape) wherein a positive charge $+q\,$ is located:

At the electrostatic equilibrium, the inner wall of the conductor will have a negative charge $-q_{1}$, and the outer wall a positive charge $q_{1}$.

The $E$-field in the region between the inner and outer wall is determined only by $+q$ and $-q_{1}$. How can one prove the total $E$-field in the layer is null?

The electrostatic equilibrium argument only tell us the potential is constant on the outer and inner surface of the conductor, it does not give any additional information about the $E$-field or the potential inside the thickness since there is no charges in it. Also, we cannot claim $q_{1}= q$, because, since we don't know the $E$-field within the layer, we cannot apply Gauss's law. How can we get out of this impasse?

Answer 1

It is important to realize that conductors inside contain mobile electrically charged particles, so called "charge carriers", even if the conductor is macroscopically neutral inside.

Since electrostatic equilibrium is assumed, there are no electric currents anywhere in the body or on its surface. In real conductors, the weakest macroscopic electric field inside the conductor means the mobile charge carriers present in the conductor (electrons in metals, or also holes in some semiconductors) experience electric force due to that electric field. If that was the only external force, it would make the mobile charge carriers move in concerted way and that means macroscopic electric current inside would occur.

If that current does not occur, either the electric forces on all charge carriers is counterbalanced by other force (for example, magnetic force can do that when the body moves through magnetic field) or, if there is no such counterbalancing force, the only possible conclusion is that electric force must be zero, hence electric field inside must be zero.

So, for conductor at rest with no other forces pushing the mobile charge carriers, and no current inside, the electric field inside must be zero. This is accomplished by special arrangement of electric charge near the surface of the conductor, whose electric field inside cancels any possible external static field, so that total field inside is zero. This is possible unless the external field is extremely high. In case the body is in vacuum in too high external electric field, the electric charge will jump out of the conductor surface and the system will no longer be in equilibrium.