1:
The answer to any question along the lines of "why is this term not present in the Standard Model Lagrangian" is always the same: because it works experimentally.
You could make a theory with 9 gluons, that would be a Lagrangian with a $U(3)$ symmetry and hence $9$ gauge fields. Then you would go to the experiment and see that you cannot adequately describe what you see.
2:
The correct interpretation is as follows.
You choose an $SU(3)$ symmetry for your Lagrangian. I.e. if you Lagrangian has terms like $\partial \psi \cdot ( \partial \psi)^\dagger$, you say this is invariant under $\psi \rightarrow g\psi$ where $g\in SU(3) = \mathrm{e}^{\mathrm{i}\sum_j\phi_j \lambda_j}$. $\lambda_i$ are the generators of the group, in this case known as Gell-Mann matrices. For $SU(N)$, you have $N^2-1$ generators, so $SU(3)$ has $8$ Gell-Mann matrices.
You choose to make this phase symmetry local, i.e. the phases $\phi_j$ are not global but a function of space $\phi_j(x)$. If you plug $g\psi$ in the Lagrangian now, you have extra terms $\propto \partial \phi_j$.
You requre local phase invariance, for it has been phenomenologically deemed a law of nature (read: it has never been observed to break down experimentally). For this invariance, you need to "get rid' of the spurious $\propto \partial \phi_j$ terms that spoil the local invariance.
How? You include gauge fields $A_j$ in the original Lagrangian, which you know can undergo gauge transformations $A_j\rightarrow A_j + \partial\Lambda_j$ without affecting the physics. You then match $\Lambda_j$ and $\phi_j$ to be equal and opposite, so as to cancel out.
How many gauge fields do you need? As many as required to cancel all $8$ independent phase terms for $g\in SU(3)$. So $8$ gauge fields. Which we call gluons.
This is why there are $8$ gluons.
You look at experimental data, and the results fit.
2a
Why did I choose $SU(3)$, and not $U(3)$?
Short answer: experiments agree with $SU(3)$.
Long answer:
$U(3) = SU(3)\times U(1)$, i.e. the same reasoning above would apply but we'd just need another Gell-Mann matrix to generate the extra $U(1)$ symmetry. The generator for $U(1)$ is the identity. So the extra gauge field you'd need to balance this local phase is independent on the specifics of $SU(3)$, i.e. it would be the same were $SU(3)$ symmetry not to exist at all. Mathematically, we say that it would be a singlet under $SU(3)$ (more about this in 2b).
Now, particles and fields participating in the strong force $SU(3)$ have a charge associated with it, called colour. The singlet would have no colour. This is analogous to neutral particles having no electrical charge and therefore not participating in electromagnetic interactions (and vice versa).
$SU(3)$ has a particular property (due to its non-linearities) called colour confinement. The strong force increases with distance, meaning it would take infinite energy to have two coloured objects at an infinite separation. Hence, you cannot have free coloured objects. They can only be bound and hence confined.
So if $U(3)$ were correct, you'd have a gluon that is a colour singlet and that could therefore be observed free-roaming around, not tightly confined to the nucleus.
No experiment so far has seen this, hence $SU(3)$ is taken instead.
2b
The decomposition thing that you quote is now a different story.
$SU(3)$ deals with $3\times 3$ matrices. The 'S' means that you restrict these matrices only to those which have determinant $=1$.
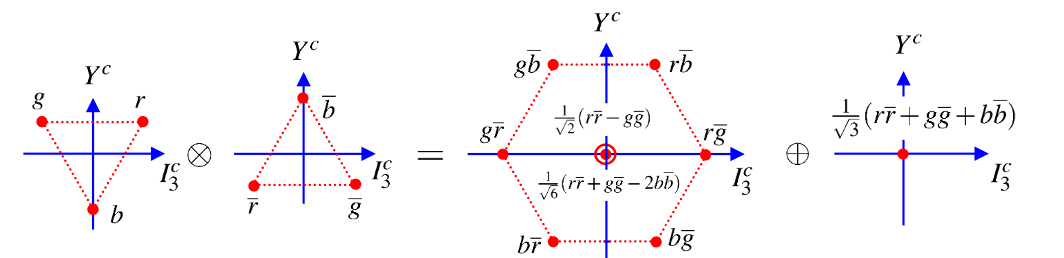
But let's consider all $3\times 3$ matrices, i.e. constructed by the product of two $3$-vectors (denoted by bold numbers $\mathbf{3}$).
Matrices and vectors are meaningless in physics unless you specify a basis. So let's choose bases.
red : $r = (1,0,0)$, green: $g = (0,1,0)$ and blue: $b = (0,0,1)$.
Let's take the product between a generic colour and anti-colour vector:
$$ \mathbf{3} \otimes \bar{\mathbf{3}} = \mathbf{8} \oplus \mathbf{1}, $$
which means you can decompose the representation of the LHS into two independent bits. Leaving aside all the group theory related maths, physically you interpret this as $8$ gluons and the $1$ colour singlet discussed in 2a.
And you can draw these pretty "Eightfold way" diagrams (source):
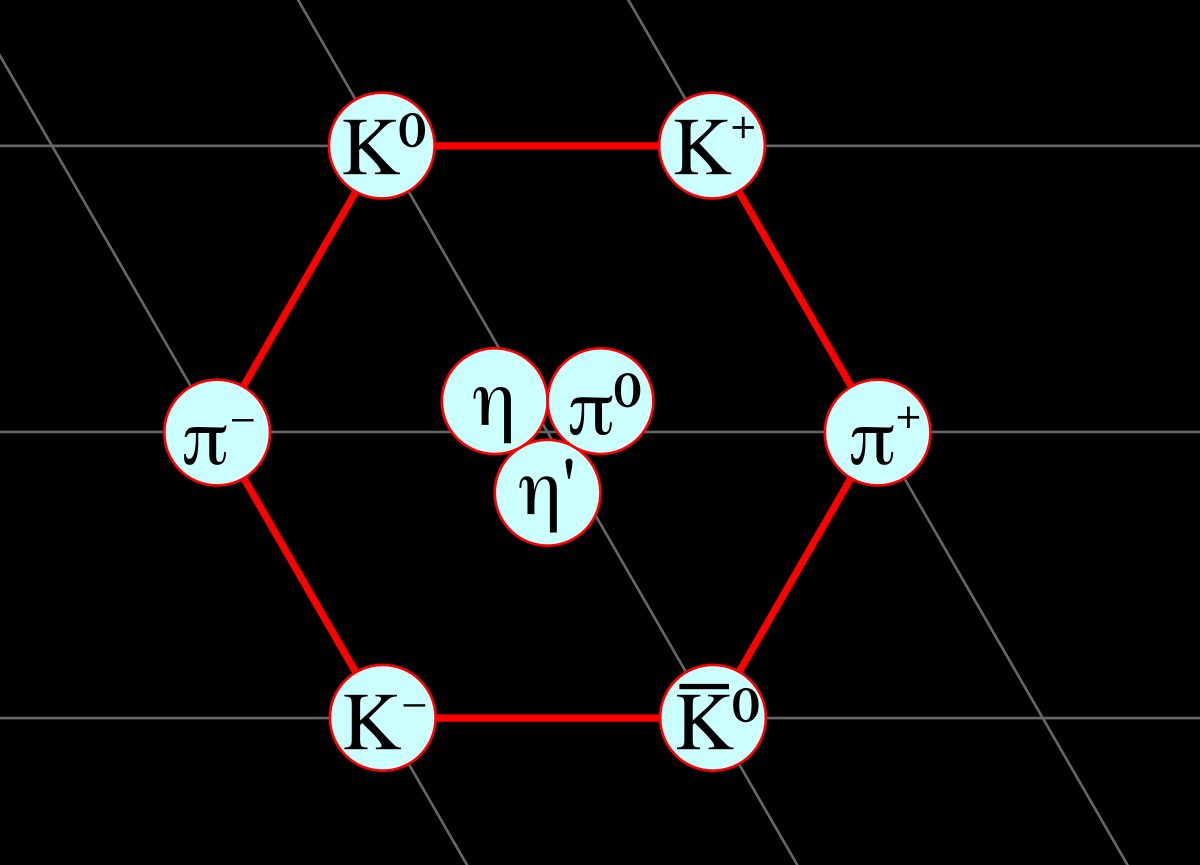
up $u = (1,0,0)$, down $d = (0,1,0)$ and strange: $s = (0,0,1)$.
Again, let's take the product between a generic quark and anti-quark vector:
$$ \mathbf{3} \otimes \bar{\mathbf{3}} = \mathbf{8} \oplus \mathbf{1}, $$
which we interpret as the $\boldsymbol{\eta}'$ meson singlet, and the $\;\boldsymbol{\lbrace}\boldsymbol{\pi}^{+},\boldsymbol{\pi}^{-},\boldsymbol{\pi}^{0},\mathbf{K}^{+},\mathbf{K}^{-},\mathbf{K}^{0},\overline{\mathbf{K}}^{0},\boldsymbol{\eta}\boldsymbol{\rbrace}\;$ octet (see here for maths).
3
Depending on which basis you choose, i.e. on what $\mathbf{3}$ corresponds to physically, it could be a meson, a yet-experimentally-not-discovered gluon etc.


