1
$\begingroup$

This is a question from Class 11 Physics NCERT. 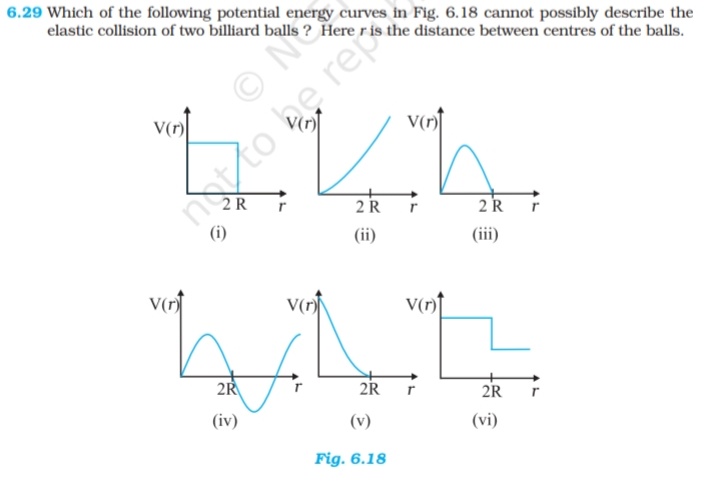enter image description here

The answer is that only graph (v) is correct and the reason given here is that potential energy varies inversely with separation. But does that always have to be the case?

Also, I had learnt that during elastic collision, all the kinetic energy momentarily converts to elastic potential energy due to deformation, which means that potential energy should be maximum. But here the graph shows that the potential energy is minimum during collision.

I'm certain I am missing a key concept here. Can anyone help me understand where I'm going wrong? Thank you.

Improve this question
$\endgroup$
2
  • $\begingroup$ Notice the label on the horizontal axis? What happens to the separation between the balls' centers during a collision (unless there is more text that you haven't shown us I suppose that you are expected to intuit that $R$ is the radius of an individual ball)? $\endgroup$
    – dmckee --- ex-moderator kitten
    Commented Sep 4, 2019 at 19:55
  • $\begingroup$ There isn't any more text but I assumed that R was the radius of the balls too. $\endgroup$
    – laksheya
    Commented Sep 4, 2019 at 19:57

1 Answer 1

Reset to default
1
$\begingroup$

But here the graph shows that the potential energy is minimum during collision.

But it doesn't. Keep in mind that $r$ becomes smaller as the balls get closer together and then becomes larger as they move apart. The horizontal axis is not time. So the potential energy actually increases to a maximum at the minimum separation, and then it goes back down as the balls separate.

As for the other points, it makes sense that you want $V$ to increase as $r$ decreases. This is because $$F=-\frac{\text dV}{\text dr}$$ The inverse relationship between $V$ and $r$ means that the force is pushing the balls in contact towards being farther apart.

None of the other answer choices give you the correct force behavior (check for yourself).

To answer your title question, this does not always have to be the case. But for your system we don't want any attraction between the balls, so we do want this behavior here. It doesn't necessarily have to be inversely proportional, but it does need to monotonically decrease as $r$ increases (i.e. an inverse relationship).

Improve this answer
$\endgroup$
14
  • $\begingroup$ Thank you for the answer! Did you mean force= -dV/dr? Also here: "The inverse relationship between V and r means that the force is pushing the balls in contact towards being farther apart." Is this because we want the system to have minimum potential energy? $\endgroup$
    – laksheya
    Commented Sep 5, 2019 at 3:57
  • $\begingroup$ @laksheya We want $dV/dr$ to be decreasing with increasing $r$ (force pushing to larger $r$) and we want this decreasing behavior to become weaker at larger distances (stronger force for smaller separation). I'm unsure what you mean by having the potential energy at a minimum, since the potential energy changes during the collision. $\endgroup$
    – BioPhysicist
    Commented Sep 5, 2019 at 9:51
  • $\begingroup$ By your argument, wouldn't the van der Waals and Lennard-Jones potentials be disallowed? $\endgroup$
    – garyp
    Commented Sep 5, 2019 at 10:52
  • $\begingroup$ @garyp Can you expand more on that? I'm not sure I follow. Do colliding billiard balls follow those potentials? $\endgroup$
    – BioPhysicist
    Commented Sep 5, 2019 at 11:51
  • $\begingroup$ @AaronStevens Oh sorry I misunderstood you earlier. Thank you! $\endgroup$
    – laksheya
    Commented Sep 5, 2019 at 13:33

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.