Your intuition wasn't accounting for an important factor, and that is why you got the incorrect answer.
If you're only considering the mass at the cart at any point in time, and the acceleration required to move a cart of that mass, your intuition would be correct. There is more going on than that though, and this is what seems to have been missed by your intuition.
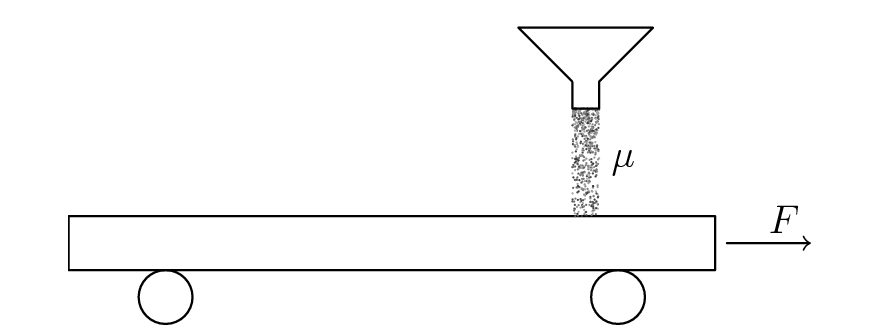
Consider what is happening to the falling sand. The sand is falling without any horizontal velocity, but the car is moving horizontally. For the sand to move with the cart, it needs to gain a horizontal velocity. To analyze how this might change the motion, it's easiest to consider conservation of momentum.
At any given point in time, sand is falling, and that sand requires a change in momentum to move with the car. This means that the faster the car is moving, the greater the impulse that has to be added to the falling sand along with the increased resistance to movement that is induced by the increased mass.
This adds another term to the relationship between acceleration and time, and this term will have to depend on the cars velocity. This leads to a differential equation relating the two.
An example of the derivation can be found on the Wikipedia page for Variable-mass system (accretion).
I will leave the specific derivation of this answer to you.