The relative phase between a superposition of H and V will typically not be preserved by a polarization maintaining fiber.
Linear Birefringence in Optical Fibers
To understand this let’s consider what problem a PM fiber is solving. T
The fiber core is made out of glass which has a certain index of refraction. If the glass is isotopic then the index of refraction is the same for both polarizations. However, if there is any anisotropy (due to strain in the core material as the fiber makes a tight bend or sustains a thermal gradient) then the index of refraction will be slightly different for H vs. V polarization’s of light. The relative phase collected by light traveling through a material is given by
$$
\phi = n k L
$$
Where $n$ is the index of refraction, $k$ is the (vacuum) wave vector of light and $L$ Is the length of the material.
Since the phase difference scales with $L$ we see that a tiny difference in $n_H$ compared to $n_V$ can result in a major ($\gg 2\pi$) phase difference between H and V by the end of the fiber. A material which gives a different phase for H and V is said to exhibit linear birefringence.
Note that the power in H or V does not change. Only the phase. However, this change in phase can convert diagonally linear polarized light into elliptical or circularly polarized light which may be problematic for some applications.
the big problem is that the relative phase is uncontrolled. If someone bumps the fiber or the air conditioning changes by a degree the birefringence can change resulting in a different output polarization. This instability can be ruinous for particular applications.
Polarization Maintaining Fibers
How do PM fibers solve this problem? The approach taken to reduce polarization instability in a PM fiber is to build in a large anisotropy into the fiber. This anisotropy can be realized by putting multiple materials into the cladding of the fiber which strains the core along a particular axis.
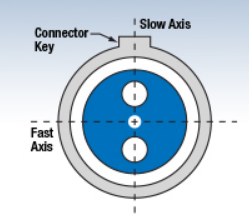
The outer blue circle represents the fiber cladding. The central inner circle is the core. The upper and lower white circles are different glass materials. The presence of these materials on the vertical axis (but not on the horizontal axis) creates an anisotropic strain in the core of the fiber.
This means that even under ideal conditions (no thermal gradients or strain) the fiber will exhibit a large difference in $n_V$ and $n_H$. That is, the fibers have a large built in birefringence.
How does this solve the problem when birefringence was the problem in the first place?? The answer involves thinking about two things. It involves thinking about the axis of the birefringence (which corresponds to the axis along which the strain is applied, either intentionally or unintentionally) and the magnitude of the birefringence (essentially how different is $n_H$ from $n_V$.
First suppose that light is injected which has a polarization directly along one of the birefringence axes (call it the slow axis with a smaller index of refraction). It will collect some arbitrary phase but it will maintain its linearity. Now imagine straining the fiber. This will create new strain in the core. However, since there is a large built in strain into the core this new applied strain is unlikely to change the direction of the overall strain within the core*. This means that even in the presence of external strain the fiber maintains the same birefringence axis. The overall phase of the light might change as a result of this new strain but the polarization will remain unchanged.
Contrast this with the case for a non-PM fiber. For a non-PM fiber imagine the fiber is initially strained so that it has a birefringence axis and suppose light is initially linearly polarized along this axis. This means the light will maintain that linear polarization throughout the fiber. However, now imagine the fiber is strained differently such that the total strain axis changes direction. In fact, imagine the strain axis is such that the original light is now polarized diagonally with respect to the new strain axis. This means that the light which was originally polarized along the slow axis is now a superposition of fast and slow axis polarized light. These two components can gain a relative phase shift now and the light can again become elliptically or circularly polarized. You can see how this can be especially problematic when you consider that the direction of the strain axis will likely be different in different parts of the length of the fiber.
So we see that for light polarized along the fast or slow axis of a PM fiber the solution is to add a built in strain so that the direction of the birefringence axis is stable in time. This means that light which is originally aligned with the strain axis of the fiber will stay aligned with strain axis even in the presence of external strain as desired.
To answer the actual question
All of the above was only background to answer the actual question. The actual question already assumes knowledge that PM fibers work for light polarized along the fast or slow axis. The question is what happens to light which is not aligned to the fast or slow axis of a PM fiber.
The answer is that the polarization will be messed up. Why? Suppose light is sent in diagonal to the strain axis of the fiber. This light is a superposition of fast and slow axis light. If the fiber is now externally strained the relative index of refraction for the fast and slow axis can still change. For example, a strain can be applied perpendicular to the main strain axis. While this will not change the overall direction of the strain axis dramatically, it will change the strain in the unstrained axis quite a lot (compared to what was originally zero). This means that the index of refraction for the slow vs. fast axis can have a big relative change. Because the light is a superposition of fast and slow axis aligned this relative phase shift will again allow the light to become elliptically or circularly polarized.
*This is because the built in strain is represented by a long vector and the external strain is represented by a small vector. No matter which direction the small vector is pointing, when you add the two vectors together, the result will be pointing in basically the same direction as the big vector.

