As to your question: "Can it be explained?"
No, it cannot. However, what can be done, and is done by all authors, is to demonstrate that the theory is self-consistent. No self-contradiction can be construed. That is in itself a very powerful thing.
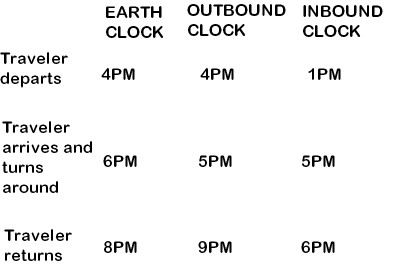
Any traveller is always moving, in the sense that you are moving forward in time. If you just stay where you are then when your journey is plotted in a diagram your journey is plotted as a straight line. Another traveller separates from you, goes far away, makes a U-turn, and rejoins you. That journey, when plotted in a diagram, is not straight. Spatially it is a longer line.
Special Relativity asserts:
For the traveller who does not travel along the spatially shortest path less proper time elapses.
(Think of the word 'proper' in 'proper time' as related to 'property'. Your proper time is your own time. 'Proper time' is the standard expression in this context.)
About acceleration
To get to my overall point I need to discuss a number of things about acceleation. Bear with me (or jump ahead to paragraphs with words in bold)
Think of measuring your own acceleration and inferring your current position from that as a higher order form of dead reckoning.
Dead reckoning at sea is accumulating velocity measurements. (If you are unfamiliar with the concept of dead reckoning at sea, I recommend you make yourself familiar with it.)
As we know, velocity is the first time derivative (derivative of position with respect to time)
Acceleration is the second time derivative (double derivative of position with respect to time)
With an Inertial Measurement Unit you can do the acceleration counterpart of dead reckoning.
Today's navitagion systems (such as used in cars) use Inertial Measurement to crosscheck the known current position.
Knowing your acceleration and direction of motion continuously means you can reconstruct your velocity (relative to some origin) continuously, which in turn means you can reconstruct your position (relative to some origin) continously.
When two spaceships depart from each other, and make a large journey, they can rejoin by both returning to the point where they were together, using the above described accelerational dead reckoning.
Special relativity asserts that if you measure your acceleration accurately, and you use that information to plot your motion (relative to some chosen origin) you can accurately predict how much proper time will have elapsed for you as compared to another traveller (whose journey is also known and plotted). (When you and the other traveller have rejoined you can proceed to compare elapsed proper time.)
It is this measurability that makes the difference.
For contrast: in the case of relative velocity there is no such thing as measuring that one is actually motionless and the other is moving. Well, if there is nothing measurable anyway then how about not admitting it in the theory altogether? As we know, that is what special relativity does: special relativity asserts that velocity is inherently relative.
The point is: this does not extend to acceleration.
Whether or not you are acccelerating is measurable.
In special relativity acceleration is just as fundamental as in newtonian dynamics.
My best guess is that this is the key point:
What I always see is that novices automatically apply the following reasoning: if position is inherently relative, and velocity is inherently relative, then surely acceleration must be relative too.
That expectation is understandable, but it is totally not how special relativity works. In special relativity acceleration is just as fundamental as in newtonian dynamics.
Time dilation
The thing that special relativity does is that it gives exact mathematics that allows you to calculate how much difference in elapsed proper time there will be between two travellers who have travelled different journeys, accumulating a different amount of distance travelled.
The key factor is difference in distance travelled. For example, a journey can consist of one long haul out, one U-turn, and one long haul back, or the journey can consist of a zigzag trajectory. You can plot those two trajectories to accumulate the same distance travelled. If the distance travelled is the same then the time dilation is the same, even though during the zigzag trajectory much more acceleration is accumulated.
The acceleration is necessary in the sense the without acceleration you cannot accumulate more distance travelled. But as I said, the calculation involves only difference in distance travelled, not difference in accumulated acceleration. That rules out thinking of the acceleration as the cause of time dilation.
Incidentally, since position and velocity are inherently relative, the calculation is itself independent of the choice of point of origin of the coordinate system. But for any choice of point of origin the difference in distance travelled comes out the same.
The mathematics of special relativity is self-consistent, and special relativity is corroborated by many different experiments. For the physics community that is sufficient.