Ondřej's answer is partially correct. In reality, you actually do have to worry about how far your eye is from the "eye lens" as the secondary lens system is called in a telescope. This is due to the need to match or over fill the eye's entrance pupil with the telescopes exit pupil. If you do not do this, you will see a small circle where the telescope is actually working, and it can limit your field of view.
You can also think about the telescope in the following way :
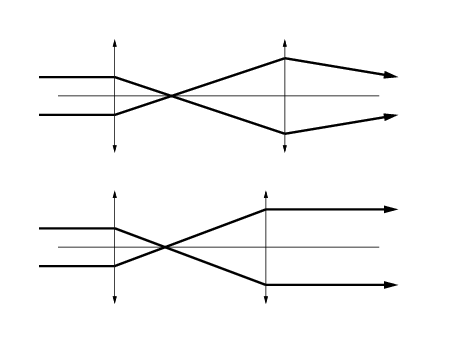
- The objective lens (the first lens or lens system) is forming an
aerial image (a really tiny one) near the shared focal point.
- The eye lens (the second lens or lens system) is magnifying that
image, in exactly the same way that you would use a simply magnifier
to observe beetles or something.
So, to select an eye lens first you want the most magnification which you can achieve without distortion or blurriness. You can do this by taking your eye lens system and looking at normal objects as if it were a magnifying glass. The calculation for the magnification of the eye lens is :
$$
M_{\text{eye}} = \frac{250mm}{f_{\text{eye}}}
$$
where $f_{\text{eye}}$ is the focal length of the lens (or lens system) and $250mm$ is the near point of the average relaxed human eye.
The telescope magnification is then given by
$$
M_{\text{telescope}} = -\frac{f_{\text{objective}}}{f_{\text{eye}}}
$$
The second thing that you have to worry about is the exit pupil matching as I stated above. A Galilean telescope will always have a narrower field of view and a little circle that you can see. For a reflective or Keplerian telescope, you can add field lenses to increase the field of view. This is basically what Huygens or Ramsden eyepieces do.
Since you want your eye's field of view to be maximized, you can do this by making sure that your exit pupil is located a reasonable distance outside of the telescope system. You will have to do calculations to find this distance, I suggest buying Grievenkamp's Field Guide to Geometrical Optics for a complete description of first order optics and a decent account of aberrations.