1
$\begingroup$

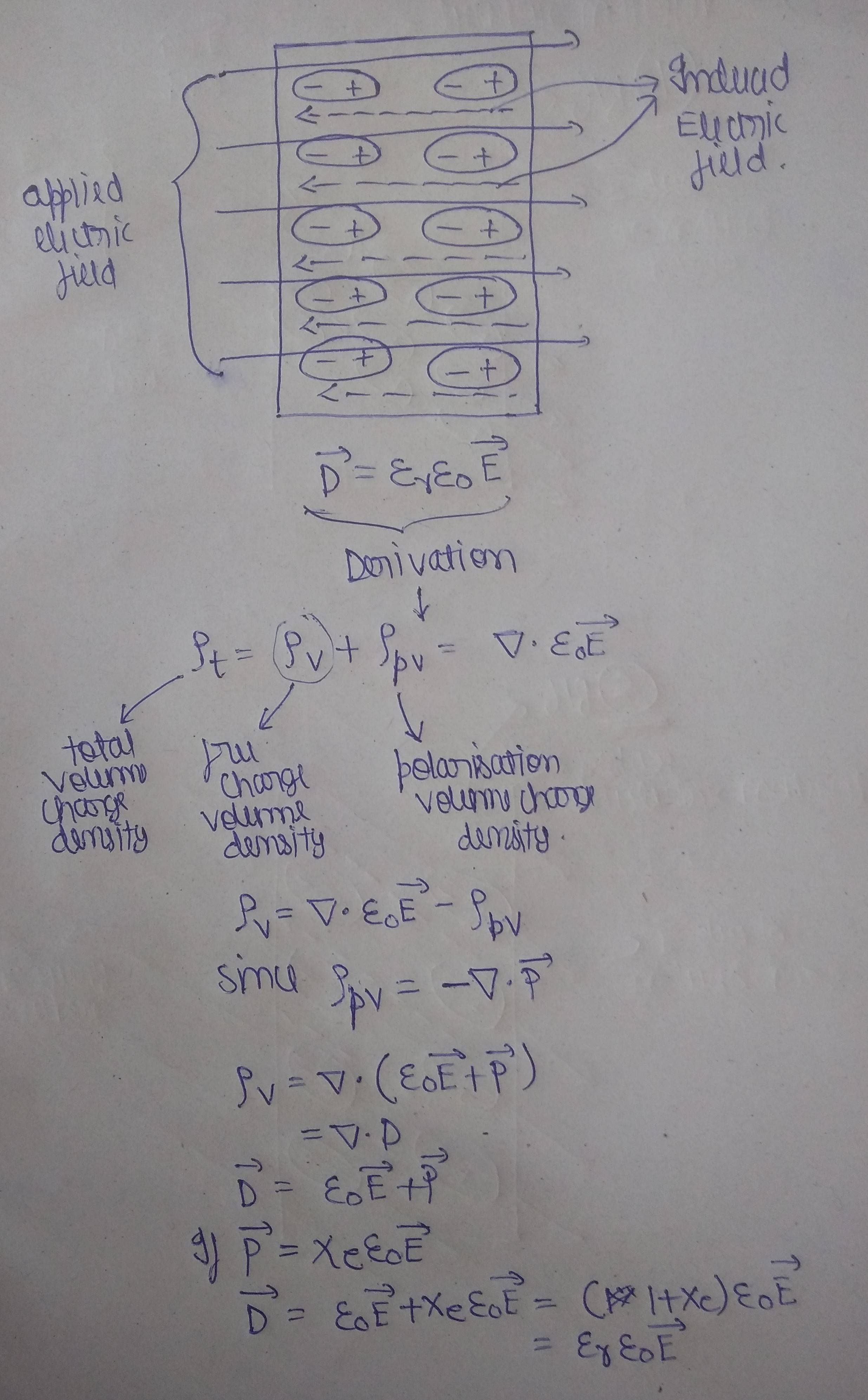
enter image description here

The above figure shows a polar dielectric medium in an external electric field which is normal to it's surface.

From the equation of D(electric flux density) we see that flux density increases but from the figure I see that flux density is decreasing as polarization field is in opposite direction.

I am surely missing out some point and I am not able to physically interpret the derivation.

Please help me understand where I am missing out and also the derivation.

Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

Your equation for electric flux density shows that it is proportional to the electric field. The electric field induced by the polarization of the dielectric opposes the applied electric field and therefore reduces the effective electric field according to

$$E_{effective}=E-E_{polarization}=\frac{σ}{kε_o}$$

Where $E$ is the external field, $k$ is the dielectric constant, $σ$ is the charge per unit area, and $ε_o$ is the permittivity of free space.

Since the electric flux density is proportional to the effective electric field, it decreases due to the dielectric, not increases.

For a more complete description, including the influence of the dielectric on the capacitance of a capacitor, take a look at the following:

http://hyperphysics.phy-astr.gsu.edu/hbase/electric/dielec.html

Hope this helps.

Improve this answer
$\endgroup$
7
  • $\begingroup$ Thank you so much for your answer. But my book clearly states that the flux density increases based on the equation D= (epsilon zero) *(electric field applied) + P. Even in the derivation this equation is written. So I wanted to know why my interpretation is wrong? For capacitor I have understood that electric field decreases so not a problem. I wanted about electric flux density $\endgroup$
    – Trilok Girish Kamagond
    Commented Jun 4, 2019 at 13:41
  • $\begingroup$ @TrilokGirishKamagond What is the title of the book? And what is $ε_ϒ$ in your equation. I assume $ε_o$ is the permittivity of free space. $\endgroup$
    – Bob D
    Commented Jun 4, 2019 at 14:01
  • $\begingroup$ @TrilokGirishKamagond Never mind, I see it is the relative permittivity. $\endgroup$
    – Bob D
    Commented Jun 4, 2019 at 14:04
  • $\begingroup$ @TrilokGirishKamagond Could it be that the electric field $E$ in your equation for electric flux density $$D=ε_{γ}e_{o}E$$ is the applied (external) electric field in my equation? That is, the field before the introduction of the dielectric? $\endgroup$
    – Bob D
    Commented Jun 4, 2019 at 14:08
  • $\begingroup$ @TrilokGirishKamagond In that case there is no conflict, since the effective field in the dielectric is the external field reduced by the polarization of the dielectric. Right? $\endgroup$
    – Bob D
    Commented Jun 4, 2019 at 14:30

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.