The problem is formulated as follows:
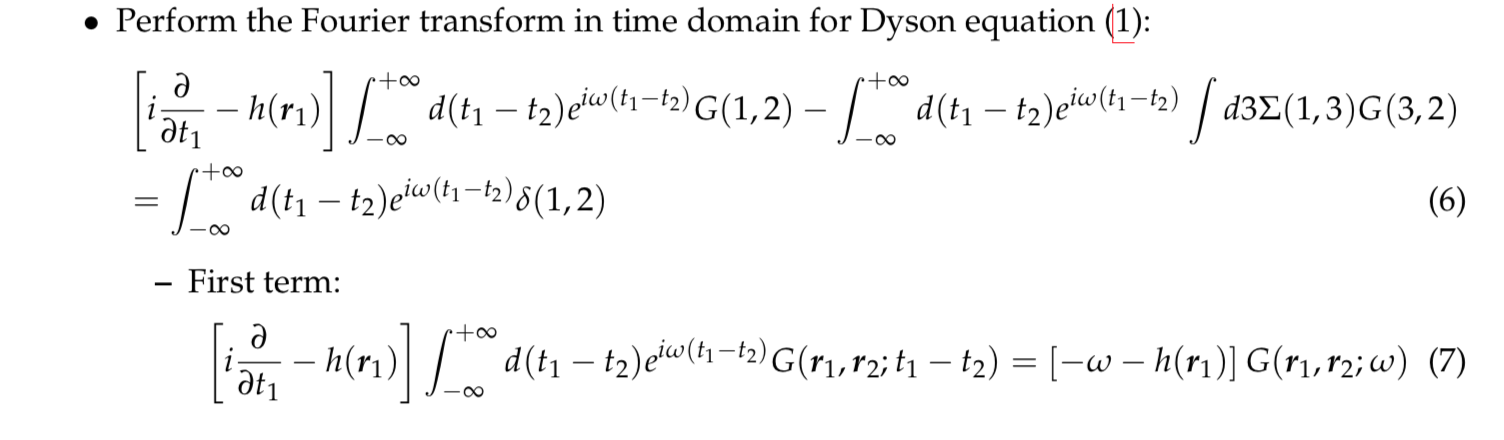
Dyson equation for zero temperature Green's function: \begin{equation} \left[ i\dfrac{\partial}{\partial t_1} - h(\vec{r}_1) \right] G(1,2)-\int d3 \Sigma(1,3)G(3,2)=\delta(1,2) \tag{1} \end{equation} in which \begin{equation*} h(\vec{r}_1)\equiv-\dfrac{\nabla^2}{2}+V_H(\vec{r}_1)+V_{ext}(\vec{r}_1) \qquad 1\equiv(\vec{r}_1,t_1) \end{equation*}
Take Fourier transformation to the energy domain: \begin{align} \left[ -\omega - h(\vec{r}_1) \right] G(\vec{r}_1,\vec{r}_2;\omega)-\int d\vec{r}_3 \Sigma(\vec{r}_1,\vec{r}_3;\omega) G(\vec{r}_3,\vec{r}_2;\omega) = \delta (\vec{r}_1-\vec{r}_2) \tag{2} \end{align}
The zero temperature Green's function under quasiparticle approximation can be represented as: \begin{equation} G(\vec{r}_1,\vec{r}_2;\omega) = \sum_i \dfrac{\psi_i^{QP}(\vec{r}_1)\psi_i^{QP*}(\vec{r}_2)}{\omega-E_i^{QP}} \tag{3} \end{equation} Insert $(3)$ into (2) one can obtain the following quasiparticle equation: \begin{equation} \left[ -\dfrac{1}{2}\nabla^2+V_H(\vec{r})+V_{ext}(\vec{r}) \right] \psi_i^{QP}(\vec{r}) + \int \Sigma(\vec{r},\vec{r}';E_i^{QP})\psi_i^{QP}(\vec{r}')d\vec{r}' =E_i^{QP} \psi_i^{QP}(\vec{r}) \tag{4} \end{equation}
How can I complete the final step? I cannot build any connection between $(2)$ and $(3)$.
This problem is related to $(6)$, $(7)$ and $(8)$.
For completeness, the Fourier transform of $(1)$ is presented: