0
$\begingroup$

In R. Shankar's Principle of quantum mechanics book in the problem 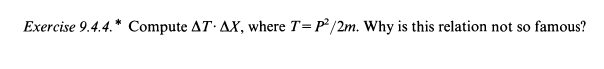9.4.4

Now $$\Delta T = \frac{-\hbar^2}{2m} \Delta( p^2)$$ And I don't arrive anywhere using this, but I also know that $\Delta A \Delta B = \left|\frac{1}{2i}\left<[A,B]\right>\right|$. So, using this I find the commutation relation \begin{align*} \frac{1}{2m}[p^2,x] &= \frac{-i p\hbar}{m}\\ \end{align*} And, $$ \Delta T \cdot \Delta X = \frac{\left<p\right>\hbar}{2m} $$ Here the author asks why this relation is not so famous. Though this seems nothing special to me. Am I donig anything wrong here?

Improve this question
$\endgroup$
2
  • $\begingroup$ is p an operator in the final expression? Or an expectation value? Perhaps it is not so famous because T is not a partner variable to x. These relations compare uncertainty between canonical variables and their "momentum" as derived from Largrange-Hamilton formalism and T is simply not related to x in this manner. One can use [p, x] to derive uncertainty relations between f(p) and g(x) so why treat these as special? $\endgroup$
    – user196418
    Commented May 28, 2019 at 11:19
  • $\begingroup$ Closely related: physics.stackexchange.com/questions/137750/… and physics.stackexchange.com/questions/481484/…. $\endgroup$
    – user87745
    Commented May 28, 2019 at 11:54

1 Answer 1

Reset to default
0
$\begingroup$

The relation will give you $$[x, T] = \frac{i \hbar}{m} p$$

Now, $\Delta T \Delta X \geq \dfrac{\langle p \rangle \hbar}{2m}$. Notice that for a state with zero momentum, the product of uncertainties can have the minimum value zero, unlike the case for any canonically conjugate pair (like momentum, position).

Improve this answer
$\endgroup$
4
  • $\begingroup$ To be sure, there wouldn't be a physical (normalizable) state where this uncertainty vanishes, right? Just that you can make it as close to zero as you want with a state more and more cleverly. $\endgroup$
    – user87745
    Commented May 28, 2019 at 12:00
  • 1
    $\begingroup$ In principle, an eigne state gives zero uncertainty, the standard deviation for the corresponding operator is zero. Not sure if it is possible to prepare in reality. Is that what you wanted to point out @FeynmansOutforGrumpyCat ? $\endgroup$
    – Galilean
    Commented May 28, 2019 at 12:08
  • 1
    $\begingroup$ @Galilean Yes, precisely. An eigenstate of the momentum operator is not normalizable (and thus, cannot be realized experimentally even in theory). $\endgroup$
    – user87745
    Commented May 28, 2019 at 12:13
  • $\begingroup$ Ah, one more comment. The state that saturates the uncertainty bound of vanishing uncertainty would be the eigenstate of the momentum operator. This is interesting because of the fact that the uncertainty in the measurement of position for such a state would still be infinitely large. So, $\Delta T\Delta X$ is as such $0\cdot \infty$ (just like in the case of $\Delta p\Delta x$), however, since $T\sim p^2$, a systematic regularization of that product tells us that this $0\cdot \infty$ is actually $0$. $\endgroup$
    – user87745
    Commented May 28, 2019 at 12:20

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.