5
$\begingroup$

In LECTURE 5: Fluid jets from MIT's 1.63J/2.26J Advanced Fluid Dynamics equation 23 is

$$\omega^2 =\frac{\sigma}{\rho R_0^3} k R_0 \frac{I_1(k R_0)}{I_0(k R_0)}\left(1 - k^2 R_0^2 \right)$$

and is described as

Combining (20), (22) and (27) yields the dispersion relation, that indicates the dependence of the growth rate ω on the wavenumber k

when describing the instability and growth of oscillations in the shape of a cylindrical column of fluid. Here $\rho$ and $\sigma$ are the density and surface tension of the fluid.

The example is for a falling column or jet of fluid in gravity but gravity does not appear in this particular expression which makes me hope that it can apply to the problem below.

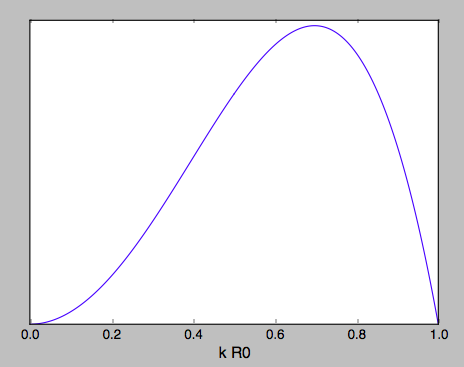
If I plot the dependence on $k R_0$ I can reproduce the maximum at about 0.7. Using $\lambda = 2 \pi / k$ I get a maximum instability growth rate at period of about 9 times the cylinder radius.

plot

The newly released Periodic Table of Videos Exploding Wires shows a variety of wires melting and then failing when heated by electrical currents. Different properties lead to different behaviors, but the molybdenum wire formed a periodic array of beads of presumably molten metal as it melted.

These beads reminded me of the Plateau–Rayleigh instability thus the math above.

I've ballpark-estimated the wire diameter to be about 6 pixels, and the bead period to be about 36 pixels, so I estimate the period to be 12 times the wire radius which nicely matches the ratio above.

Question: Is this wire-melting phenomenon likely to be a manifestation of the Plateau–Rayleigh instability? Have I used the linked lecture notes and Equation 23 in a reasonable way?

Exploding Wires, Periodic Table of Videos

Exploding Wires, Periodic Table of Videos

As a loose analogy only:

From Rayleigh-Plateau Instability: Falling Jet Analysis and Applications Oren Breslouer, MAE 559, 1/08/10, Final Project Report

water droplets on a plant stem

Improve this question
$\endgroup$
4
  • 1
    $\begingroup$ Ah dispersion relations... my current work-induced nightmare. For the melting wires, are they also aligned with a gravity field? Or are these horizontal wires, which might suggest a different mechanism for how the waves form? $\endgroup$
    – tpg2114
    Commented May 21, 2019 at 14:12
  • $\begingroup$ @tpg2114 The wire was horizontal when it melted. It's hard to imagine how it could melt and flow on the outside and remain solid on the inside. The wires were maybe 15 cm long, suspended and contacted at the ends, and all other materials tended to expand, soften, then droop and break at one point. Tungsten and magnesium burned. $\endgroup$
    – uhoh
    Commented May 21, 2019 at 14:26
  • 2
    $\begingroup$ I'm not sure about the structural mechanics of it, but maybe it does melt on the outer surface first and then surface tension pulls the beads together... That would be a different mechanism than the R-P instability though (I think). Interesting question! $\endgroup$
    – tpg2114
    Commented May 21, 2019 at 14:30
  • 2
    $\begingroup$ I agree with @tpg2114 - surface tension on the surface melt created the beads (kudos on the heating to be uniform along that much wire). I suspect that a combination of viscosity and surface tension results in a somewhat similar balance as for your equation, and an optimal periodicity. $\endgroup$
    – Jon Custer
    Commented May 21, 2019 at 15:00

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.