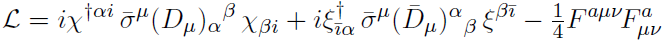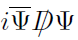2
$\begingroup$

I know the mathematical difference that one means $ absolutevalue(det) = 1$ and one means det = 1 (rotation) and that ones the subgroup of the other and so on.

But:

enter image description here

has a local/gauged $SU(3)$ colour gauge symmetry and global $U(2)\times U(2)$ flavour symmetry. This is the Weyl-field lagrangian for $u$ & $d$ quarks.

I'm confused why it should be a $U$ or a $SU$ symmetry, since both are unitary: $1=U^{\dagger} U$ makes bilinears like

enter image description here

invariant. So you should choose $U$ as the symmetry group, since its bigger. Do gauged/local symmetries have to be $SU$ and global can be $U$? Or what's the point here?

EDIT: I think that's the reason, since a local $U(N)$ transformation would need a local absolute value

$a(x)\exp(i\theta^{a}(x) t^{a})$

and then you need to use the product rule for differentiation and that makes things complicated.

Improve this question
$\endgroup$
7
  • 2
    $\begingroup$ I don't really understand the question. The $a$ index of the $F^a_{\mu\nu}$ there by definition is an SU(3) index, i.e. runs from 1 to 8. If it were a U(3) gauge theory, the index would have to run from 1 to 9, so $F_{\mu\nu}$ would need to be a different object. Also, unitary means $\lvert \det(A) \rvert = 1$, not $\det(A) \neq 0$ (The latter defines GL, not U). $\endgroup$
    – ACuriousMind ♦
    Commented May 19, 2019 at 9:30
  • $\begingroup$ its a very general and kinda vague question. not about explicit objects. of course, you need an extra generator for U(3) since its a bigger group and you have one extra parameter. $\endgroup$
    – VN23
    Commented May 19, 2019 at 9:35
  • $\begingroup$ Can you gauge the U(3) or why use the SU(3) instead? $\endgroup$
    – VN23
    Commented May 19, 2019 at 9:37
  • 4
    $\begingroup$ You seem to think that there's some deep reason for "choosing" SU over U, but we use SU(3) for color charges because the predictions of the SU(3) theory match experiment (and that of a U(3) theory do not), not for any theoretical reasons. $\endgroup$
    – ACuriousMind ♦
    Commented May 19, 2019 at 9:40
  • 1
    $\begingroup$ Related: physics.stackexchange.com/q/52452/2451 $\endgroup$
    – Qmechanic ♦
    Commented May 19, 2019 at 10:10

1 Answer 1

Reset to default
1
$\begingroup$

$U(3) \sim SU(3)_{color} * U(1)_{B-L}$ has been considered in some versions (e.g. as components of Pati-Salam's SU(4)) of Standard Model extension. If $SU(3)_{color}$ and $U(1)_{B-L}$ are considered as direct product, then they can have different coupling constants. Otherwise (if embedded in $SU(4)$) they share the same CC.

Improve this answer
$\endgroup$
0

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.