If we have a catenary line with a mass per unit length of m with one end fixed and the other end moving in sinusoidal motion like $A.sin(\omega.t)$, how would I derive the formula for the dynamic tension at the top (Assuming the line stays in one plane).
I understand the maths behind a static catenary line and also how a taut string vibration works but do not know how to start deriving for above situation.
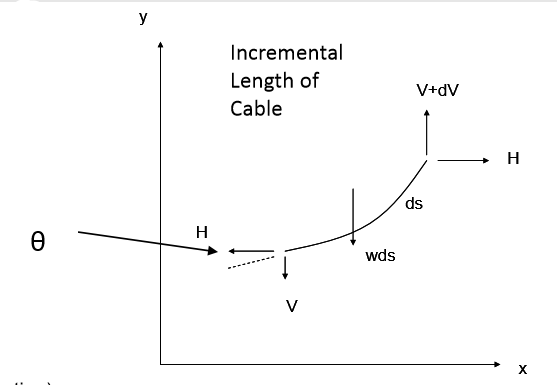
I want to start the equations as below but not sure if this is the right approach or how to proceed from here as the equations have derivative with respect to time and space both. The below image is a small segment for a static catenary line. I think for a dynamic line we need to consider the acceleration for the segment too.
$$m.ds.\ddot y + dV = mg.ds$$ $$ m.\ddot x + dH = 0 $$ $$ ds = \sqrt{dx^2 + dy^2} = dx.\sqrt{1 + (dy/dx)^2} $$
There are some finite element methods also where the line is discretized and the equations at each node are solved, but I'm only concerned about the tensions at the ends and so want to have a more analytical solution.
Any help is greatly appreciated. Thanks.