Weight and potential energy for a spring pendulum

Question

Consider a spring pendulum like in this figure

suppose the spring is arranged to lie in a straight line and its equilibrium lenght is $l$.

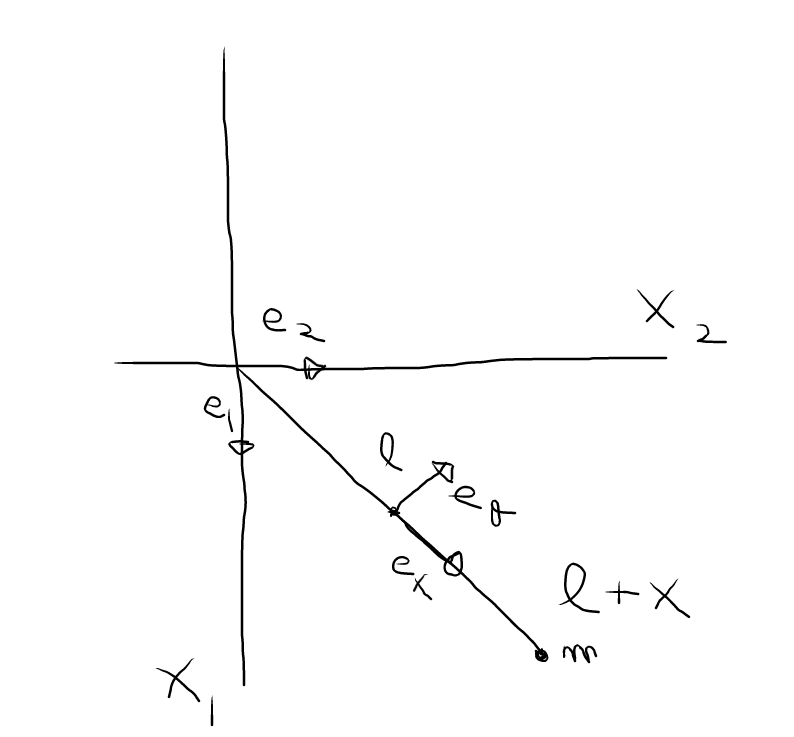
Consider the unit vectors $e_1, e_2$, $e_x, e_{\theta}$ like here

I have some trouble expressing the weight of $m$ and its potential using $e_x, e_{\theta}$.

We have $W=mge_1$ and it seems to me that $e_1 = \cos{\theta}e_x-\sin{\theta}e_{\theta}$, so $W=mg\cos{\theta}e_x-mg\sin{\theta}e_{\theta}$.

Now consider the potential (relative to the weight) $V(x_1, x_2)=-mgx_1$ with $x_1=(x+l)\cos{\theta}$.

We can also write $V(x, \theta)=-mg(x+l)\cos{\theta}$ (or at least this is what my text does) getting $P=-\partial_xVe_x-\partial_{\theta}Ve_{\theta}=mg\cos{\theta}e_x-mg(x+l)\sin{\theta}e_{\theta}$ which is in contradiction with what I wrote above.

Could you help me understand what I'm doing wrong? Thanks

Answer 1

Your definition of $\nabla$ is wrong. The correct form of $\nabla$ (in polar coordinates) is $\nabla f(r,\theta)=\partial_r f(r,\theta)e_r+{\frac{1}{r}}\partial_{\theta}f(r,\theta)e_{\theta}$ with $r=(x+l)$.

and not

$\nabla f(r,\theta)=\partial_r f(r,\theta)e_r+\partial_{\theta}f(r,\theta)e_{\theta}$.