Variance of an interacting quantum field in its vacuum state

Question

A non-interacting quantum field $\hat{\phi}(x)$ can be decomposed into $a_{\textbf{k}}$ and $a_{\textbf{k}}^\dagger$. This enables us to calculate the variance of a free field. For example, the variance of the free real scalar field, in the vacuum $|0\rangle$ of the theory, is computed to be (without a momentum cut-off) $${\rm Var}(\phi)_0=\langle0|\phi^2|0\rangle-\big(\langle0|\phi|0\rangle\big)^2\\=\int\frac{d^3k}{(2\pi)^3}\frac{1}{\sqrt{\textbf{k}^2+m^2}}\rightarrow \infty.$$ Now, consider an interacting quantum field theory described by the Hamiltonian $H$ and $|\Omega\rangle$ is the vacuum state of the interaction theory i.e. $$H|\Omega\rangle=0|\Omega\rangle\hspace{0.5cm}\big(\text{also,}~P^\mu|\Omega\rangle=0|\Omega\rangle\big).$$ Decomposing the field into creation and annihilation operators is no longer possible. So how does one compute the variance $${\rm Var}(\phi)_\Omega=\langle\Omega|\phi^2|\Omega\rangle-\big(\langle\Omega|\phi|\Omega\rangle\big)^2$$ in $\lambda\phi^4$ theory?

Answer 1

As already mentioned by other people, $\phi^2(x)$ is not really well defined as it is because there is an UV divergence in taking $x\to y$ on $\phi(x)\phi(y)$. But in QFT we can give a meaning to $\phi^2(x)$ as a composite operator. The UV divergence we encounter can be subtracted order by order in perturbation theory and obtain a finite answer in the end.

Let me assume for simplicity that the expectation value of $\phi(x)$ is zero on the vacuum. Then we need to compute $\langle \phi^2(x)\rangle$. I am assuming you are familiar with the path integral formalism. Let us define a source $L(x)$ to which the composite operator $\phi^2(x)$ is coupled. We then have a partition function $$ Z[L] = \int \mathcal{D}\phi\, \exp\left(-S[\phi] + \int \mathrm{d}^dx\,L_B(x)(\phi_B)^2(x)\right)\,, $$ where the subscript "$B$" stands for "bare". The field renormalizes with the usual wave function renormalization $\phi_B(x) = \sqrt{Z_{\phi}}\phi(x)$ and $L$ renormalizes as $L_B(x) = Z_L L(x)$. We have by definition $$ (\phi^2)_B(x) = Z_L^{-1} (\phi^2)(x)\,, $$ where I use the parentheses to distinguish between the square of the operator $\phi$ and the operator $\phi^2$. Correlators with the operator $(\phi^2)$ can be computed as $$ \langle (\phi^2)(x_1)\cdots (\phi^2)(x_n)\rangle = \frac{1}{Z[0]}\frac{\delta^n}{\delta L(x_1)\cdots \delta L(x_n)} Z[L]\,. $$ If we want to consider higher point insertions of $(\phi^2)$, by power counting we would need to add also a term $a\int L^2(x)$ and renormalize the coupling $a$, but for this case we do not care.

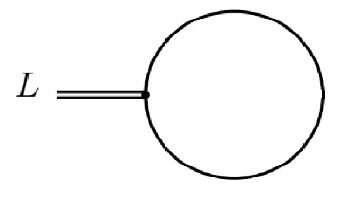
The Feynman rules are simple, just add to the rules for $S[\phi]$ a new vertex with an $L$ leg and two $\phi$ legs. The function we need $\langle (\phi^2)(x)\rangle$ is the sum of all Feynman diagrams with one external $L$ leg. At one loop in dim-reg this is

$$ (\textbf{Fig. 1})= \int \frac{\mathrm{d}^d p}{(2\pi)^d} \frac{1}{p^2+m^2} =\frac{m^4 \mu^{-2\varepsilon}}{2(4\pi)^3 \varepsilon}\left(\frac{4\pi \mu^2}{m^2}\right)^\varepsilon + (\mathrm{finite})\,. $$

You can then absorb that pole in $\varepsilon$ in the definition of $Z_L$ to obtain a finite answer in the $\mathrm{MS}$ scheme. Note that if the field is massless, this integral identically vanishes in dim-reg.

---

Fig. 1

---

$[1]$ Damiano Anselmi, Renormalization. 14B1

Answer 2

1. You don't need to compute the variance of a scalar field to see that it will always diverge: The expression $\langle \phi(x)\phi(x)\rangle$ is essentially the limit of the propagator for $y\to x$ and $\langle \phi(x)\rangle$ is constant because it's a Lorentz invariant. The propagator must diverge for $y\to x$ since otherwise it would predict a non-unit probability for a particle to propagate from event $x$ to event $x$, which would be non-sensical.

2. In the interacting theory, any attempted "computation" would have to proceed by computing the Dyson-resummed propagator to the desired accuracy and then taking the limit $y\to x$. Which, as argued above, will always diverge, so it is pointless to attempt.

3. Bonus fact: The ill-definedness/divergence of $\langle \phi(x)^2\rangle$ is a reflection of the fact that a quantum field is an operator-valued distribution and you can't square a distribution in a mathematically rigorous way.