2
$\begingroup$

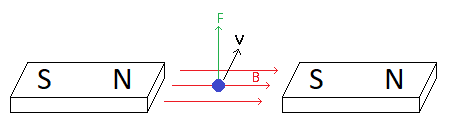
When an electron with charge $q$ travels with velocity $v$ perpendicular to a magnetic field generated between two permanent magnets with field strength $B$ and no electric field, it experiences a Lorentz force equal to $$F = qv \times B$$ The resulting change in momentum for the electron will be transferred through the magnetic field to the magnets. For example in a setup like this:

Lorentz force

the electron would experience a change in momentum upwards and the magnets would experience an equal and opposite change in momentum downwards, due to conservation of momentum.

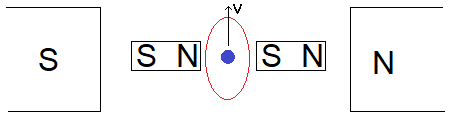
My question is, does this "reaction force" on the magnets also apply when you have a magnetic field inside a magnetic field, such that the superposition of the two fields results in no magnetic field at the electron's position. For example, lets say you have this following thought experiment with 4 magnets and a moving electron:

Electron in neutral region

Where the red oval represents zero (or essentially zero) magnetic field. The magnetic field lines pointing right from the small magnets exactly cancels out with the magnetic field lines pointing left from the big magnets. If you just had the big magnets the electron would experience a force down (into the screen), and if you just had the small magnets the electron would experience a force up (out of the screen), but these cancel out, so there is no net force on the electron.

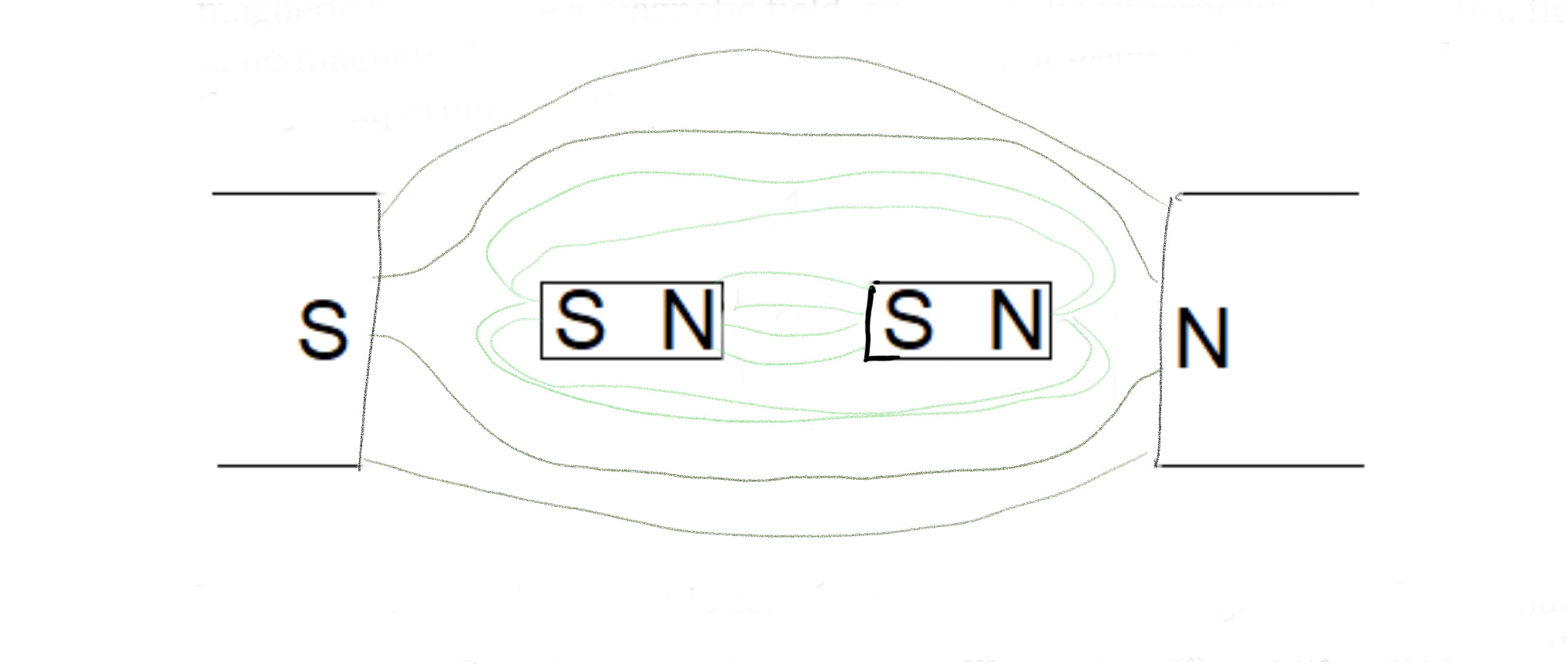
If you think there must still be a field between the two small magnets, either mentally increase the strength of the two larger magnets or mentally move the smaller magnets further apart. Here is a zoomed in visualization of the magnetic field lines to help visualize this. Sorry for the image quality:

enter image description here

This might seem counter intuitive, but it is possible because the strength of a magnetic field is proportional to the inverse cube of distance to the magnet. Imagine how a compass still points to earth's magnetic north, even if it is between 2 magnets spaced 100 meters apart.

So which of the following happens?

1) The big magnets experience a change in momentum up and the small magnets experience a change in momentum down.

2) None of the magnets experience a change in momentum. This would be the case if the experiment results in the magnets not moving.

Notes:

  • There is no violation of conservation of momentum here, in both 1 and 2, the net momentum change is 0.
  • I expect there is a knowable concrete answer (1 or 2) because this could be tested in the real world with a relatively uncomplicated experiment.
  • I am more interested in a concrete 1 or 2 and less interested in a why, but a general reasoning of why would be nice. I won't be able to follow a math explanation if it uses more than simple derivatives or integrals.
  • I tried to look for duplicate questions that might already answer this, and there are a lot of related questions, but I couldn't conclude a concrete answer to this question from them. The closest I found was this question, which might hold the math to get the answer, but unfortunately I couldn't follow all the details.
Improve this question
$\endgroup$

1 Answer 1

Reset to default
-2
$\begingroup$

The resulting change in momentum for the electron will be transferred through the magnetic field to the magnets.

What you describe never was observed. Magnets, being involved in the phenomenon of Lorentz force, don’t experience a momentum nor does their field strength weaken. The influence of the magnet is comparable to that of a catalyst in chemistry, it is not consumed. So we need a different explanation, how the Lorentz force works in detail.

Perhaps you know that the deflection of the moving electron in the magnetic field is accompanied by the emission of electromagnetic radiation and the loose of kinetic energy of the electron. A photon has a momentum and that is the reason why the moving electron gets deflected and moves in a spiral path until it’s kinetic energy is exhausted.

you have a magnetic field inside a magnetic field, such that the superposition of the two fields results in no magnetic field at the electron's position.

The magnetic field between the inner magnets still exist, even with the stronger magnets outside. Magnetic fields, imaginated by field lines, are always closed loops (going even through the source) and opposite directed magnetic fields displace each other.

enter image description here

For permanent magnets it is clear that the source of the field are the aligned (and “frozen”) magnetic dipoles of the involved subatomic particles. With a very strong magnetic field you are able to destroy the alignment of the smaller magnet, but this leads again to a resulting magnetic field in the position of your electron.

Improve this answer
$\endgroup$
8
  • 1
    $\begingroup$ I am unable to see, how the first two sentences of your answer have to be understood. First, momentum conservation is fundamental. Second, fields mediate interactions between matter. One electron will always feel the repulsive force exerted by another electron and vice versa. In such an interaction, momentum is conserved. I see the Lorentz force as the result of the relativistic transformation of the electric field seen in the electron's rest frame into the lab frame in which the magnets are at rest. I do not think that there is doubt that momentum is conserved in EM interactions. $\endgroup$
    – flaudemus
    Commented Mar 8, 2019 at 8:13
  • 1
    $\begingroup$ Concerning the observation: perhaps the effect was not observed in this particular setting, because it is very small. But we know, for example, that two parallel current carrying wires (which are otherwise charge neutral) do interact and there is a mutual force due to the magnetic field between them. $\endgroup$
    – flaudemus
    Commented Mar 8, 2019 at 8:18
  • $\begingroup$ "What you describe never was observed" I believe that is incorrect. Have you ever felt the kick back of a powerful electric drill when you turn it on in the air? This is because of conservation of angular momentum. The drill's rotational force is entirely generated by electrons travelling (through a wire) in a magnetic field and can be explained with only the Lorentz force. $\endgroup$
    – Andrew
    Commented Mar 8, 2019 at 10:07
  • $\begingroup$ "The magnetic field between the inner magnets still exist" When I say "no magnetic field" I elaborate later saying "the red oval represents zero (or essentially zero) magnetic field". This means there is a magnetic field, but the value of the field strength is zero (or essentially zero). I didn't draw the field lines because they would have cluttered an already busy diagram, but see here ece.neu.edu/fac-ece/nian/mom/img/How%20Magnets%20Work/… for an example of no magnetic field at a point between two magnets. $\endgroup$
    – Andrew
    Commented Mar 8, 2019 at 10:12
  • $\begingroup$ @Andrew Please compare your sketch with N and S against each other and the sketch from your link. $\endgroup$
    – HolgerFiedler
    Commented Mar 8, 2019 at 19:57

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.