In the PARTICLE PHYSICS BOOKLET, Extracted from the Review of Particle Physics, we read that the fine structure constant has a value $\alpha \approx 1/137$ at $Q^2=0$ and $\alpha\approx 1/128$ at $Q^2\approx m^2(W)$. What are those $Q^2$ and $m(W)$ and what does it means such difference?
$\begingroup$
$\endgroup$
3
-
2$\begingroup$ Where in that 256 page document are we supposed to be looking ? $\endgroup$– StephenG - Help UkraineCommented Feb 25, 2019 at 23:04
-
1$\begingroup$ Near duplicate. "Constant" is a historical misnomer. It is actually a "running coupling" dependent on the energy scale of the process in question, as dictated by the Renormalization Group of QFT. $\endgroup$– Cosmas ZachosCommented Feb 25, 2019 at 23:21
-
$\begingroup$ @StephenG. It is at pg. 6: the table1.1 of Physical constants. $\endgroup$– Emilio NovatiCommented Feb 26, 2019 at 21:27
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
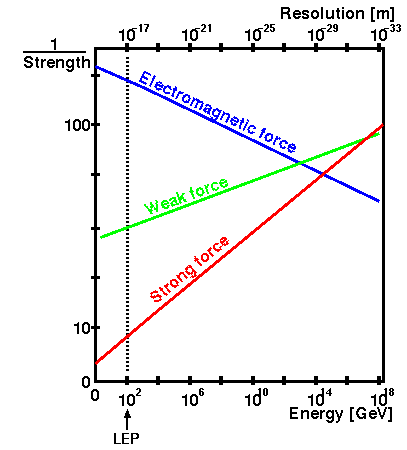
Even though they are called coupling "constants", the strong, weak and electromagnetic coupling constants all vary depending on the energy scale of the involved particles. In this case, the second value of approximately $1/128$ can be measured at the energy scale of the W boson (so around $80$ GeV).
The dependence of a coupling constant on the energy scale is described by a so-called beta function. The electromagnetic coupling strength increases with the energy scale, whereas the coupling of the strong and weak interaction decrease with the energy scale. In the image below you can see the energy scale dependence of the three coupling constants.
$\hspace{3.5cm}$ 
