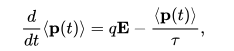0
$\begingroup$

Specifically I'm referring to the equation below which I took from wikipedia.

enter image description here

The derivation of this equation based on the Drude model seems to somewhat standard as all the resources I've looked at do it the same way: Consider the momentum an infinitesimal time (dt) after some collision at t. This would result in an equation with differentials (differentials as in dt not dp/dt for example) and after some algebraic manipulation with these differentials and application of probability theory, they would finish by neglecting a differential of small order and taking a limit from which the above equation would arise. Now I understand heuristically why these derivations are reasonable, however, I do not understand the underlying mathematics behind the differentials and the neglect of a differential term of low order. As such I am hoping someone could

a) Provide a derivation of the equation based on the Drude model with more mathematical rigour (No differentials like dt on their own only full derivatives or in integrals, and have everything well defined mathematically)

b) Provide a reference for a)

I should note, the derivation does not need to be simple, nor do I need every little bit explained, so long that everything is defined precisely I am confident that I will be able to understand it.

Thanks!

http://www.physics.iisc.ernet.in/~aveek_bid/PH208/Lecture%201%20Drude%20model.pdf

If you are not familiar with the standard derivation I am referring to, here is a link

Improve this question
$\endgroup$
4
  • $\begingroup$ Ashcroft & Mermin would disagree on the 'infinitesimal time (dt) after some collision at t' part - they explicitly consider the fact that $(1 - dt/\tau$ electrons do not collide in time $dt$. Now, why do you not understand the dropping of terms of order $(dt)^{2}$ as one takes the limit of $dt$ going to zero? $\endgroup$
    – Jon Custer
    Commented Feb 8, 2019 at 18:09
  • $\begingroup$ The main issue is that I don't understand mathematically what (dt) is. I know the description "arbitrarily small value", however, I don't understand the mathematical implications of this. I do, however, understand the rigorous definition of a limit, derivative, integral etc which is why I was hoping the derivation could be rephrased in terms of rigorous notions which I do understand. $\endgroup$
    – Mateo Duran
    Commented Feb 8, 2019 at 18:20
  • $\begingroup$ $dt$ just goes back to the limit theorms used to derive calculus in the first place. It is some short time interval, and as you take the limit as it goes to zero, throwing out higher order terms, you get the derivative. $\endgroup$
    – Jon Custer
    Commented Feb 8, 2019 at 18:27
  • $\begingroup$ Drift Velocity in the Drude Model $\endgroup$
    – Farcher
    Commented Oct 25, 2021 at 8:21

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.