1
$\begingroup$

How the angular fringe width remains constant in Young's double slit experiment when the number of fringes goes on increasing 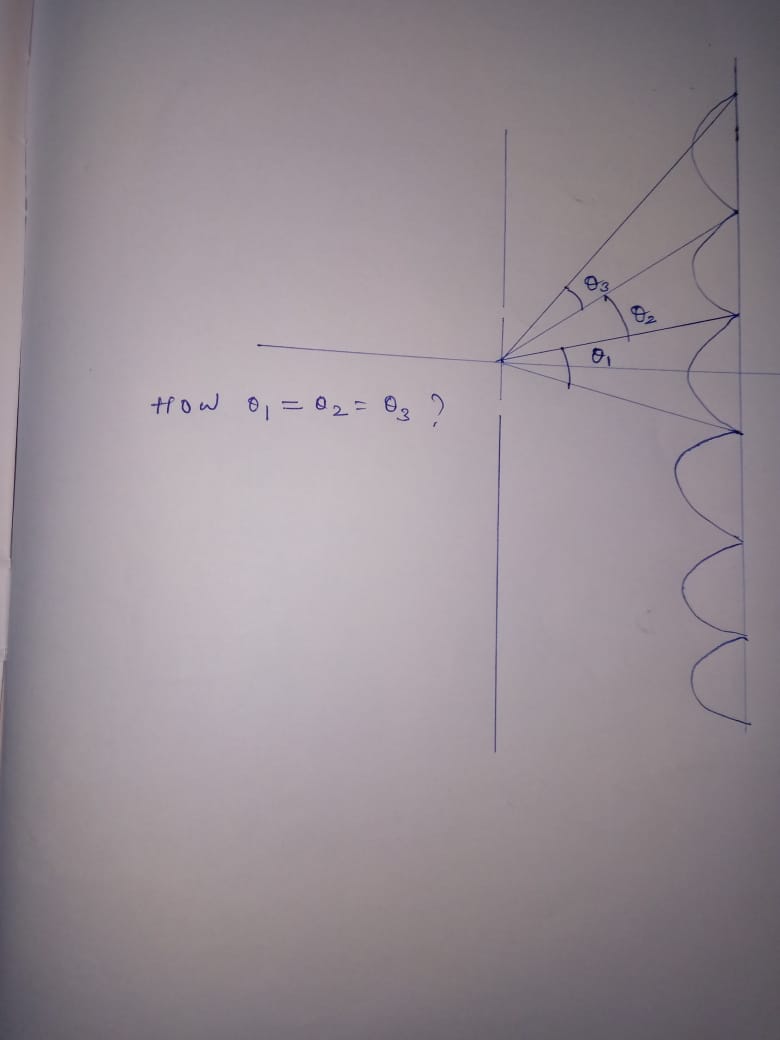enter image description hereas we move above or below the central fringe,

in the text its given angular fringe width or angular fringe separation = (λ D/d)/D = λ/d,D is the distance of central fringe from the slit,d being the size of the slit, also it states angular separation of fringes is increase in θ needed to increase the path difference by λ, as we move above the central fringe I am finding the angle(angular fringe width) goes on decreasing,how its remaining constant.

Improve this question
$\endgroup$
3
  • $\begingroup$ Usually, to compute path differences in double slit experiment, we use small angles approximation $\tan (\theta )\approx \theta $ $\endgroup$
    – Vincent Fraticelli
    Commented Jan 26, 2019 at 17:31
  • $\begingroup$ can u clarify what it means by " angular separation of fringes is increase in θ needed to increase the path difference by λ" $\endgroup$
    – sachin
    Commented Jan 26, 2019 at 18:08
  • $\begingroup$ English is not my native language and maybe I don't understand your text properly. Usually, one say that to pass from a fringe to the next one, you have to increase the path difference $\delta =\frac{ax}{D}$ (first order on x) by $\lambda $ and so $x$ has to increase by $i=\frac{\lambda D}{a}$ (distance between fringe). The angular width of a fringe is $\frac{i}{D}=\frac{\lambda }{a}$, constant. But you have to suppose that the first order development in $x$ is OK. Maybe the validity of this development is the source of your questions ? $\endgroup$
    – Vincent Fraticelli
    Commented Jan 26, 2019 at 18:20

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Browse other questions tagged or ask your own question.