0
$\begingroup$

The problem I am working on is:

"A block of mass m1 = 1.80 kg and a block of mass m2 = 6.30 kg are connected by a massless string over a pulley in the shape of a solid disk having radius R = 0.250 m and mass M = 10.0 kg. The fixed, wedge-shaped ramp makes an angle of θ = 30.0° as shown in the figure. The coefficient of kinetic friction is 0.360 for both blocks."

The provided diagram: 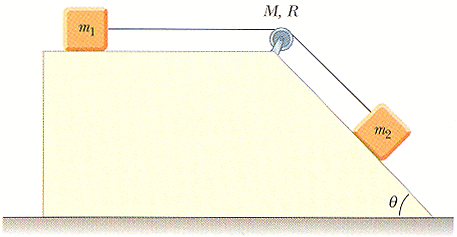enter image description here

Determine the acceleration of the two blocks. (Enter the magnitude of the acceleration.)

Determine the tensions in the string on both sides of the pulley.

What I was wondering is why there are two different tension forces acting on the pulley? Could someone give me a descriptive answer? Also, does the mass of the pulley somehow affect the tension forces? Why exactly?

Improve this question
$\endgroup$

2 Answers 2

Reset to default
3
$\begingroup$

Using the principle of virtual work, if you move the blocks a distance a, the inclined block is lowered by an amount equal to $a\sin(\theta)$, meaning that it gains energy $m_2 ga\sin(\theta)$. The total moving mass is $m_1 + m_2$, so that the acceleration is the same as for a mass $m_1 + m_2$ in 1 dimension with a force $m_2 g \sin(\theta)$, so that

$$ a = {m_2 \over m_1 + m_2} g \sin\theta $$

This is how you solve these types of problems, it's equivalent to writing the Lagrangian, but more elementary sounding.

Improve this answer
$\endgroup$
0
1
$\begingroup$

Tension is a vector, so it has different directions on either side. For second question, imagine what would happen to tension if you had a pulley with the mass of the moon.

Improve this answer
$\endgroup$
5
  • 1
    $\begingroup$ Great point. To understand questions like this it is always a good idea to think of extreme situations. $\endgroup$
    – John Alexiou
    Commented Dec 1, 2012 at 14:37
  • $\begingroup$ Oh, yes...The pulley would definitely have more rotational inertia, and that would diminish the amount of force transmitted, right? $\endgroup$
    – Mack
    Commented Dec 1, 2012 at 15:11
  • $\begingroup$ I've sort of run into a road-block trying to solve this problem. I try to solve for the acceleration, but I have no way of eliminating the unknowns from my equations; what still remains is the two tensions forces. So, I tried to figure out how they were related through torque on the pulley; and despite the fact that there is a relationship, it introduces another unknown, namely, the torque. How do I solve for the acceleration? $\endgroup$
    – Mack
    Commented Dec 1, 2012 at 15:19
  • $\begingroup$ I actually figured it out. However, I have a new question: if the tension forces on either side of the pulley are different, then why is there only one acceleration for the two blocks and the particles on the outer rim of the pulley? $\endgroup$
    – Mack
    Commented Dec 1, 2012 at 15:43
  • $\begingroup$ There is two accelerations, of the same magnitude, acceleration is a vector too. A pulley is an idealized solid contraption, where motion is restriced to circular. $\endgroup$
    – Hobo
    Commented Dec 1, 2012 at 22:06

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.