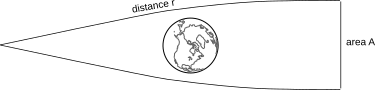1
$\begingroup$

Update: I have updated the the question, see below.

The radial excess due to the curvature of spacetime is a well-known prediction of General Relativity that is often mentioned in introductory texts, and then surprisingly (to me) never mentioned again.

Feynman's description in section II-42-3 of the Feynman lectures is this:

Suppose we were to measure the surface of the earth very carefully, and then dig a hole to the center and measure the radius. From the surface area we could calculate the predicted radius we would get from setting the area equal to $4\pi r^2$. When we compared the predicted radius with the actual radius, we would find that the actual radius exceeded the predicted radius by the amount given in Eq. (42.3). … it turns out the the earth has 1.5 millimeters more radius than it should have for its surface area.

To my knowledge there have been no experimental tests of this. This has led me to wonder whether it would be feasible to do so. The challenges I am aware of are:

  1. The large amounts of mass necessary to have a measurably meaningful effect (for a uniform density sphere of the mass of the earth the effect is predicted to be around 1.5mm). I take ‘measurably meaningful’ to mean within technically realisable limits and large enough that we are not solidly in the realm of quantum mechanics, i.e. the radial excess should be suitably larger than the de Broglie wavelength of the measured mass
  2. The difficulty in accounting for deviations from spherical symmetry
  3. The contribution from neighbouring massive bodies

The last one particularly is causing me some difficulty, as I don’t know how to quantify the effect of neighbouring bodies on the radial excess of a given object. The non-linearity of the Einstein Field Equations leads me to believe that this may be a non-trivial problem. Am I wrong? Is there a simple way to estimate the order of magnitude effect on the radial excess of a body from nearby masses?

My questions are then the above paragraph, and whether in general such a measurement is technically feasible, now or even in principle.

Edit: I have added this update to make explicit that the effect I am mentioning here is unique to General Relativity, unlike e.g. the bending of light by a gravitational source, which also occurs in purely Newtonian gravity (albeit with a different factor).

We can see this by noting that the proper distance between two points involves an integral with the metric tensor in the integrand (see e.g. the answer to this question How to calculate spatial distance in space-time?). In Newtonian gravity this would just be the Euclidean metric, and we would obtain the standard flat prediction for the radius.

It is this relativistic effect I am referring to, not the generic prediction of the bending of light. That is also why I mentioned my difficulty in understanding whether such an experiment would be feasible, since the presence of inhomogeneities would break the spherical symmetry, and thus make the Schwarzschild metric an unacceptable ansatz. Can we estimate the order of magnitude deviation from the simple result of Feynman if we have a more complicated metric?

Improve this question
$\endgroup$
4
  • $\begingroup$ While it is not as direct as you seem to have in mind the very first test that GR passed (anomalous precession of Mercury's perihelion, address in the 1915 paper) partakes of this effect. $\endgroup$
    – dmckee --- ex-moderator kitten
    Commented Dec 28, 2018 at 15:06
  • $\begingroup$ @dmckee That is interesting, could you flesh out how? In a sense I suppose any calculation involving the GR equations does involve the radial excess in some sense, but I imagine it is not always simple to see how! $\endgroup$
    – Martin C.
    Commented Dec 28, 2018 at 15:08
  • 1
    $\begingroup$ I would think that the GPS general relativity corrections would also involve the radial distance from the center of the earth . see equation 10 stanson.ch/files/GPS/Vol%2028_16.pdf . Also the satellite radius here ipgp.fr/~tarantola/Files/Professional/GPS/Ashby_2003.pdf $\endgroup$
    – anna v
    Commented Dec 28, 2018 at 17:37
  • $\begingroup$ We can see this by noting that the proper distance between two points involves an integral with the metric tensor in the integrand (see e.g. the answer to this question How to calculate spatial distance in space-time?). In Newtonian gravity this would just be the Euclidean metric, and we would obtain the standard flat prediction for the radius. I don't think this really works as a way of defining what effect you want to see. GR can be described in formalisms with and without curvature, and so can Newtonian gravity. $\endgroup$
    – user4552
    Commented Jan 1, 2019 at 23:21

1 Answer 1

Reset to default
1
$\begingroup$

Feynman's treatment is a little misleading in a couple of ways. (1) It makes it sound as if general relativity were all about spatial curvature, when in fact it's about spacetime curvature. (2) It makes it sound as if these effects were small or hard to measure.

Consider the example shown in the figure. Test particles are all released from a point, spreading out on the surface of a cone with a solid angle $\Omega$. The cone encircles the earth and goes beyond it, forming the surface of a sort of bullet or nose-cone shape. On the right-hand side, we have a circle of area $A$.

drawing of test particles deflected by earth's field

If you take $\Omega$ and $A$ and try to infer $r$, on the assumption that the figure is a Euclidean cone, you get an $r$ that is too small. The actual $r$ is greater. This is the radial excess Feynman is talking about. The effect isn't small or hard to measure. The effect is what we see in virtually any example of Newtonian gravity.

Improve this answer
$\endgroup$
6
  • 1
    $\begingroup$ but test particles released from the center of the earth would not bend like those in your example; they would fly straight, in the radial direction, wouldn't they? $\endgroup$
    – Tom B.
    Commented Dec 29, 2018 at 0:40
  • $\begingroup$ @BenCrowell I have updated the question, you may want to review your answer. I do not think your example addresses the heart of my question. $\endgroup$
    – Martin C.
    Commented Dec 31, 2018 at 9:20
  • $\begingroup$ @TomB.: but test particles released from the center of the earth would not bend like those in your example; they would fly straight, in the radial direction, wouldn't they? The lines in the diagram are straight. They're geodesics, which is the definition of straightness. They look curved in this representation only because of the way we visualize the two-dimensional projection of the four-dimensional spacetime. $\endgroup$
    – user4552
    Commented Jan 1, 2019 at 23:06
  • $\begingroup$ @BenCrowell, Ah, I see, but why would one infer that the shape described by the test particles is a Euclidean cone in a test where they originate from anywhere but the center of the Earth, not to mention at speeds less than c? Indeed if the particles were released on the right side, in your example, and start off moving to the right, one might infer an r that is too big instead. $\endgroup$
    – Tom B.
    Commented Jan 2, 2019 at 3:52
  • $\begingroup$ @BenCrowell, Is there nothing special about a geodesic, like a radial, where particles of varying speeds released along it would all reach the same point on the surface? (neglecting the Earth's rotation). I can see the phenomenon in your example which would create wildly different values for A and r, but that phenomenon seems absent for radials. $\endgroup$
    – Tom B.
    Commented Jan 2, 2019 at 3:52

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.