Rolling objects released from rest: time down the ramp [closed]

Question

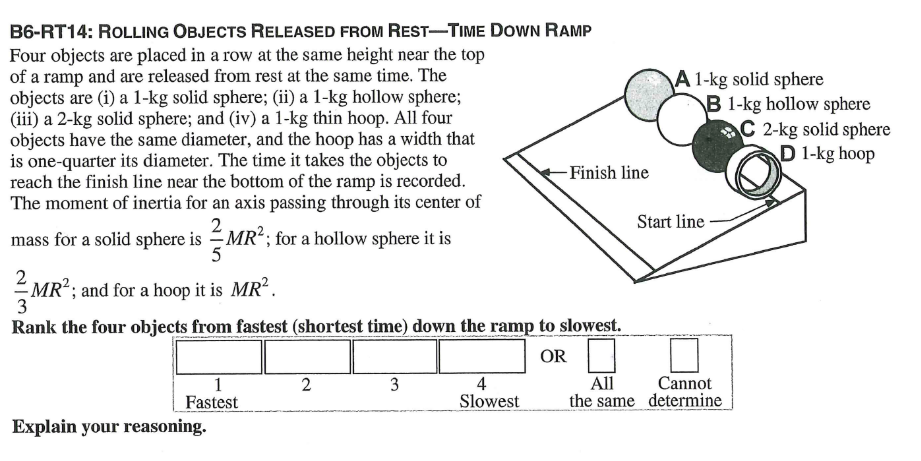
Since we are given the values of I for each object, I was able to calculate the KE's of each: the solid spheres had KE of $1/5mv^2$; the hollow sphere had a KE of $1/5 mv^2$, and the hoop had $1/2mv^2$ (for KE).

Since $KE = W = Fd = mad$, $a = KE/(md)$. Since all KE's had m's in their equations, the m's can be cancelled out. This will give us a, and since from $v^2 = v_0^2 + 2ax$ where $x$ is the same for all and $v_0 = 0$ for all (and thus v depends solely on the a). Then I had $a_D = v^2/(2d), a_B = v^2/(3d),$ and $a_A = a_C = v^2/(5d)$.

That tells me that then the order from fastest to slowest should be D > B > A = C.

The answer, however, is the exact opposite: A=C > B > D.

Could someone please help me see why?

Answer 1

Each object starts at the same height, and ends at the same height, converting its gravitational potential energy into kinetic energy. For extended objects that rotate, the kinetic energy of the object is the sum of translational (straight-line) kinetic energy and rotational kinetic energy, because it takes energy to cause an object to increase its rate of rotation. Note that it takes more energy to achieve an equal increase in the rate of rotation of an object with a large moment of inertia vs. an object with a small moment of inertia. Assuming equal masses for the objects, this means that all objects reach the bottom of the ramp with the exact same total kinetic energy, but this total kinetic energy is divided between translational kinetic energy and rotational kinetic energy. Thus, the objects with the higher rotational kinetic energy (in other words, the higher moment of inertia) will have a lower translational kinetic energy, and hence, a lower velocity at the bottom of the ramp. Note that this effect applies even if the objects in the picture have different masses.

Answer 2

Your analysis has to include change from gravitational potential energy into the translational kinetic energy of the centre of mass of the object $\frac12mv^2$ and the rotational kinetic energy of the object $\frac 12 I_{\rm C}\omega^2$ where $I_{\rm C}$ is the moment inertia about a horizontal axis through the centre of mass.
You also need to assume that the no slip condition between the linear speed and rotational speed $v=r\omega$ is satisfied.

This is an often used demonstration in a rotational dynamics course.