Work done but no change in velocity

Question

I’m taking an introductory course in thermodynamics right now and I have learned that in a quasi static process, one way to increase internal energy is to do work on a system or supply heat to it. But when you do work on a system, it increases its velocity- so how can it be in a state of equilibrium? For example, if I raise a block from one height to another height really slowly, its velocity is zero when it’s at the top because I’ve done negative work on it along with air resistance to stop it. So, how can work be done on a system without increasing its velocity such that it remains in equilibrium through every step?

Answer 1

But when you do work on a system, it increases its velocity- so how can it be in a state of equilibrium? So, how can work be done on a system without increasing its velocity such that it remains in equilibrium through every step?

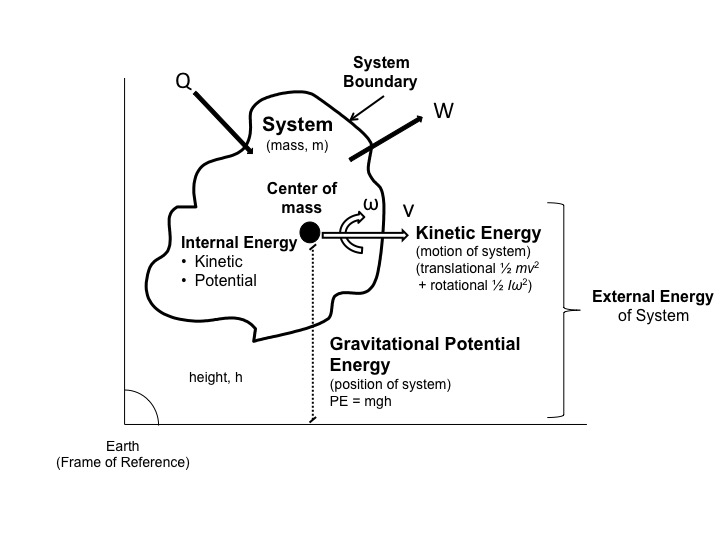
When you do work on a system it does not always increase its velocity. You need to differentiate between work done on a system that increases its internal energy versus work done on a system that increases its external energy. Refer to the figure below showing a generic system, its boundaries, and internal and external energies.

Work can be done on the system shown in the figure that, for example, compresses its boundaries. If there is less heat out of the system than work done on the system, there will be an increase in its internal energy. This does not produce a velocity of the system as a whole. An example is a system of a gas in a cylinder fitted with a piston. If the gas is compressed it increases the internal energy. This can be done quasi-statically or not.

On the other hand, if work is done on the system as a whole it can increase its velocity and therefore kinetic energy. Also possible is changing its potential energy. These are considered the systems external energy, because the kinetic and potential energy is with respect to an external frame of reference.

So when looking at both the internal and external energy, we have the following expression of the first law: $$\Delta E=Q-W=\Delta U+\Delta KE+\Delta PE$$

Where $\Delta E$ is the total energy change of the system, which is the sum of change in internal energy and change in external energy with respect to an external frame of reference.

$\Delta U$ is the change in internal energy of the system.

$\Delta KE$ is the change in kinetic energy of the system as a whole. This relates to the motion of the center of mass of the system as a whole with respect to an external frame of reference. This is different than the random translational, rotational and/or vibrational kinetic energy at the molecular level (internal energy).

$\Delta PE$ is the change in the external potential energy of the system as a whole. For example, the change in potential energy due to a change in elevation of its center of mass in a gravitational field as shown in the figure. This is analogous to considering the system in the diagram to your block example.

$Q$ is heat, which is energy transfer due solely to temperature difference, between the system and its surroundings, considered positive if transfer is to the system.

$W$ is Work. It can be work that crosses the system boundaries causing expansion or contraction of the system boundaries, or in other forms such as shaft work, electrical work that crosses the boundary. It could external work on the system as a whole that results in a changes in kinetic or potential energy.

Hope this helps.

Answer 2

When learning basic concepts in thermodynamics, the best system to examine is, by far, a dilute gas contained in a closed container whose volume can be varied, and which may or may not be thermally insulated. You should then also seek other examples, such as pushing everyday objects around, but at all times try to speak of your objects in the language of thermodynamics, which means first clarify what small set of macroscopic physical properties are sufficient to specify any given equilibrium state.

The word "quasistatic" means that a process carries the system through a sequence of thermodynamic equilibrium states. You can imagine it as a stop-start process, with each step consisting of a small change followed by time to reach equilibrium, or else a continuous but slow process, much slower than the equilibration time for your system. To do work on a gas, you would push the piston in so as to reduce the volume of the gas. Your question asserts "when you do work on a system, it increases its velocity". This is not always true. For example, when you do work on a spring, no velocity change need be involved. In the case of a gas, the centre of mass of the gas need not acquire any velocity, but work is done becaus a gas is springy owing to its pressure. If the internal energy rises, then there is a kinetic energy increase: it is the kinetic energy of the gas molecules. However, if heat meanwhile leaves the system, this internal energy may be going either up or down overall, depending on the amounts of work and heat.

Finally, in your example of raising a block in a gravitational field, suppose the force you provide is $f$ and the weight of the block is $m g$. Suppose you push with $f = mg + \epsilon$ for a time $\tau$, then you push with $f=mg$ for a longer time, enough to get most of the way to the destination, then finish wih $f=mg - \epsilon$ at the end to allow the block to slow and come to rest. The work done by your applied force is $\int_{z_0}^{z_0+h} f dz = m g h$ where $h$ is the net change in height. On the journey the velocity was $\epsilon \tau/m$. The strict quasistatic limit is the limit $\epsilon\tau \rightarrow 0$ in this example, but the example is sufficiently simple that we don't need that limit in order to get the answer for the work done. To make this thought-experiment illustrate the concept of "quasistatic" a bit more fully, one might take into account the fact that the block itself can vibrate; if it were pushed too abruptly by a sudden force then this would set up such vibrations. By taking the limit $\epsilon\tau \rightarrow 0$ one can ensure that the process will not bring in complications of that sort.

Answer 3

I would like to address a general point implied in your title. In several cases I've seen a tendency to reason about thermodynamics as if general mechanical principles were - so to say - suspended. A case in point is just your question.

Consider the classical cylinder with a movable piston. If you push the piston you're doing positive work on the system and its internal energy will increase, unless energy is also allowed to flow away through cylinder's vessels as heat. But your question would be: how can I do work on this system yet it doesn't move, except for piston (which could have negligible mass)?

The answer is that the cylinder is not floating in the air. It will be fastened to some heavy and solid body (a table, a motor) which applies to the cylinder a force opposing to yours. So it may well be that net force applied be zero, which explains the absence of overall motion. More exactly, c.o.m. of your mechanical system (cylinder + piston + contained gas) rests where it was.

Then another question may arise: how can I do work on my system if it's subjected to a zero net force? Here is that general mechanical principles come into play again. Zero net force ensures no motion of c.o.m., but tells nothing about work unless system is rigid. In this case alone (not considering rotation) zero net force implies zero work.

But your system is not rigid: a relative displacement between piston and cylinder is allowed and actually present when you push on piston. In fact, even if two opposite forces are acting, the one you apply on piston does positive work, whereas the other (e.g. acting on cylinder's bottom, which doesn't move) does no work. So total work is positive.

Another example, not related to thermodynamics but useful to understand the matter, is a spring you keep between your hands and pull at its extremes, symmetrically. The spring elongates but its center stays put. Forces applied by your hands, even if opposite, both do positive work, increasing spring's potential energy.

Answer 4

Equilibrium does not imply zero velocity at all. A quasi static process is an ideal process and will require a lot of time to complete. At every stage the block is moving with some velocity which is very small (so that Kinetic Energy tends to zero) and this velocity does not change with time (because the system is in equilibrium.)

Doing work does not imply a change in velocity. Think of pushing a big box with a constant velocity. You are doing work but still the velocity is not changing.

EDIT:-

Because a gas is present inside, due to it's pressure an opposite force (F=PA) is also present. If the Piston is located in a vacuum the the pressure force pushes the Piston out. If the Piston is on earth, then it depends on the difference in the atmospheric pressure and the pressure of the gas inside.