1
$\begingroup$

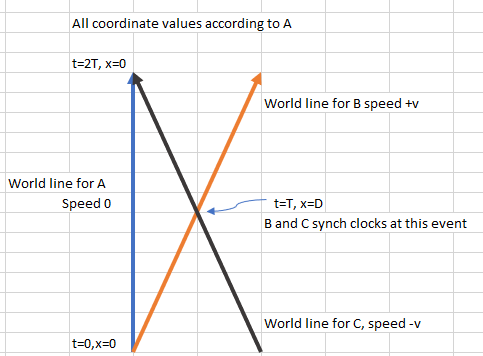
Here's a new take on the age old Twins Paradox that I have not been able to figure out.

We have two twins in two separate space ships at the same place and time. Twin A fires his thrusters for 1 year (proper time) of acceleration at 1 g, followed by 2 years of reverse acceleration at 1 g, followed by 1 year of acceleration at 1 g in the original direction, thus returning to his original position and inertial reference frame with twin B. During that time, Twin B went nowhere, but spent the time in a centrifuge, experiencing 1 g of acceleration, just like Twin A (albeit in different directions).

Both twins experienced 1 g of acceleration for 4 years (of Twin A's life), but analysis indicates that Twin B would have aged more than 4 years. Even if we treated the time in the centrifuge under 1 g as equivalent to 1 g of gravity on Earth, the gravitational time dilation is nowhere near the time dilation caused by Twin A's travel. Can someone explain where my analysis might be off?

Edit:

Just to clarify the paradox of this new scenario: Both twins experience 1 g of acceleration for the entire interval. Twin A experienced linear acceleration. Twin B experienced centrifugal acceleration. How does this only difference cause time-dilation between them?

Edit:

Sorry to have compared this scenario to the Twins Paradox, because that is not really what it is about. The answers to the Twins Paradox were accurate, but not the point.

Let's take 3 persons all staring at the same place and same time with no velocity with respect to each other. Person A experiences linear acceleration only. Person B experiences centrifugal acceleration only. Person C experiences gravitational acceleration only. There is no experiment that any of the 3 could do to determine the type of acceleration they are experiencing (in a black-box scenario). After an interval, they all return to the same position, each one having experienced the exact same magnitude (but not direction) of acceleration for the whole time. How is it that Person A experiences Lorentz time-dilation with respect to Person B, and Person C experiences gravitational time-dilation with respect to Person B? The appropriate analysis and formulas indicate that this is the case, but I would appreciate someone trying to explain the conceptual difference.

Improve this question
$\endgroup$
18
  • 2
    $\begingroup$ All of the acceleration is at the same 1g, so both twins are equally affected by gravitational time dilation, so you can ignore that part. Once you've ignored it, this is just the usual twin paradox, with the usual resolution. This question should certainly be closed. $\endgroup$
    – WillO
    Commented Sep 23, 2018 at 1:28
  • 2
    $\begingroup$ @kenshin: If you're going to allow the question "How do you resolve the twin paradox when one twin sits in a centrifuge?" then you've also got to allow the question "How do you resolve the twin paradox when one twin wears a red hat?" or "How do you resolve the twin paradox when one twin is a mezzosoprano?", and for that matter "Is momentum still conserved on holidays?" There is no end to asking the same question over and over with some new irrelevant hypothesis tacked on. I don't think it's a good idea to allow such questions. $\endgroup$
    – WillO
    Commented Sep 23, 2018 at 3:32
  • 3
    $\begingroup$ @WillO, I don't think this question is quite like those examples. The question's misunderstanding was that it is the "acceleration" that breaks the symmetry in the twin paradox, and thus posits what happens if both twins undergo acceleration. This is a perfectly logical question to ask, and happens to have a simple solution. $\endgroup$
    – Kenshin
    Commented Sep 23, 2018 at 3:52
  • 2
    $\begingroup$ @kenshin: Yes, but it's also perfectly logical to think that color of one's hat breaks the symmetry in the twin paradox. The question is not whether it's logical, but whether it's a reasonable thing for the questioner to have thought about before posting. There's room for disagreement about where to draw the dividing line, but to me it seems that a) a very small amount of noodling around with equations will dispose of this misconception, b) it's reasonable to expect the OP to have done that noodling around, and in any event (CONTINUED), $\endgroup$
    – WillO
    Commented Sep 23, 2018 at 4:18
  • 2
    $\begingroup$ (CONTINUED) c) if you take a few minutes to read through any one of the six kajillion good explanations of the twin paradox that are already on this site, it's crystal clear that they're not going to change if you put a twin in a centrifuge. I very much think that before asking a question like this, the OP should have read one of those answers, stuck in his centrifuge, and looked to see if it changed anything. $\endgroup$
    – WillO
    Commented Sep 23, 2018 at 4:20

2 Answers 2

Reset to default
2
$\begingroup$

The traveling twin ages less, and the acceleration doesn't resolve the paradox. In the standard version, the paradox is resolved when the traveling twin (who's name is traditionally "B") turns around. This turn around can take $\epsilon \rightarrow 0\ $ seconds in the Earth frame, so it cannot account for "lost" time. The change in the definition of the hyper-plane of simultaneity at the turn around event resolves the paradox--so that each twin can observed the other's clock going slower for the duration of the journey (which is a paradox with or without different total aging).

Improve this answer
$\endgroup$
1
  • $\begingroup$ That very change in the definition of simultaneity is caused by acceleration. And no matter how small $\epsilon$ can be made, the change in velocity $\Delta v$ is finite and fixed. So I agree with your explanation, but not with "acceleration doesn't resolve the paradox". $\endgroup$
    – Prof. Legolasov
    Commented Sep 23, 2018 at 21:30
2
$\begingroup$

The twin paradox is purely special relativity. You don't need the general relativity tag.

The twin paradox can be resolved without acceleration by using three or more observers.

enter image description here

The first one, A, sits at home with no acceleration. The second, B, starts out at t=0, x=0, right next to A, with speed v. The third, C, starts out at x=D, t=T, speed negative v as observed by A. C is arranged such that he passes B at the point we will call the "turn around."

So you've got a little triangle with three observers. It's possible to synchronize the clocks of B and C at the point they pass. Then you wait for C to return to A. Then you can compare the clock of A and C when they just pass.

Then by symmetry, the time B observes from being beside a to beside C has to be the same as the time C observes from being beside B to beside A.

Then you can have comparison of clocks only at the points two observers are at the same location. And you can do it with no acceleration. You don't have to worry about confusion over synchronizing clocks at a distance.

No acceleration. No synchronization except for clocks right beside each other. You will still get the Lorentz transform.

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ Thanks for this explanation. To remove acceleration completely makes it a much simpler scenario and puts it completely in the realm of Special Relativity. $\endgroup$
    – Stuart Van Horne
    Commented Sep 25, 2018 at 1:01
  • $\begingroup$ @StuartVanHorne I agree that GR isn't needed in the twin paradox but I don't think this scenario does anything to prove that. If the GR explanation were valid, it would still apply in the limit as $t \rightarrow 0$ and $a \rightarrow \infty$ at the turnaround (and note that $t$ could only ever approach $0$; it couldn't reach it because then there would be a discontinuity in the traveling twin's perception of his sibling's age). But there are other good arguments (including experimental evidence) that acceleration doesn't directly cause time dilation. $\endgroup$
    – Gumby The Green
    Commented Mar 25, 2022 at 6:45

Not the answer you're looking for? Browse other questions tagged or ask your own question.