1
$\begingroup$

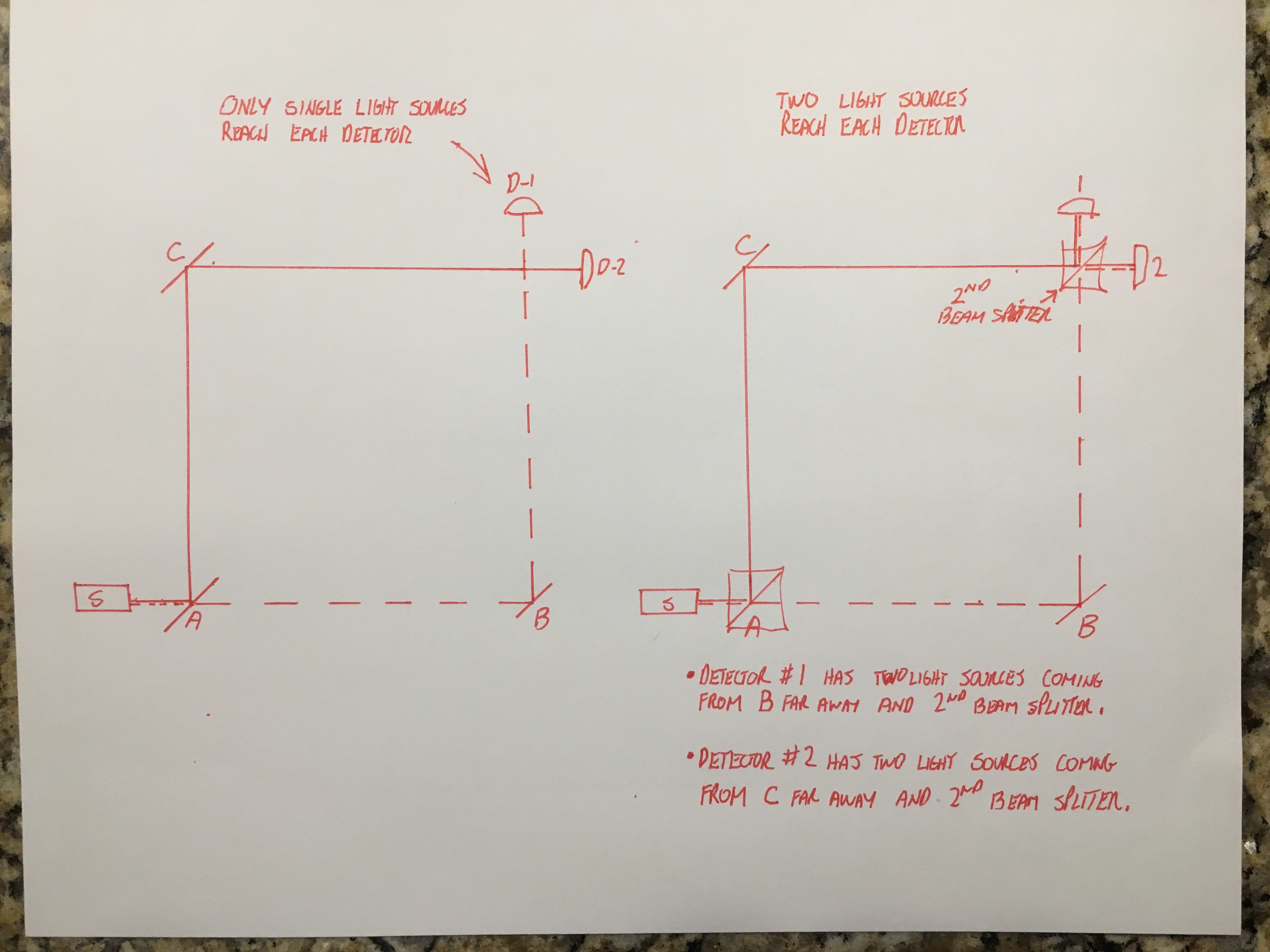
I have read many articles about the quantum eraser experiment and I am still trying to figure out why we need it. Quantum eraser

In this sketch I show the experiment with and without the second beam splitter. What I can't figure out is why we wouldn't expect interference from the setup on the right which includes a 2nd beam splitter. In that setup each detector is receiving photons from two separate sources. Detector 1 receives photons reflected from the 2nd splitter and photons reflected from a farther radius point at mirror B. Detector 2 receives photons reflected from the 2nd beam splitter and photons reflecting from from a farther radius point at mirror C. There should be interference at either detector. In the first setup (the one on the left), each detector only receives photons from one source so there should not be interference. What am I missing?

Improve this question
$\endgroup$
3
  • $\begingroup$ What do you mean by "need an experiment"? $\endgroup$
    – Luke
    Commented Sep 14, 2018 at 9:43
  • 2
    $\begingroup$ Your description what happens in the depicted experiments is correct. Interference happens in the right picture. So what is the question? $\endgroup$
    – Luke
    Commented Sep 14, 2018 at 9:44
  • $\begingroup$ The answer below says my experiment is set up wrong and you say it is correct. Which is it? I don’t understand what’s mysterious about the experiment because they should be interference whentwo beams meet at one detector. $\endgroup$
    – Bill Alsept
    Commented Sep 14, 2018 at 15:22

2 Answers 2

Reset to default
2
$\begingroup$

The setup you have drawn shows no 'eraser' part of the quantum eraser experiment. One way to add an eraser is using polarizing elements in the Mach-Zehnder setup you have drawn on the right.

Try reading this article :

http://www2.optics.rochester.edu/workgroups/lukishova/QuantumOpticsLab/homepage/snyderlapuma.pdf

To answer why we need the quantum eraser experiment, it is more a proof of principle to show that an interference pattern is destroyed if we have 'which-path' information. (This is Neils Bohr's complementarity principle which states one cannot examine both particle and wave properties simultaneously. Here, the 'particle' property = 'which path' information and 'wave' property = interference). You can find a detailed description in the link above.

Improve this answer
$\endgroup$
3
  • $\begingroup$ The second splitter erases all the which way information. They say that is why we get interference. I’m saying we get interference because there’s two beams hitting each detector when the 2nd splitter is in place. When you take away the 2nd splitter only one beam hits each detector, so there should not be interference then. It has absolutely nothing to do with knowing or not. That’s what I don’t get. $\endgroup$
    – Bill Alsept
    Commented Sep 14, 2018 at 15:32
  • $\begingroup$ Did you read the linked article? The two setups you sketched have nothing to do with quantum erasers. $\endgroup$
    – Jasper
    Commented Sep 14, 2018 at 15:50
  • $\begingroup$ @Jasper thanks I will read the article later tonight. I have read other quantum eraser articles that do not have polarizers in the set up so I’m not sure what you’re saying. $\endgroup$
    – Bill Alsept
    Commented Sep 14, 2018 at 16:30
1
$\begingroup$

We need quantum eraser experiments as experimental evidence for the complementarity of interference and which-path information for quantum objects.

For classical waves (sound, light at sufficient intensities), we get interference whenever two waves overlap. This is what happens in the experiment on the right of your sketch. On the left, there can't be any interference because the waves don't overlap at the location where your detector is.

The following experiment is done with a laser. The important thing is that it works exactly the same with a single photon source or for quantum objects with mass, for example electrons.

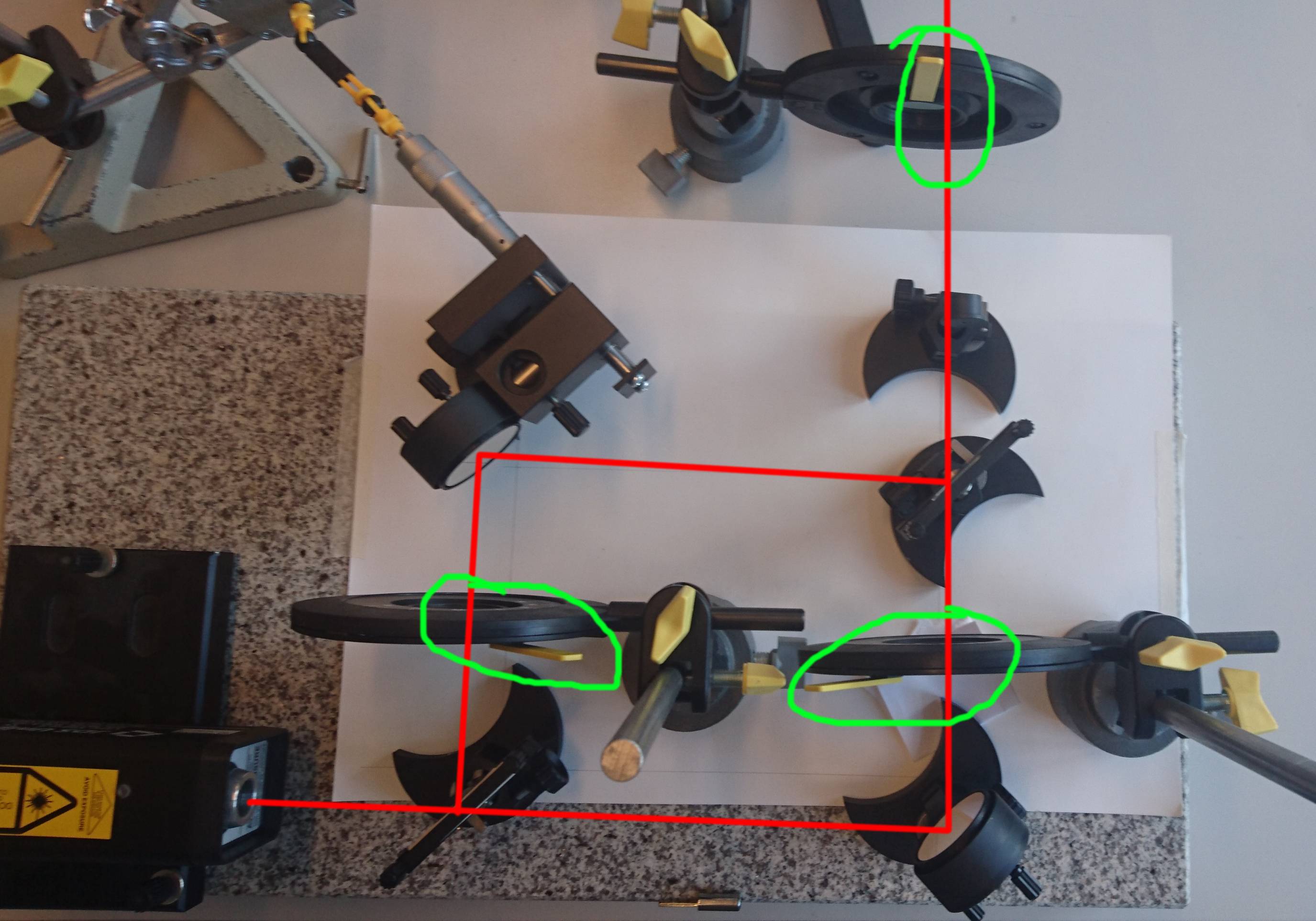
mach zehnder interferometer with polarisators and quantum eraser

The red lines show the light path, the setup is the same as in your right sketch, the screen/detector is at the top.

The parts circled in green are polarizers.

  1. Without any polarizers you get interference because the light travels both paths and they are combined again by the second beam splitter. The same happens for single photons. They "must have taken both ways" to produce interference, but this statement has to be put in huge quotation marks.
  2. When you put in the two polarizers at the bottom and set their polarization angles perpendicular to each other, the interference disappears. This can easily explained for light as electromagnetical wave because perpendicular fields can't interfere. The first funny part is that the same happens if you send single photons through the interferometer. This can be explained by the fact that you can have either interference or which-path information. With the two polarizers in place, you can tell at the detector which path a photon has taken, but you can't get interference. If the angle of polarization is NE-SW, the photon took the upper path, for NW-SE-polarization, the photon must come from the bottom path.
  3. It gets even funnier when you also put the third polarizer in place. That is the quantum eraser. Before the polarizer, you have which-path information and you can tell which path the photon has taken. After the polarizer, all photons are polarized along N-S, so you can no longer tell which path was taken. And you also get interference.
Improve this answer
$\endgroup$
15
  • $\begingroup$ @hsyed OK, I have reevaluated the experiment with polarizers and now understand the eraser effect of the third polarizer. I am still confused as to what the mystery is? What else should we expect?? Everyone talks about duality, but enough emphasis is ever given to the particle aspect of light. Consider photons with properties of at least polarization, phase and coherency. For interference to be noticed, coherent photons from separated sources need to impact the same area of the detection screen and be polarized parallel to each other. All vertical or all horizontal etc. $\endgroup$
    – Bill Alsept
    Commented Sep 17, 2018 at 1:51
  • $\begingroup$ Let’s follow photons as they travel either arm of the experiment without the third polarizer. LOWER ARM Unpolarized photons enter the first splitter. 50% still unpolarized are transmitted straight through to the lower arm toward the mirror. Photons are reflected up at the mirror and become vertically polarized (parallel to the reflecting surface) during this process. These polarized photons travel to the polarizer where 50% of them are close enough aligned so they can enter and become fully polarized in the NW-SE direction. $\endgroup$
    – Bill Alsept
    Commented Sep 17, 2018 at 1:55
  • $\begingroup$ These new polarized photons enter the second splitter where 50% are reflected to the right and 50 % continue on up where they impact the screen still polarized in the 45 degree or (NW-SE) angle. These photons are coherent and radiate from way back at the surface of the mirror. $\endgroup$
    – Bill Alsept
    Commented Sep 17, 2018 at 1:55
  • $\begingroup$ UPPER ARM Unpolarized photons enter the first beam splitter. 50% are reflected up becoming vertically polarized and travel to the polarizer where 50% are close enough aligned so they can enter and become fully polarized in the NE-SW direction. These polarized photons reflect off the upper mirror (becoming vertically polarized again and continue toward the second beam splitter. $\endgroup$
    – Bill Alsept
    Commented Sep 17, 2018 at 1:56
  • $\begingroup$ The photons enter the second splitter where 50% are transmitted through and 50% are reflected up toward the detection screen, staying vertically polarized. These photons are coherent and radiate from the second beam splitter (or the last reflecting surface) Notice this radiating point is shorter than the other arms radiating point. This geometric difference along with frequency derives the spacing of the fringe pattern. $\endgroup$
    – Bill Alsept
    Commented Sep 17, 2018 at 1:56

Not the answer you're looking for? Browse other questions tagged or ask your own question.