Earthing the plates (one or both) of a parallel plate capacitor — How can one make use of the fact that the potential of the plate is zero? [closed]

Question

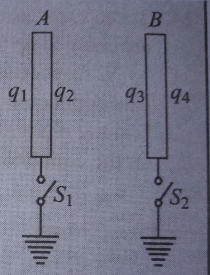
Please take a look at this question :

In the figure, plate $A$ has $100 \times 10^{-6}$ C charge, while plate $B$ has $60 \times 10^{-6}$ C charge. Find the values of $q_{1}, q_{2}, q_{3}, q_{4}$ when

1. Both switches are open (no doubts here)

2. Only switch $S_{1}$ is closed

3. Switch $S_{2}$ is also closed / both switches are open

The answers provided are (all values in $10^{-6}$ C)

1. (This one is easy) $q_1 = 80, q_2 = 20, q_3 = -20, q_4 = 80$

2. (This one is a doubt) $q_1 = 0, q_2 = -60, q_3 = 60, q_4 = 0$

3. (This one is also a doubt) $q_1 = q_2 = q_3 = q_4 = 0$

My approach for such problems

Basically, there are three things at work here.

1. Guass's law helps us to show that the charges on the inner/facing surfaces (2 & 3) will be equal in magnitude and opposite in sign.

2. We know that the electric field inside the plate (assuming that it has some thickness), a conductor, is zero under electrostatic conditions. This helps us to show that the charge on the outer surface of a plate will be equal to the algebraic sum of the rest of the charges, irrespective of whether or not the plate is earthed.

3. Thirdly, and finally, when a plate is earthed, its potential becomes zero.

I know that the third point is an important clue to solve such problems, but I'm having trouble making use of this clue.

Also, notice the pattern in the answers key — whenever a plate is earthed, the charge on its outer surface becomes zero — I've seen this in Manny problems. Why is this so? I know that in colloquial language, it is easy to say that the earth just neutralises the excess charge on the outer surface of the plate. But, I can't understand it terms of something concrete, like Guass's law. Also, if this is indeed the case, why wouldn't the earth neutralise all of the charge on the plate?

Answer 1

The really important thing about ground isn't that it has potential 0: we can define the zero of the potential scale to be wherever we want. The really important thing about it is that it can give or accept large amounts of charge while remaining at more or less the same potential. (You can think of it as a really gigantic capacitor.) For an ordinary capacitor, $\frac{dV}{dq} = \frac{1}{C}$. For ground, $\frac{dV}{dq} \approx 0$.

The pattern where a plate connected to ground has no charge on its outside surface is because of this property of ground.

When there is an electric field in a region of space, there is energy stored in that space with an energy density proportional to the square of that electric field. That is the reason why like charges want to spread out: they want to reduce the strength of the field they produce.

When a plate is not connected to ground, charge collects on its outside surface. This charge produces an electric field that fills space. When it is connected to ground, however, a new lower energy configuration becomes available: The charges that formerly lived on the outside surface can flow to ground at a very low energy cost. And the energy gain is quite large: all the energy that used to be stored in the electric field around the plate has been released.

You should be able to solve many problems of this type by keeping the following three principles in mind:

1) The energy density of the electric field at a point in space is proportional to the square of the electric field at that point in space.

2) The actual charge distribution will generally be the one for which the total amount of energy stored in the electric field is minimized.

3) A plate connected to ground can take on whatever charge is most convenient for minimizing the energy in the electric field.

Answer 2

Understand 2nd part of the question in step wise manner

• Plate A is connected to the ground (at lower potential) so all the charge on it tend to go to the ground.

• Intermediate condition - Plate A is neutral, but Plate B has charge 60 x 10^-6 C, so it induces -60 x 10^-6 C charge on inner side(2) of plate A and 60 x 10^-6 C charge on outer side(1) of plate A.

• Now, charge on outer side(1) of plate A have nothing to hold it there, so it again flows down to the ground.

In 3rd part of the question, the system formed would be similar to the system where the two plates of given capacitor are connected through a wire. Only difference is that the conductor(ground) here is at a lower potential than both the plates, so all charges flow to the ground and don't go to the other plate.