5
$\begingroup$

Can anyone properly tell me the difference between the two, and which one historically came first.

A majority of sources directly connect "emf" to flux but the 'flux rule' isn't absolute. It isn't universal. It has been generally interpret as flux creates emf. But it seems more natural to me to say that a changing magnetic field produces an electric field (everywhere in the universe however small) whose curl is nothing but change in flux.

Is it true that changing magnetic fields creates an electric field? (that need to be in a conductor. )

Griffiths' is the book which gave me this conclusion but everywhere else I have looked so far (Irodov, The Feynman Lectures, University Physics (by Zemansky) and even the MIT online lecture on induction by Walter Lewin) have said otherwise.

Improve this question
$\endgroup$
3
  • $\begingroup$ Incase both interpretations are correct then can you derive each of them from the other? $\endgroup$
    – Debaditya
    Commented Jul 22, 2018 at 18:01
  • $\begingroup$ The Maxwell-Faraday law states that a time varying magnetic field implies an electric field. This requires no conductor or any medium. $\endgroup$
    – my2cts
    Commented Jul 22, 2018 at 18:30
  • $\begingroup$ You can think.about maxwell.displacement current relation,where.time varying magnetic field.produces electric field $\endgroup$
    – आर्यभट्ट
    Commented Sep 13, 2019 at 9:42

2 Answers 2

Reset to default
5
$\begingroup$

(a) As far as I know, Faraday's law in electromagnetism is another name for Faraday's law of induction.

(b) You refer to the equation $$\vec{\text{curl}}\ \vec{E}=-\frac {d \vec{B}}{dt}.$$ Applying Stokes's theorem (and taking the differentiation outside the integral sign) this integrates to $$\oint \vec{E}.d\vec{s}=-\frac{d}{dt} \int_S \vec{B}.\vec {dS}$$ The left hand side is the line integral of the electric field, that is the induced emf, in a closed loop enclosing an area S, and the right hand side is the rate of change of magnetic flux through S, so we have $$\text{emf in loop = –rate of change of flux through loop.}$$ So the difference implied in your second paragraph between the curl equation and "emf = – rate of change of flux" isn't a difference at all!

(d) All this is valid whether or not there is a conductor.

The betatron is a particle accelerator that can be understood in terms of an induced emf in a non-conducting toroidal chamber. You could argue, I suppose, that the presence of electrons being accelerated in the chamber makes it a conductor! But it would be weird, wouldn't it, for the emf suddenly to appear when electrons are injected into the chamber?

(e) The equation "emf = – rate of change of flux" can, though, also be applied to a moving conducting loop cutting flux, though this time the emf arises from magnetic Lorentz forces driving charge carriers around the loop.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Is it correct to talk about emf outside conductors? Point e) Then why do people generally avoid ackknowleding that fact? They directly conclude that changing flux produces a field. Regardless of the phenomenon involved which are two completely different ideas. $\endgroup$
    – Debaditya
    Commented Jul 23, 2018 at 1:19
  • $\begingroup$ I believe that it IS correct to talk about an emf in an imaginary loop outside a conductor, but you don't come across the idea very often, as emfs in conductors are more useful! $\endgroup$
    – Philip Wood
    Commented Jul 23, 2018 at 7:00
  • $\begingroup$ I've added to (d) in my answer... $\endgroup$
    – Philip Wood
    Commented Jul 27, 2018 at 11:34
-6
$\begingroup$

The Faraday’s law of induction is actually not true.

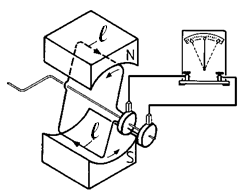
One of the fundamental laws of electromagnetism is the “Faraday's law of induction”. This law states that the induced voltage in a wire loop is equal to the speed of change of the magnetic flux enclosed with the loop, or V=dΦ/dt. In the textbooks is often given an example of a loop in the shape of a rectangle which rotates in a magnetic field.

enter image description here

What is meant by “the speed of change of the magnetic flux enclosed with the loop”?

To explain this, we will make a comparison. If we hold a ring in front of our eyes as if we want to see through it, then it has a shape of a circle. If we turn it 90°, we only see a line. In every other intermediate position of the ring, we see an ellipse. In the first position, the ring has the maximum area in front of our eyes; in the second, the minimum, i.e., zero. If the ring starts to rotate about its axis starting from the second position (0) and has turned 180°, then the area we see in the course of this rotation can be represented with a sine curve of half a period.

Similarly, when the wire loop is in the vertical position (image above), then the magnetic flux is zero, and when the wire loop is in the horizontal position, then the flux is maximal. This flux changes according to a sine function, too. So, when the flux is maximal, then the speed of its change is minimal, more precisely, zero, because the slope of the sine curve in this point is zero. But when the flux is minimal, then the speed of its change is maximal, because the slope of the curve in this point is maximal.

So, from the Faraday's law of induction it follows that when the wire loop is in vertical position, then the induced current in the loop is maximal; and when it is in horizontal position, then the current in the loop is zero.

I claim that just the opposite is true, because it is not relevant the speed of change of the magnetic flux through the loop, but the speed of the wire towards the magnet or away from it. In producing the current in the rectangular loop, only the two shorter sides of the loop play a role. When these sides are nearest the magnet, then their speed of moving towards or moving away from the magnet is zero, thus the current is also zero.

For better understanding, let’s take a look at this picture. The projection of the circling dot on the vertical axis behaves like a pendulum. When the projection dot is at the top or at the bottom of the vertical axis, its speed is zero. And when it is in the middle, its speed is maximal. The same concept applies also to the two mentioned sides of the wire loop. enter image description here

I claim that the concept of the contemporary physics called “magnetic flux through a surface” is an absolute misconception, something that is not founded in the reality. What real is and what relevant is to this case are two things: first, the strength of the magnetic field, and second, the speed of the conductor towards the magnet or away from it, that is, the component of this speed which is in line with the magnetic lines of force, not the component perpendicular to them, as it follows from the Faraday’s law of induction.

As a consequence of this misconception follows another, and that is the misexplanation of the working principle of synchronous generators and motors. Let’s look at this picture from a textbook called “Elektronik 1” from the following authors: Helmut Röder, Heinz Ruckriegel, Willi Schleer, Dieter Schnell, Dietmar Schmid, Werner Zieß, Heinz Häberle. The picture refers to synchronous motor, but it can also refer to synchronous generator. On the picture we see a magnet, three coils and three sine curves: black, blue and red. The black sine curve corresponds to the current of the black coil. From the picture we see that in the first position of the rotating magnet the current in the black coil is zero; in the second position, the current in that coil is maximal. enter image description here Just the opposite is actually true (this means: in the first position the current in the black coil is maximal; in the second, it is zero). And with this new explanation the torque from the coils upon the rotating magnet is the same at every moment of time, as it should be for its smooth rotation.

The other concept is contradictory, because the torque is not the same at every moment. Let’s take a look at the second position of the magnet when it is in line with the black coil (the current at this moment is at maximum)(the magnet rotates counter-clockwise). Until this moment the coil has attracted the white pole; then the pole goes to the left side of the coil; the coil still has the current in the same direction, which means that it still attracts the pole and thus acts against the direction of rotation. At the same moment (i.e., when the magnet is in line with the black coil) the blue and the red coil have equal currents in the same direction and both act on the opposite pole of the magnet. Thereby both exercise an attractive force. It follows that the red coil attracts the lower pole of the magnet in the direction of rotation and the blue coil attracts it against the direction of rotation. We see that on two places, both up and down, contradictory effects take place. When the upper pole of the magnet has passed the black coil a little bit, then of the three coils only the effect of the red one on the magnet will be in the direction of rotation, making the whole assembly impossible.

When the pole of the rotating magnet is moving towards the coil, then the coil attracts it. When the pole is exactly in line with the coil, then the current comes to zero, the magnetic field, too. Then a current flow begins in the contrary direction, the magnetic field of the coil is reversed and it begins to repel the pole of the magnet. This applies to a motor. The reverse applies to a generator.

Consider also this very well-known experiment: we move a magnet in and out of a solenoid. Instead of moving the magnet, we can move the solenoid. Is the wire of the solenoid moving perpendicular to the magnetic lines of force, or is it moving in line with them?

More about this you can find in my book which is downloadable free of charge at https://newtheories.info

P.S. I have great esteem for Michael Faraday and have searched his work to find where he has defined this law, but unsuccessfully. If someone can cite where it is, I will be very grateful to him/her. P.P.S. Please, read also these two answers:

https://www.quora.com/Is-it-possible-to-increase-the-current-of-a-power-source-via-an-induced-current/answer/Mitko-Gorgiev

https://physics.stackexchange.com/q/502784

Improve this answer
$\endgroup$
8
  • 4
    $\begingroup$ "When these sides are nearest the magnet, then their speed of moving towards or moving away from the magnet is zero, thus the current is also zero." This where you are mistaken. It is not the velocity component of the wire towards or away from the magnet that matters, but the velocity component at right angles to the magnetic field, $\vec B$. This is because the charge carriers in the wires move with the wires and experience magnetic Lorentz forces:$$F=q \vec{ v} \times \vec B.$$ It is these Lorentz forces that give rise to the induced emf. $\endgroup$
    – Philip Wood
    Commented Sep 13, 2019 at 10:25
  • $\begingroup$ That's where you and all others are mistaken. The component of the wire speed that is in line with the magnetic lines of force is crucial, not the component perpendicular to them. Please read these answers: quora.com/… quora.com/How-can-you-explain-the-right-hand-rule-conceptually/… $\endgroup$
    – Mitko Gorgiev
    Commented Sep 13, 2019 at 11:13
  • 3
    $\begingroup$ But these are $your$ answers, so they don't provide real support for your non-standard claims. I'll just add that the simplest of experiments (moving a wire (connected to a voltmeter) in the gap between the poles of a U-shaped magnet provides evidence for the standard interpretation. $\endgroup$
    – Philip Wood
    Commented Sep 13, 2019 at 11:30
  • 4
    $\begingroup$ Neither of us will benefit from further comments of mine. $\endgroup$
    – Philip Wood
    Commented Sep 13, 2019 at 11:55
  • 1
    $\begingroup$ It seems what you claim is not a new explanation of the old experiment, but a new phenomenon. So what you need to do is to carry out the experiment, as you shown in your figure, "When these sides (the two short sides) are nearest the magnet, then their speed of moving towards or moving away from the magnet is zero, thus the current is also zero". Just show us your experiment, best with video, that the current is 0 when the two sides are nearest the magnet, and maximum at 90 degree from that position. $\endgroup$
    – verdelite
    Commented Sep 13, 2019 at 21:33

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.