What exactly is happening in a laser's gain cell when the irradiance is greater than the saturation irradiance?
Also can someone offer a clear conceptual treatment of the gain-coefficient and its connection to the effective pump rate densities.
I apologize if my question is vague, I'm just having trouble seeing their connection.
Thanks
Below is a diagram of a 4-level gain medium (courtesy of wikipedia).
The pump pumps electrons into the 4th energy state, a state with more energy than the laser transition. Usually, there is a very fast decay from the transition that the electrons were pumped to, and the upper level of the laser transition. This allows electrons in the upper state of the laser transition to build up, and be greater than the number of electrons in the lower state of the laser transition. This is the population inversion. Ideally, we also want the electrons to decay quickly out of the lower state of the laser transition (to some lower state which the pump then pumps back up to a state above the upper laser transition state) to maximize the difference between the electrons in the upper energy state and the lower energy state of the laser transition.
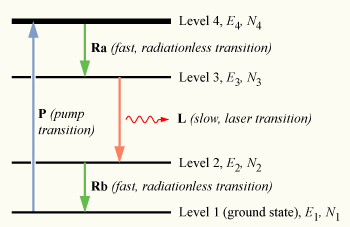
My in depth explanations of the different emission processes is contained in this answer, if you want want more info about the emission processes of the laser transition.
The rate equations can be solved with some approximations to get the following equation : $$ \gamma(I)=(N_g-N_e)\frac{\sigma_0}{1+\frac{I}{I_{\text{sat}}} + 4\left(\frac{\Delta}{\Gamma}\right)^2} $$ where $\gamma$ is the gain coefficient, $N_g$ is the initial lower or ground state (of the laser transition) population, $N_e$ is the initial excited or upper state population, $\sigma_0=\frac{3\lambda_0^2}{2\pi}$, $I$ is the irradiance, $I_{\text{sat}}$ is the saturation irradiance, $\Delta$ is the detuning (how far off resonance we are), and $\Gamma$ is the linewidth of the transition. For simplicity let's assume $\Delta=0$. Then the above equation becomes: $$ \gamma(I)=\frac{\sigma_0(N_g-N_e)}{1+\frac{I}{I_{\text{sat}}}}. $$ Now, $N_g(t)$ and $N_e(t)$ can be dependent on the irradiance $I$ as time evolves. This is key to understand what is occurring in the gain medium.
Conceptually the following steps occur to get to the saturation point:
Spontaneous emission occurs randomly, causing stimulated emission of other atoms with excited electrons.
Because of the build up of photons in the cavity causing stimulated emission of the gain medium when $N_e-N_g$ is large, the irradiance increases exponentially at first (look up Beer's law).
The above exponential build up resulted in a lot of population loss for $N_e$. At the same time there are now a lot of photons in the cavity causing stimulated emission of atoms with electrons in excited states. If the pump rate remains the same this causes $N_e-N_g$ to not be as large as it was initially. Solving the differential rate equations shows that in this regime, $I \approx I_{\text{sat}}$, the irradiance will only increase linearly.
If we ever get to a point where $I>I_{\text{sat}}$, you can conceptually think about how there will be many, many photons depleting the $N_e$ population, in fact they will deplete $N_e$ so quickly that the pump can no longer keep up.
The gain medium is like a self-damping system, the more photons (higher irradiance), the faster $N_e$ is depleted, and the fewer $N_e$ states exist for stimulated emission, so the gain in irradiance will slow down. Pumping takes time and the pumping rate is fixed, so there will exist a point at which the pump can no longer maintain a population inversion, in fact the system can dip into absorption for very short periods. The irradiance at this point will be the maximum possible.
I hope this answers your question.
