I think this explained reasonably well in Bender & Sarkar (2012). The freeze-out temperature comes about from approximate solutions of the Boltzmann equation for the time-dependence of the dark matter particle number density. This is a Ricatti equation of the form
$$ \frac{dN(x)}{dx} = \frac{-\lambda}{x^2} \left[ N(x)^{2} - N(x)_{\rm eq}^2\right],$$
where $N(x)$ is the number density of dark matter particles and $x = m/T$, where $T$ is the temperature, and $N_{\rm eq}$ is the equilibrium number density which is approximately proportional to $x^{3/2}\exp(-x)$ when $x$ is large and is equal to some constant when $x \ll 1$.
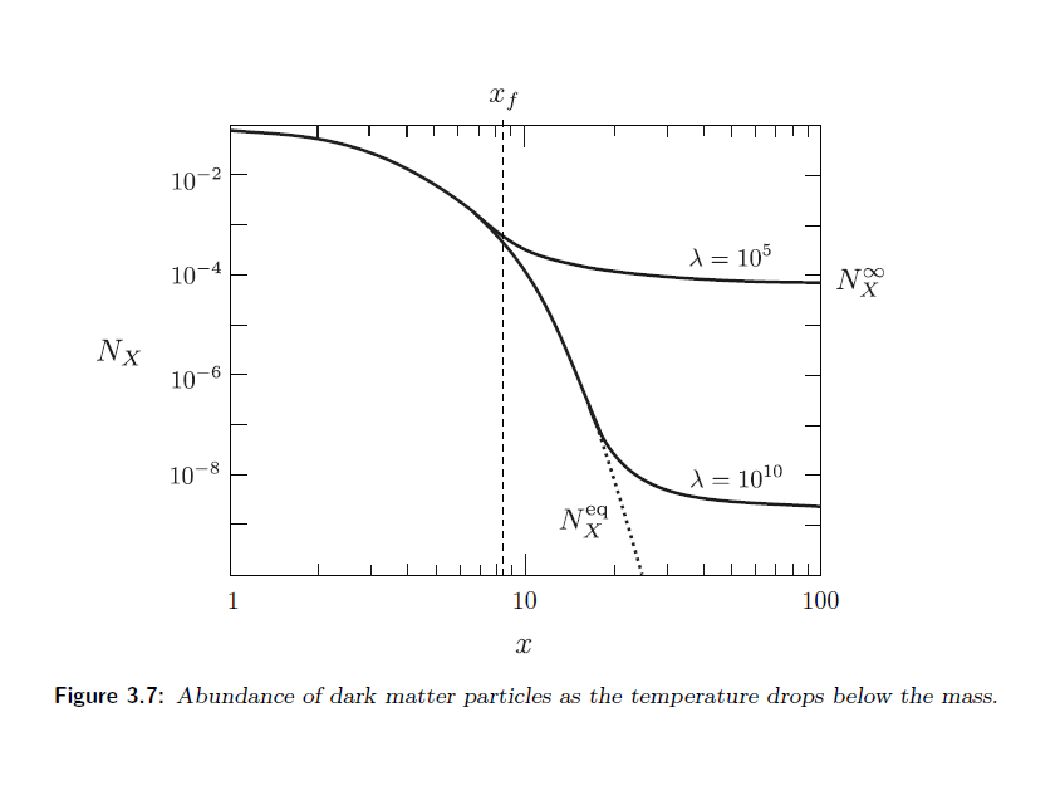
As $x$ gets larger there comes a point where the dark matter particles cannot achieve equilibrium because they do not interact sufficiently this is the freeze-out temperature. After the freeze out temperature $N(x) > N(x)_{\rm eq}$ and asymptotically trends towards the "relic density".
My understanding is that some of the complications concerning what exactly is the manner in which the dark matter interacts are hidden away in the $\lambda$ parameter, which is a dimensionless number that can be assumed to be $\gg 1$. If that is so, then the behaviour of this differential equation is that $N(x)$ departs significantly from $N(x)_{\rm eq}$ when $x \sim 20$, although the exact value does depend somewhat on the value of $\lambda$ According to the lecture notes by Daniel Baumann (from which the picture below is taken), the critical value of $x$ scales as $\ln \lambda$. This I think is the origin of the assertion in your question if $\lambda \sim 10^{10}$.