3
$\begingroup$

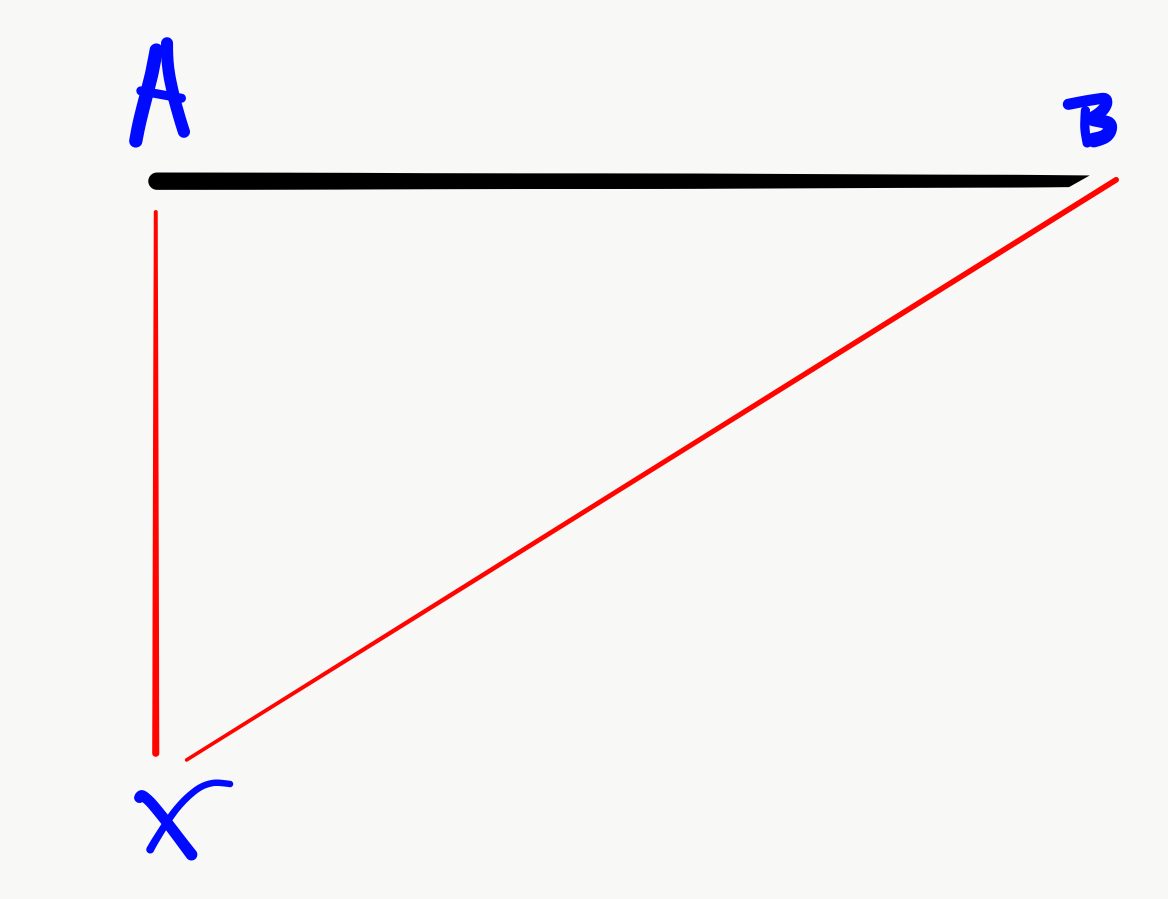
I have a question about how an observer sees the speed of light or the distances when at rest.

Suppose two events occur simultaneously in $A$ and $B$. If someone in $X$ is watching, they will see what happens first in $A$ and then in $B$ because the light will take less time to reach $A$ to $X$ than $B$ to $X$ because the distance is shorter.

Now let's say there's a light detector in $A$ and a light detector in $B$ and a flash of light coming from a midpoint between $A$ and $B$. Hopefully you'll see the $A$ detector react first and then the $B$ detector.

My doubt is: if I observe the flash from the midpoint until it reaches the detectors,

Will I see the light go faster towards $A$ than towards $B$ or will I see the shorter distance from the midpoint to $A$ than from the midpoint to $B$ so I can see the detector of $A$ react first?

Picutre

Improve this question
$\endgroup$
4
  • $\begingroup$ Your question seems flawed. X is a stationary point, and hence Lorentz factor is 1. $\endgroup$
    – QuIcKmAtHs
    Commented May 21, 2018 at 11:23
  • 1
    $\begingroup$ There's a difference between "I can see the detector of A reacts first" and "first I can see the detector of A reacts". You used the former phrase but in fact your direct observation is the latter. $\endgroup$
    – Kamil Maciorowski
    Commented May 21, 2018 at 11:50
  • 1
    $\begingroup$ I think understand what you mean, but you should make your mind a little bit first. Indeed it's a hard topic, but you must be careful when using certain words. Be sure to define who are the observerS (in plural, because one only obsevrer has no problem, this is about relativity between two observers). Think deeply and set clearly who they are. Also, the word "simultaneously" is a hard topic. Simultaneously for whom? It can only be simultaneous for one. That's the issue. $\endgroup$
    – FGSUZ
    Commented May 21, 2018 at 12:46
  • $\begingroup$ Suppose two events occur simultaneously in A and B This is a problem as simultaneity is not trivial in relativity. With relativity any problem that starts with "simultaneous" as a condition is often going to find that as the basis of the resulting paradox. $\endgroup$
    – StephenG - Help Ukraine
    Commented May 21, 2018 at 16:30

3 Answers 3

Reset to default
2
$\begingroup$

It all depends on what the meaning of the word "see" is.

The light leaves the midpoint $M$ at time $0$. It reaches both $A$ and $B$ at time $1$. If "seeing" is used in the everyday sense to mean "light from an event reaching your retina", then you see the event where the light hits $A$ at (say) time $2$ and the event where the light hits $B$ at time approximately $2.24$. In that sense you've "seen" the light travel more rapidly leftward than rightward.

But of course you can then do a quick calculation, correcting for the fact that signals take longer to reach you from $B$ than from $A$, and conclude that the two arrivals did in fact happen simultaneously at time $1$, even though the signals from those arrivals reached your eyes at times $2$ and $2.24$. In relativity, the word "see" is usually used to refer to your description of the world after you've made that correction. In that sense, you "see" both arrivals happen at the same time, so you "see" both light rays moving at the same speed.

Not everyone chooses to use the word "see" the same way, so it always pays to be clear about which meaning you have in mind. People who are talking about relativity usually, but not always, have the second meaning in mind.

This question actually has nothing to do with relativity, because there is only one observer. But if it came up in a relativity-related context, it's probably best to stick to the second definition of "see", according to which you do in fact see both arrivals simulataneously.

Improve this answer
$\endgroup$
1
  • $\begingroup$ "It all depends on what the meaning of the word "see" is." Good point. I'm sure this ambiguity misleads many newcomers to SR. I prefer to use "measure" for your second sense of "see". $\endgroup$
    – PM 2Ring
    Commented May 22, 2018 at 4:11
0
$\begingroup$

An arbitrary observer measures locally (inertial reference frame) the speed of light always at $c$. In your question the events A and B are simultaneous. What is not simultaneous are the flashes from A and B to reach X, but that is another issue. It is due to the different distances to X required to the flashes.

The simultaneity of the events in A and B is measured ideally by a set of rulers and clocks which are synchronized assuming the constancy of the speed of light in inertial reference frames, according to the second principle of SR (special relativity).

In the observer frame the speed of light is always $c$ and the distance from the midpoint to A is equal to the distance from the midpoint to B.

Improve this answer
$\endgroup$
0
$\begingroup$

It is an interesting attempt at breaking relativity, but I don't think there are any surprises. Presuming you have a series of half silvered mirrors at your disposal along the routes that do not interfere with the timing, which allow your remote ray trace to occur, then you are simply tracking triangular routes. The most direct and earliest signal is directly from M, the midpoint which goes unlabeled in the diagram. Beyond this you will certainly see the repeater A first, including the incident ray. You've asked a trick question posed about the speed of light: 'Will I see the light go faster towards A than towards B'but the logical analysis already presumes the constant speed of light whereas the geometry of the length of paths is straightforward. Still, good for you for pushing it to the limit. Keep at it and you may find the hole you are looking for. Probably the first complexity would be to put a mass to one side so that space curvature is present. Then you'll have problems.

Improve this answer
$\endgroup$

Not the answer you're looking for? Browse other questions tagged or ask your own question.