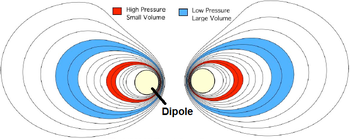
It is well known that the earth's dipole-like magnetic field is able to confine charged particles to paths that spiral along the field lines. That's why we have auroras and the Van Allen radiation belts. Could we use the same idea to confine a fusion plasma?
This isn't a new idea; several research groups have investigated similar proposals over the last few decades. Most notably, a team at MIT made a fairly serious attempt to build a dipole fusion reactor, the LDX (Levitated Dipole Experiment), but abandoned the project in 2011. There has also been some research on storing positron-electron plasmas in dipole fields. Overall, the consensus in the literature seems to be that plasma confinement in a magnetic dipole field is MHD stable. (1 2 3) I won't try to reproduce all of the results in those papers, but here's a quick summary of the main argument:
Suppose that the dipole field is created by a magnetically levitated current-carrying ring, and that the plasma in the field is in MHD equilibrium with zero pressure at the surface of the ring. (Numerical simulations confirm that an equilibrium solution exists. The pressure gradient from the interior of the plasma to the edge of the ring is opposed by the Lorentz force from the diamagnetic current.) To find out if the equilibrium is stable, we can apply the extended energy principle. If the plasma is perturbed by a small amount $\tilde{\xi}(\mathbf{r}, t)$, the total energy of the plasma changes by
\begin{align} \delta W_F = \frac{1}{2} \int_P d\mathbf{r} \left[ \frac{|\mathbf{Q_{\perp}}|^2}{\mu_0} + \frac{B^2}{2\mu_0}|\nabla \cdot \xi_{\perp} + 2\xi_{\perp} \cdot \kappa|^2 + \gamma p |\nabla \cdot \xi_{\perp}|^2 \\ - 2(\xi_{\perp} \cdot \nabla p)(\kappa \cdot \xi_{\perp}^*) - J_{\parallel}(\xi_{\perp}^* \times \mathbf{b}) \cdot \mathbf{Q}_{\perp} \right] \end{align}
where $\mathbf{Q}$ is the first-order perturbation in the magnetic field $\mathbf{B}$; $\mathbf{Q}_{\perp}$ is the component perpendicular to the $\mathbf{B}$-field; $\kappa = \mathbf{b} \cdot \nabla \mathbf{b}$ is the magnetic field curvature; $\xi ^*$ is the complex conjugate of $\xi$, which is just $\xi$ here since the perturbation is real. If the change in energy is positive, the perturbation shrinks; if it is negative, the perturbation grows.
Applying this equation to a magnetic dipole-confined plasma, we notice that the equilibrium poloidal current $J_{\parallel}$ is 0, so the last term vanishes. That leaves us with three stabilizing terms representing the energy required to bend the magnetic field lines, compress the magnetic field, and compress the plasma itself; and one destabilizing term representing the curvature drive. Thus, to ensure that our plasma is MHD stable, all we need to do is make sure that the stabilizing terms are larger than the destabilizing term. We can do this by increasing $B$; by making the ring larger (thus decreasing $\kappa$); or by reducing the pressure gradient $\nabla p$ in the most instability-prone regions of the initial equilibrium.
Since we have several degrees of freedom to play with, it's not particularly surprising that stable solutions exist with reasonable parameter values. Indeed, Garnier et al. found a specific example using numerical methods and reported it in this paper. A paper by Krasheninnikov justifies this conclusion on a theoretical basis, showing that the plasma can be stabilized against interchange and ballooning modes if certain conditions are met.
It's worth noting that many of the most troublesome instabilities that plague tokamak design do not exist in a dipole reactor. In particular, the kink and tearing modes are automatically ruled out because there is no current parallel to the B-field.
Nevertheless, despite the obvious technical advantages described above, physicists seem to have lost interest in the dipole fusion reactor. In 2011, all funding was cut to the LDX — Wikipedia says the money was redirected to tokamak research — and it appears that few or no papers have been published on the topic since that date. Assuming this decision was not made for purely political reasons, what problems with the dipole design led MIT and the rest of the fusion community to give up on it? Are there additional instabilities that I'm overlooking? Did the engineering issues (e.g. heat management, keeping the superconducting ring levitated away from the vacuum chamber walls) prove overwhelming?