I just want to confirm this, because this type of diagram seems pretty popular.
The electric field and magnetic field actually surround in all directions orthogonal to x axis, right? It is not just 2d pointing only in the y direction and z direction respectively.
This is a fairly common point of confusion. The arrows you see in that diagram express the magnitude and direction of the electric and magnetic fields on the $\hat x$ axis.
When you are introduced to vectors for the first time, they are usually represented as arrows which extend from one point to another. The vector $\langle 2, 3\rangle$ is depicted as an arrow which starts on the point $(0,0)$ and ends on the point $(2,3)$.
This is nice and intuitive, but it implies that the vector extends across physical space, which it emphatically does not. Vectors exist at isolated, individual points.
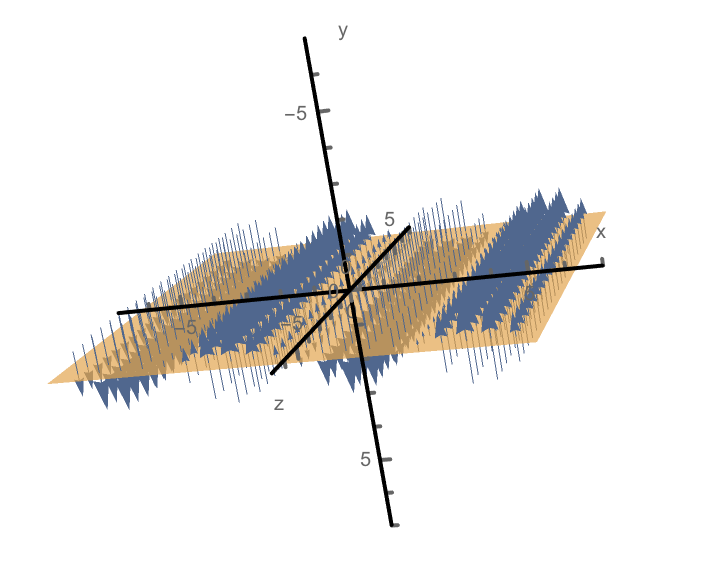
Here is a "slice" of the electric field of an electromagnetic plane wave defined over the plane $z=0$, rather than just along the $\hat x$ axis:
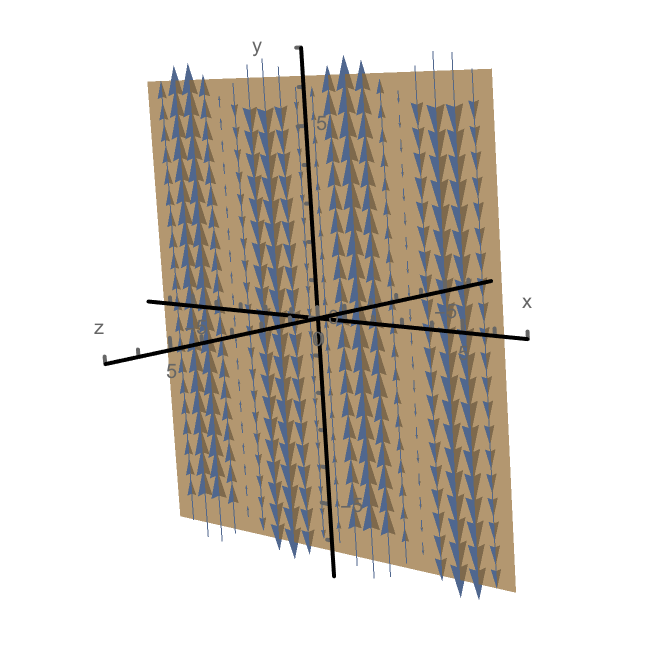
Now imagine identical copies of this plane stacked along the $\hat z$ axis, and you have a picture of what the electric field from a plane wave looks like. A faithful visual depiction would be far too cluttered to be useful, but this is the general idea.
For completeness, here's a slice of the field in the plane $y=0$:
The wave in your figure is linearly polarized with E along the $\hat{y}$ axis and B along the $\hat{z}$ axis. That's built into the wave when it was created, and it stays that way. You won't find any E along $\hat{z}$ or B along $\hat{y}$ for that wave.
Some other wave might be different. If could be linearly polarized along some other axis, or it could be circularly polarized. A circularly polarized wave has E pointing in different directions at different times.
So no, in the wave you show, it doesn't surround the $\hat{x}$ axis. But other waves could do that.
Yes, the fields are everywhere surrounding the direction of propagation. But it would be linearly polarized, in the same direction as the vectors in the figure. If one only draws the E-field vectors, it could be a representation like this: https://commons.wikimedia.org/wiki/File:Linear_Polarization_Linearly_Polarized_Light_plane_wave.svg
The EM wave in this diagram is just a ray, a geometrical visualization of the EM wave.
The EM wave is in reality a 4D wave, or at least you have to visualize it in 3D, where the wave rotates, that is, it goes from E wave to a M wave, because in the Maxwell equations, a moving E wave creates a moving M wave and this is how light propagates in the classical framework.
According to QM, the photon is the excitation of the EM field. So the E field creates an excitation in the M field, that creates an excitation in the E field, and that is how EM waves propagate in 4D.
