This says that the tension acts downwards. But then what about the weight of the bodies?
0
Add a comment
|
3 Answers
$\begingroup$
$\endgroup$
Tension in the string is the work required to pull a unit length of the string. So, when the tension is one pound, the reeling in of a one inch length of string requires one inch-pound of work.
But, the tension holding the pulley is the work to pull the pulley up one unit length. That length-of-displacement is applied to TWO strings when the pulley is moved, so if we disregard the weight of the pulley, the tension is twice that of a single string.
$\begingroup$
$\endgroup$
Basically, there is an error in understanding what basically a system is.
System is that portion of the universe you choose to study the effect of external forces on it. What to include in your system? Simply, the external forces. In case you have a difficulty in identifying the internal and external forces, I have a 'golden rule' for you. Simply remember: If action and reaction pairs both are present in a system then it's internal (this will be further explained).
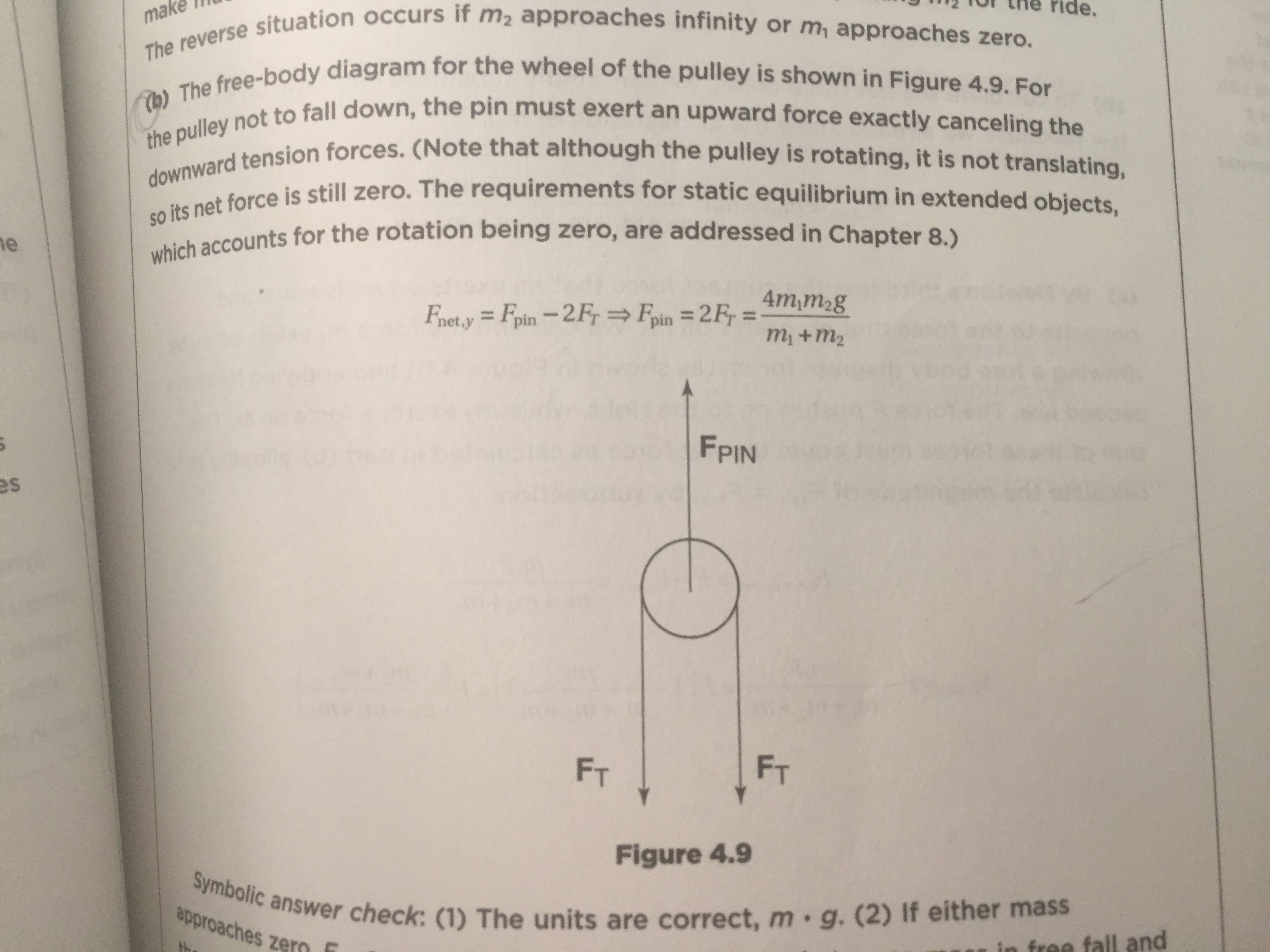
Coming back to your question, why the weight of the blocks has not been considered? Just because we have chosen to do so. As in your figure the system consists only of the pulley. What are the extrenal forces that act on it? It's only $F_T$ (tension applied by the rope) and $F_{pin}$ (exerted by the clamp). The weight of blocks will make the string taut which will eventually give rise to $F_T$ and will have no other role to play. Infact, no other forces will have any influence on this system. So by Newton's Second Law we have $$\sum F_{ext}=F_{pin}-2F_{T} $$ $$\Rightarrow F_{pin}=2F_{T}$$ (Since the system has a net acceleration zero)
You can also consider a system in which you include the whole block pulley setup. If you consider the external forces acting on the system it's the $F_{pin} by the clamp and the gravitational pull of the earth on the two blocks. Why tension has not been considered here? I want you to think of that from the point of view of that 'golden rule'. Got your answer? Exactly, because both the action and reaction pairs are in the system.
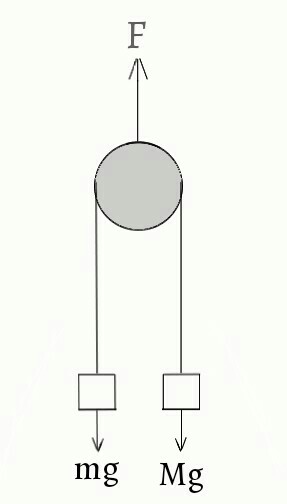
But overall it is a bit difficult to analyse this system as components of it have different acceleration. Still Newton's Law holds good. You just have to consider the acceleration of the Centre of Mass of the whole system: $$\sum F_{net}=M_{total}A_{COM} $$ Though both them will fetch you the same result, the latter one will be more time consuming.
So you tell me which one would you like to consider as your system? Of course, the first one.
$\begingroup$
$\endgroup$
The weight of the masses act on the rope and not the body. These are all contact forces, so we have to see what objects are in contact with the body (pulley) the rope is in contact with it and the pin too. Therefore the rope exerts a force T1 and T2 downwards to the pulley
