Can a massive object have an escape velocity close to $c$ and not become a black hole?

Question

It is well-documented that a given body has a well-defined Schwarzschild radius, defining a minimum radius for its volume, given its mass, before it becomes a black hole. It is clearly true, also, that the escape velocity for black holes is greater than $c$ - however, would a mass with an escape velocity 0.999$c$ also be a black hole?

My belief is that such an object would be a black hole (or on the way to being one) since such escape velocities might only be achievable in a situation where a mass is on its way to becoming a black hole (for example, it might be the escape velocity of a collapsing star). My question then, is: what is the lower-bound for the escape velocity of an object before we can know that it is or will be a black hole?

Answer 1

Unlike with the other answer, my presumption is that you're asking about the largest possible escape velocity from a stable object that's not a black hole. I.e., the circumstances you're considering don't include a situation in which the "object" involved includes some matter that's in the process of being rapidly expelled. The best candidate for such an object that's moderately well understood is likely a neutron star.

The relationship between density and mass of a neutron star isn't very precisely understood; there are a number of somewhat differing models for a neutron star's equations of state. There may well even exist quark matter that's even denser than the neutron degenerate matter that's thought to form the bulk of a neutron star, but that's venturing off even further into the limits of what's currently known.

However, according to some equations of state, there can exist "ultracompact" neutron stars which are so dense that they have a photon sphere. A photon sphere occurs at the radius at which a photon can orbit the object in question in a circle. In Schwarzschild coordinates, the photon sphere occurs at 1.5 times the Schwarzschild radius. I.e., the photon sphere occurs at a radius given by

$$r_{ps}=\frac{3GM}{c^2}\ \ .$$

Using $r_{ps}$ as the radius in the equation for escape velocity,

$$v_e=\sqrt{\frac{2GM}{r}}\ \ ,$$

which is valid even relativistically, gives that the escape velocity from a photon sphere is

$$v_e=\sqrt{\frac{2}{3}}c \approx 0.816c\ \ .$$

So it appears that there can exist stable objects (ultracompact neutron stars) which are not black holes, but which require an escape velocity of more than 0.8c to escape from the surface.

Answer 2

As a preliminary, you're using a Newtonian explanation of what is a black hole, which doesn't really work. A black hole is not defined as an object from which the escape velocity is $c$. If the Newtonian idea of escape velocity were the only thing at work, then we could hoist matter out of a black hole using a bucket on a rope.

Neither Newtonian mechanics nor general relativity prevents us from having materials with exotic properties that would allow such a collapse to halt at any stage. However, in GR we have things called energy conditions, which state some bounds on our typical expectations for the behavior of most ordinary forms of matter. Given an appropriate energy condition, there is a theorem called the Penrose singularity theorem that states that under a certain condition (the formation of a trapped surface), a singularity is guaranteed to exist somewhere in the spacetime. This singularity does not necessarily have to be a black hole singularity, and it does not necessarily have to be surrounded by an event horizon.

So your Newtonian intuition was not completely off. There is something like the thing you imagined, but the details play out much differently in GR than in Newtonian gravity.

Answer 3

You are correct, that there is a mass/radius ratio that makes it inevitable that an object will collapse to form a black hole and that this mass/radius ratio has a corresponding "escape velocity" (NB. it is a speed in Newtonian physics, but in GR it is a velocity because I think the direction matters) that is less than $c$. If an object of a given mass shrinks below this critical radius, which is larger than the Schwarzschild radius, then it will collapse to form a black hole.

The structure of a General Relativistic object is controlled by the Tolman-Oppenheimer-Volkhoff equation of hydrostatic equilibrium. This has the pressure gradient on the LHS but also features the pressure on the right hand side, because pressure is a source of space-time curvature in GR. As the object gets smaller and approaches the Schwarzschild radius, the central pressure must increase to provide the necessary pressure gradient to support the increasing weight. However, this pressure also contributes to the requirement for an increased pressure gradient and the whole thing becomes self-defeating and the object will collapse.

The details depend on the specifics of the equation of state for material at ultra-high densities, thought to exist in neutron stars, which is highly uncertain. However, there is a limit. In "Black Holes, White Dwarfs and Neutron Stars" by Shapiro & Teukolsky, (pp.260-263), it is shown, approximately, that even if the equation of state hardens to the point where the speed of sound equals the speed of light, that instability sets in if $(GM/Rc^2)<0.405$. [NB. This is for non-rotating objects, which might change things slightly, but even if causality were abandoned and you allow $P \rightarrow \infty$ then $(GM/Rc^2)<0.444$ (this the so-called Buchdahl limit).]

The Schwarzschild radius is $R_s=2GM/c^2$ and therefore $R > 1.23 R_s$ for stability. This limit is reached for a neutron star with $M \simeq 3.5 M_{\odot}$ using this equation of state. The "radial escape velocty" (according to a "shell observer" stationary at that radius) for such an object is $$ v = \left( \frac{2GM}{1.23R_s} \right)^{1/2} = \frac{c}{\sqrt{1.23}}$$

A more accurate treatment in Lattimer (2013) suggests that a maximally compact neutron star has $R\geq 1.41R_s$, which leads to an escape speed of $c/\sqrt{1.41}$.

In practice the maximum escape speed will be smaller than this because the real equation of state is unlikely to be as extreme as supposed above.

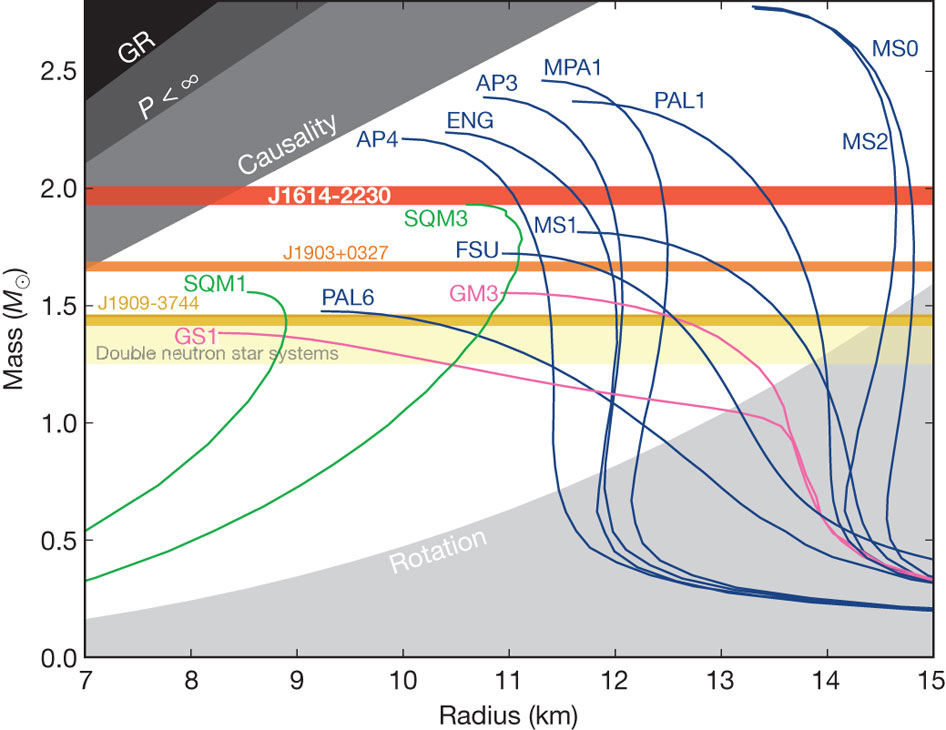
The picture below (from Demorest et al. 2010) shows the mass-radius relations for a wide variety of equations of state. The limits in the top-left of the diagram indicate the limits imposed by (most stringently) the speed of sound being the speed of light (labelled "causality" and which gives radii slightly larger than Shapiro & Teukolsky's approximate result) and then in the very top left, the border marked by "GR" coincides with the Schwarzschild radius. Real neutron stars become unstable where their mass-radius curves peak, so their radii are always significantly greater than $R_s$ at all masses and the escape speed will be given by $c$ divided by the square root of their smallest possible radius as a multiple of the Schwarzschild radius.

EDIT: Just to address the point on rotation. I found a paper that adopt the "causal" equation of state and allows neutron stars to rotate as fast as they possibly can (Friedman & Ipser 1987; see also more modern work by Cipolleta et al. 2015). These configurations do allow more massive neutron stars to exist (by 30% or more), but they also have larger radii. The net outcome is almost identical - the minimum stable radius is about $1.3R_s$. What I am unsure about is what the relationship between escape velocity and radius is in the Kerr metric. (Or even how that would be defined).

Answer 4

Whether the black hole could form at all among other thing is determined by the distribution of angular momentum in the system. So if we allow rotating bodies then the answer is: escape velocity of a stationary body could be arbitrarily close to $c$ without forming a black hole.

The only other answer that mentions rotations is Rob Jeffries' who seems to believe that rotation could only change situation only slightly. This may be true for astrophysical bodies such as neutron stars which shed excessive angular momentum during collapse. However, why limit ourselves with only natural bodies? If we can construct stationary rotating structures, then we in principle could produce extended bodies with rather extreme metrics, for example metrics arbitrarily close to extreme Kerr metric, yet not being a black hole. And so an escape velocity for such an object would be arbitrarily close to $c$ (at least in some parts of this object). The matter for the structure could be quite ordinary (no need for degenerate neutron matter) or dust-like (which simply means that internal tensions or pressures everywhere would be arbitrarily small compared to relativistic energy density $\rho c^2$).

An example of such construction could be seen here:

Neugebauer, G., & Meinel, R. (1993). The Einsteinian gravitational field of the rigidly rotating disk of dust. The Astrophysical Journal, 414, L97-L99, full text at adsabs.

There the authors construct a family of solutions for thin rigidly rotating disk (with varying density) that has an extreme Kerr metric as a limiting case. And of course, such configuration is not the only one possible. Quick googling produced another one:

Ansorg, M., Kleinwächter, A., & Meinel, R. (2002). Relativistic Dyson rings and their black hole limit. The Astrophysical Journal Letters, 582(2), L87, arXiv.

Answer 5

As long as the escape velocity is less than $c$, then the matter composing the body in question could be moving radially outward at a larger velocity which would prevent it from collapsing into a black hole. Therefore there is no threshold above the Shwarzschild radius at which something must become a black hole in the future.

Note this answer is theoretical and does not deal with what may or may not be considered "realistic" velocities or situations.