Does the escape velocity of a black hole exceed $c$ *before* a singularity is created?

Question

As an offshoot of the question Can we have a black hole without a singularity? I'm curious if the point of no return at which the massive object is condemned to become a singularity happens before its escape velocity is greater than $c$?

I envision the black hole creation process as a positive feedback loop of collapse and the escape velocity quickly shoots past $c$ on the way to becoming a singularity, but not necessarily at the very instant it becomes a singularity. This would mean that yes, a black hole (or perhaps better termed a "dark star") can exist for a brief moment in time (on the order of plank time?) without a singularity but positive feedback ensures a singularity will be created. Any truth to this statement as we know it?

Answer 1

We can represent the collapse of an object (e.g. a star) by a Penrose causal diagram looking like:

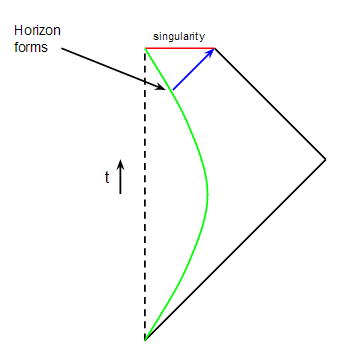
The trouble is that I now have to explain what a Penrose diagram is, but that would be a long article in itself. The vertical axis is a timelike coordinate and horizontal distance away from the vertical dashed line is sort of radial distance. One of the key points about these diagrams is that light rays always travel at 45º. You'll see why this matters in a moment.

The green line shows the surface of the collapsing object, and the red line shows the singularity. You might be a bit puzzled that the singularity is a line not as point, but remember that this is a spacetime diagram so every point in space traces out a line as it moves through time.

If we consider a light ray emitted from the surface of the star, then that light ray will travel at 45º from lower left to upper right, like the blue arrow I've drawn. It should be obvious that every light ray emitted after the blue arrow must hit the singularity, so the blue arrow shows the time at which the event horizon forms. It should also also be obvious that this happens before the singularity forms.

So the answer to your question is that yes the horizon does form before the singularity forms.

But ...

The vertical axis is not time as you and I understand it. It's actually the timelike coordinate $v$ from the Kruskal-Szekeres coordinates that has been further transformed to map the infinite time axis onto a finite line. The mapping preserves causality, so something at later "time" cannot cause something at an earlier "time". Thus we can confidently state that the singularity forms "after" the horizon. But this does not correspond in any useful way to times you might measure with your clock. In fact according your clock neither the horizon nor the singularity will ever form i.e. both would require an infinite time to form.

Answer 2

To add to John's very good answer, I'll emphasise a point that makes identifying the time when a black hole forms slightly strange: it depends on knowing the entire future evolution.

The black hole interior consists of the points from which you can't escape to infinity. But to be sure that you can escape requires knowing that nothing dramatic in the future will stop you.

For example, we could be sitting in a black hole right now: some aliens may be conspiring to collapse a huge shell of matter onto us, so great that even light we are sending out now will be pulled back in when the matter arrives. But there is no singularity, or even strong enough curvature to make Newtonian gravity invalid!

This is one manifestation of the idea that (at least in classical gravity) there is nothing special about what goes on at the event horizon.