I'm a first year student in university, when electric field of objects is taught, we have seen that an infinite large and homogeneous plane with almost zero thickness creates a constant electric field $\dfrac{\sigma}{2\epsilon_0}$ where $\sigma=Q/Area$.
1.) I can understand the following idea.
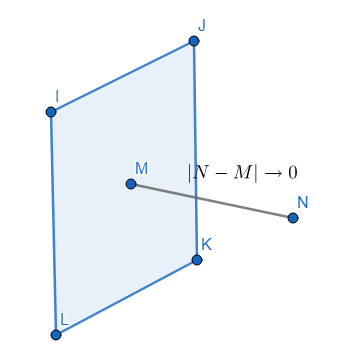
Let there be only this infinite plane(+ charged) and only one point charge $+q$:
The force on $+q$ will be $\vec F_q=kq\dfrac{\sigma\vec r}{2\epsilon_0 |\vec r| }$. Here, $\vec r$ is the radius vector from the plane to $+q$, i.e. it is independent of $r$. If the $+q$ goes so close to the plane, force must go to the infinity but also the aproached area of Q goes to the zero so $\lim\limits_{r\to 0}=k\dfrac{(Area\times \sigma)(+q)}{r^2}=\lim\limits_{r\to 0}=k\dfrac{(r^2\sigma)(+q)}{r^2}$, so again we have found a constant, so far so good.
2.) I cannot understand the following idea.
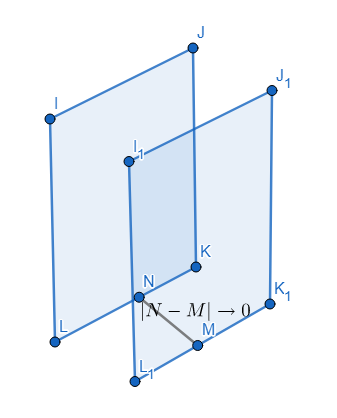
What would happen, if two infinite homogeneous (both are + charged) zero thickness planes were approached to each other?
Because of the superposition principle, we can sum their electric field in a certain area, for example when we approach two this kind plane let choose two equal area from each plane which are parallel to one another. Therefore, eventually if two planes get too close, one another force will be something like that: $F_{A_1,A_2}(r\to 0)=\lim\limits_{r\to 0}k\dfrac{(A_1\sigma)(A_2\sigma)}{r^2}=\lim\limits_{r\to 0}\dfrac{Constant}{r^2}\to\infty$
So why does not two infinity planes' electric field create an infinite force if they are too close each other.